In a radioactive decay, a nucleus is transformed into another with the emission of a positron. In this process the neutron-proton ratio
Decreases
Increases
Remains same
May decreases or increases
The correct Answer is:B
To solve the question regarding the change in the neutron-proton ratio during the emission of a positron in radioactive decay, we can follow these steps:
1. Understanding Positron Emission:
- In positron emission, a proton in the nucleus is transformed into a neutron. This process can be represented as:
p→n+e+
- Here, p is a proton, n is a neutron, and e+ is the emitted positron.
2. Change in Proton and Neutron Count:
- Before the decay, let’s denote the number of protons as Z and the number of neutrons as N.
- After the positron emission, the new number of protons becomes Z−1 (since one proton is converted into a neutron), and the number of neutrons becomes N+1 (since one neutron is created).
3. Calculating the Neutron-Proton Ratio:
- The neutron-proton ratio before the decay is:
Initial Ratio=NZ
- After the decay, the new neutron-proton ratio becomes:
New Ratio=N+1Z−1
4. Analyzing the Change in Ratio:
- To determine whether the neutron-proton ratio increases or decreases, we need to compare the initial and new ratios:
New Ratio=N+1Z−1
- Since N increases by 1 and Z decreases by 1, the numerator increases while the denominator decreases.
5. Conclusion:
- As a result, the overall neutron-proton ratio increases. Therefore, the answer to the question is that the neutron-proton ratio increases during the emission of a positron.
Final Answer:
The neutron-proton ratio increases during the emission of a positron.
---
- In positron emission, a proton in the nucleus is transformed into a neutron. This process can be represented as:
p→n+e+
- Here, p is a proton, n is a neutron, and e+ is the emitted positron.
- Before the decay, let’s denote the number of protons as Z and the number of neutrons as N.
- After the positron emission, the new number of protons becomes Z−1 (since one proton is converted into a neutron), and the number of neutrons becomes N+1 (since one neutron is created).
- The neutron-proton ratio before the decay is:
Initial Ratio=NZ
- After the decay, the new neutron-proton ratio becomes:
New Ratio=N+1Z−1
- To determine whether the neutron-proton ratio increases or decreases, we need to compare the initial and new ratios:
New Ratio=N+1Z−1
- Since N increases by 1 and Z decreases by 1, the numerator increases while the denominator decreases.
- As a result, the overall neutron-proton ratio increases. Therefore, the answer to the question is that the neutron-proton ratio increases during the emission of a positron.
The neutron-proton ratio increases during the emission of a positron.
Topper's Solved these Questions
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - NUCLEIEXERCISE - ASSIGNMENT (SECTION-D)10videos
NUCLEI
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - NUCLEIEXERCISE - ASSIGNMENT (SECTION-B)27videos
NUCLEI
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Assignment Section J (Aakash Challengers Questions)5videos
MOVING CHARGES AND MAGNETISM
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - OSCILLATIONSEXERCISE - Assignment (Section D) (ASSERTION-REASON TYPE QUESTIONS)13videos
OSCILLATIONS
Similar Questions
Knowledge Check
- Question 1 - Select One
A radioactive decay can form an isotope of the original nucleus with the emission of particles
Aone α and four βBone α and two βCone α and one βDfour α and one β
When a β− particle is emitted from a nucleus, the neutrons-proton ratio:
View SolutionThomson proposed that the nucleus of an atom contains protons and neutrons.
View SolutionIn radioactive decay process, the negatively changed emitted β−particle are
View SolutionDuring alpha-decay , a nucleus decays by emitting an α-particle ( a helium nucleus .2He4) according to the equation
.AZX→.A−4Z−2Y+.42He+Q
In this process, the energy released Q is shared by the emitted α-particle and daughter nucleus in the form of kinetic energy .
The energy Q is divided in a definite ratio among the α-particle and the daughter nucleus .
A nucleus that decays spontaneously by emitting an electron or a positron is said to undergo β-decay .This process also involves a release of definite energy . Initially, the β-decay was represented as .AZX→.AZ+1Y+e−(electron)+Q
According to this reaction, the energy released during each decay must be divided in definite ratio by the emitted e' (β-particle) and the daughter nucleus. While , in alpha decay, it has been found that every emitted α-particle has the same sharply defined kinetic energy. It is not so in case of β-decay . The energy of emitted electrons or positrons is found to vary between zero to a certain maximum value. Wolfgang Pauli first suggested the existence of neutrinoes in 1930. He suggested that during β-decay, a third particle is also emitted. It shares energy with the emitted β particles and thus accounts for the energy distribution.
During β+ decay (positron emission) a proton in the nucleus is converted into a neutron, positron and neutrino. The reaction is correctly represented asView SolutionWhich of the following assertions are correct?
(i) A neutron can decay to a proton only inside a nucleus
(ii) A proton can change to a neutron only inside a nucleus
(iii) An isolated neutron can change into a proton
(iv) An isolated proton can change into a neutronView SolutionMatch the column in radioactive decay process of nucleus with atomic number Z and mass number A.
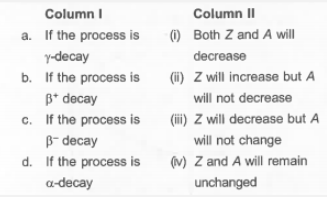 View Solution
View SolutionDuring alpha-decay , a nucleus decays by emitting an α-particle ( a helium nucleus .2He4) according to the equation
.AZX→.A−4Z−2Y+.42He+Q
In this process, the energy released Q is shared by the emitted α-particle and daughter nucleus in the form of kinetic energy .
The energy Q is divided in a definite ratio among the α-particle and the daughter nucleus .
A nucleus that decays spontaneously by emitting an electron or a positron is said to undergo β-decay .This process also involves a release of definite energy . Initially, the β-decay was represented as .AZX→.AZ+1Y+e−(electron)+Q
According to this reaction, the energy released during each decay must be divided in definite ratio by the emitted e' (β-particle) and the daughter nucleus. While , in alpha decay, it has been found that every emitted α-particle has the same sharply defined kinetic energy. It is not so in case of β-decay . The energy of emitted electrons or positrons is found to vary between zero to a certain maximum value. Wolfgang Pauli first suggested the existence of neutrinoes in 1930. He suggested that during β-decay, a third particle is also emitted. It shares energy with the emitted β particles and thus accounts for the energy distribution.
The beta particles (positron) are emitted with different kinetic energies becauseView Solution

