Find ∮→B.→dl over following loops (direction in which integration has to be performed is indicated by arrows)

The correct Answer is:(i)(mu_(0)i)/(2R)[(pi+1)/(pi)]hatk1
(ii) μ0i8r into the page
(iii) 3μ0l8r into the page
(iV)μ0l2πR+μ0l4R outside the page
(v) μ0l4r−μ0l4πr inward
Step by step video & image solution for Find oint vec(B).vec(dl) over following loops (direction in which integration has to be performed is indicated by arrows) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MOVING CHARGE AND MAGNESIUMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGE AND MAGNESIUMEXERCISE - EXERCISE
30 Videos
- MOVING CHARGE AND MAGNESIUMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGE AND MAGNESIUMEXERCISE - ASSIGNMENT (SECTION A)
34 Videos
- MOVING CHARGE AND MAGNESIUMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGE AND MAGNESIUMEXERCISE - ILLUSTRATION
12 Videos
- MOTION IN STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN STRAIGHT LINEEXERCISE - Assignment (SECTION - J)
2 Videos
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Assignment Section J (Aakash Challengers Questions)
5 Videos
Similar Questions
Find the values of ∮→B.→dl for the loops L1,L2andL3 in Fig. (The sense of →dl is mentioned in the figure)
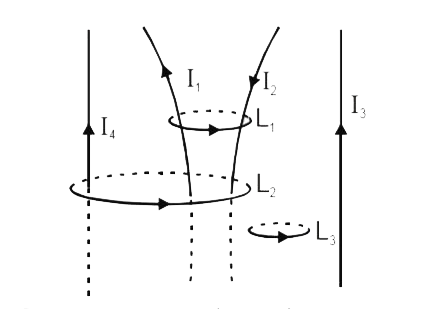
View SolutionFind the values of ∮→B.→dl for the loops L1,L2andL3 in Fig. (The sense of →dl is mentioned in the figure)

View SolutionA closed current-carrying loop having a current I is having area A. Magnetic moment of this loop is defined as →μ=−→IA where direction of area vector is towards the observer if current is flowing in anticlockwise direction with respect to the observer. If this loop is placed in a uniform magnetic field →B, then torque acting on the loop is given by →τ=→μ×→B. Now answer the following questions:
Let ring in the above question is having a radius R and a charge Q is uniformly distributed over it. Ring is rotated with a constant angular velocity (ω) as mentioned above.
Torque acting on the ring due to magnetic force is
View SolutionA cylinder wire of radius R is carrying uniformly distributed current I over its cross-section. If a circular loop of radius r is taken as amperian loop, then the variation value of ∮→B⋅→dl over this loop with radius 'r' of loop will be best represented by
View SolutionA closed current-carrying loop having a current I is having area A. Magnetic moment of this loop is defined as →μ=−→IA where direction of area vector is towards the observer if current is flowing in anticlockwise direction with respect to the observer. If this loop is placed in a uniform magnetic field →B, then torque acting on the loop is given by →τ=→μ×→B. Now answer the following questions:
A uniformly charged insulating ring is rotated in a uniform magnetic field about its own axis, then
View SolutionA closed current-carrying loop having a current I is having area A. Magnetic moment of this loop is defined as →μ=−→IA where direction of area vector is towards the observer if current is flowing in anticlockwise direction with respect to the observer. If this loop is placed in a uniform magnetic field →B, then torque acting on the loop is given by →τ=→μ×→B. Now answer the following questions:
Consider the situation shown in Fig., ring is having a uniformly distributed positive charge. Magnetic field is perpendicular to the axis of ring. Now ring is rotated in anticlockwise direction as seen from left hand side direction of magnetic torque acting on the ring is
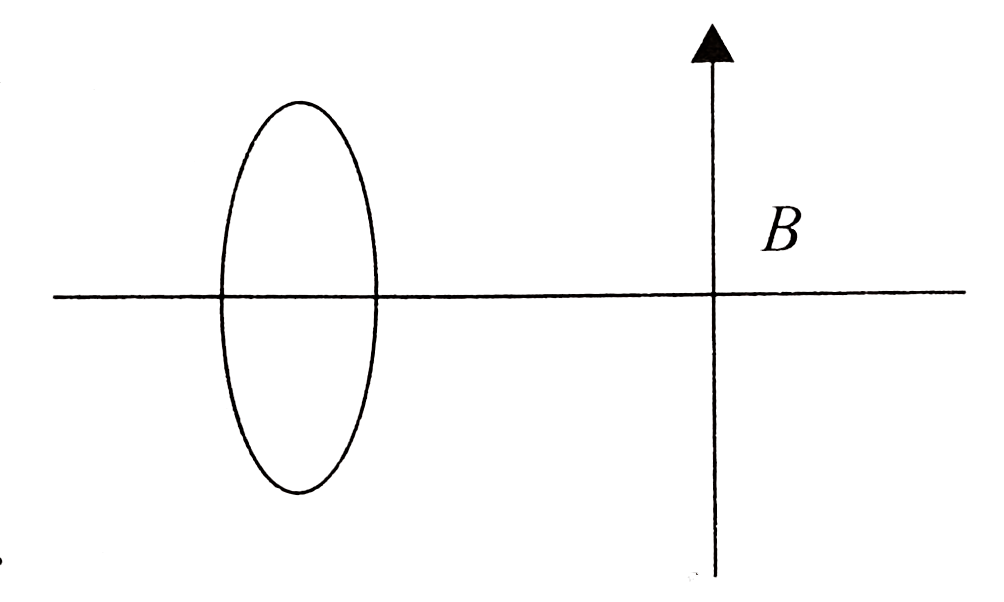
View SolutionA: In any magnetic field region the line integral ∮→B.→dl along a closed loop is always zero.
R: The magnetic field →B in the expressioin ∮→B.→dl is due to the currents enclosed only by the loop.
View SolutionFigure shows five lettered regions in which a uniform magnetic field extends directly either out of the page (as in region a) or into he page. The field is increasing in magnitude at the same steady rate in all five regions, the regions are identical in area. Also shows are four numbered paths along which ∮→E.→dl has the magnitudes given below in terms of a quantity mag. Determine whether the magnetic fields in regions b through e are directed into ot out of the page.
 ?
?
∣∣ ∣∣Path1234∮→E.→dlmag2(mag)3(mag)0∣∣ ∣∣
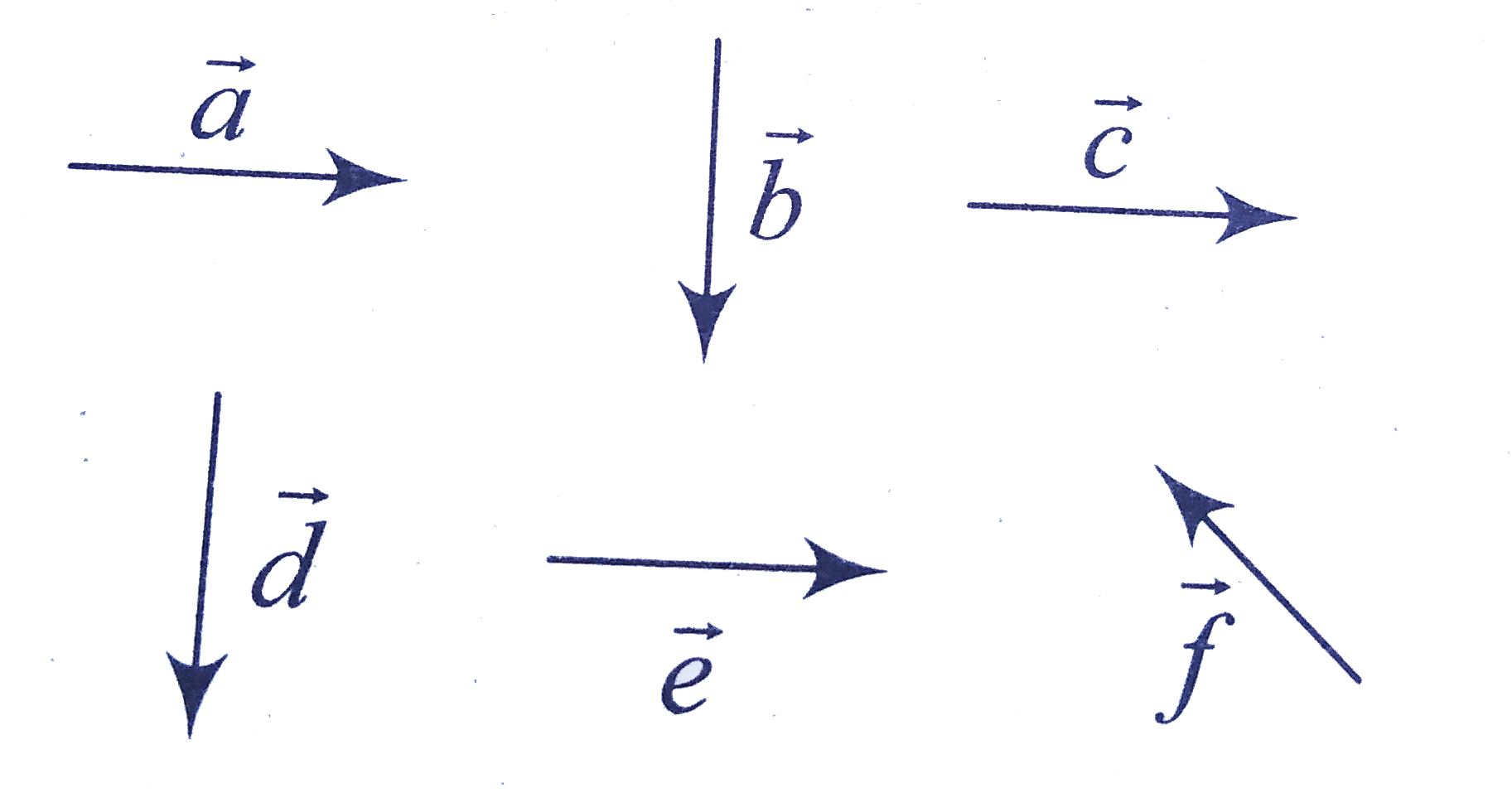
View SolutionSix vector →a through →f have the magnitudes and direction indicated in the figure. Which of the following statements is true?
View SolutionSix vector →a through →f have the magnitudes and direction indicated in the figure. Which of the following statements is true?
View Solution

