An electron having a charged e moves with a velcoity v in postive x direction. A magnetic field acts on it in positive y direction. The force on the electron acts in (where outward direction is taken as positive z-axis)
Negative direction of y-axis
Positive direction on y-axis
Positive direftion of Z-axis
Negative direction of z-axis
The correct Answer is:D
To solve the problem, we will use the concept of the Lorentz force, which describes the force acting on a charged particle moving in a magnetic field. The force is given by the equation:
F=Q(v×B)
Where:
- F is the force on the charge,
- Q is the charge of the particle,
- v is the velocity vector of the particle,
- B is the magnetic field vector.
1. Identify the Charge, Velocity, and Magnetic Field:
- The charge of the electron is Q=−e (negative because it is an electron).
- The velocity v is in the positive x-direction, which can be represented as v=v^i.
- The magnetic field B is in the positive y-direction, represented as B=B^j.
2. Apply the Lorentz Force Formula:
- Substitute the values into the Lorentz force equation:
F=Q(v×B)=−e(v×B)
- This becomes:
F=−e(v^i×B^j)
3. Calculate the Cross Product:
- We need to calculate ^i×^j:
^i×^j=^k
- Therefore, we have:
F=−e(vB^k)
4. Determine the Direction of the Force:
- Since F=−evB^k, the negative sign indicates that the force is in the negative z-direction.
- Thus, the force on the electron acts in the negative z-direction.
5. Conclusion:
- The force on the electron acts in the negative z-direction.
Final Answer:
The force on the electron acts in the negative z-direction.
- F is the force on the charge,
- Q is the charge of the particle,
- v is the velocity vector of the particle,
- B is the magnetic field vector.
- The charge of the electron is Q=−e (negative because it is an electron).
- The velocity v is in the positive x-direction, which can be represented as v=v^i.
- The magnetic field B is in the positive y-direction, represented as B=B^j.
- Substitute the values into the Lorentz force equation:
F=Q(v×B)=−e(v×B)
- This becomes:
F=−e(v^i×B^j)
- We need to calculate ^i×^j:
^i×^j=^k
- Therefore, we have:
F=−e(vB^k)
- Since F=−evB^k, the negative sign indicates that the force is in the negative z-direction.
- Thus, the force on the electron acts in the negative z-direction.
- The force on the electron acts in the negative z-direction.
The force on the electron acts in the negative z-direction.
Topper's Solved these Questions
- MOVING CHARGE AND MAGNESIUMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGE AND MAGNESIUMEXERCISE - SECTION B
17 Videos
- MOVING CHARGE AND MAGNESIUMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGE AND MAGNESIUMEXERCISE - SECTION C
47 Videos
- MOVING CHARGE AND MAGNESIUMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGE AND MAGNESIUMEXERCISE - EXERCISE
30 Videos
- MOTION IN STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN STRAIGHT LINEEXERCISE - Assignment (SECTION - J)
2 Videos
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Assignment Section J (Aakash Challengers Questions)
5 Videos
Similar Questions
An electron moving towards the east enters a magnetic field directed towards the north. The force on the electron will be directed
View SolutionA charge moving with velocity V in X direction is subjected to a field of magnetic induction in the negative X direction . As a result the charge will
View SolutionA proton is moving along the negative direction of X-axis in a magnetic field directed along the positive direction of Y-axis. The proton will be deflected along the negative direction of
View SolutionA conductor rod AB moves parallel to X-axis in a uniform magnetic field, pointing in the positive Z-direction. The end A of the rod gets-
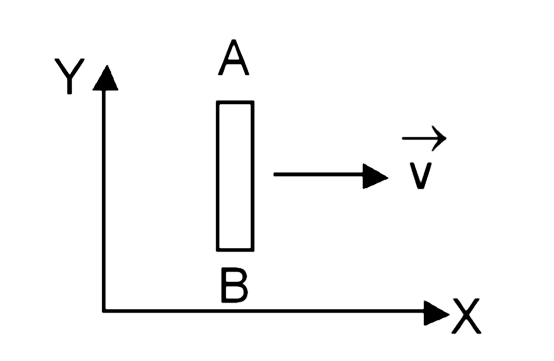
View SolutionA conducting rod AB moves parallel to the x-axis (see Fig.) in a uniform magnetic field pointing in the positive z-direction. The end A of the rod gets positively charged.
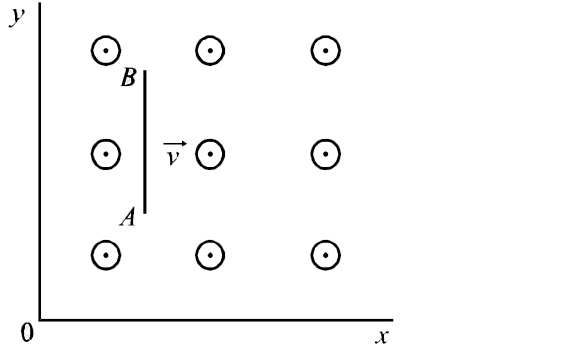 .
.
View SolutionAn electron is fired parallel to uniform electric and uniform magnetic field acting simultaneously and in the same direction. The electron
View SolutionA charge +Q is moving upwards vertically. It enters a magnetic field directed to the north. The force on the charge will be towards
View SolutionAn electron is travelling along the x-direction. It encounters a magnetic field in the y-direction. Its subsequent motion will be
View SolutionAn electron is travelling along the x-direction. It encounters a magnetic field in the y-direction. Its subsequent motion wil be
View SolutionA plane polarized electromagnetic wave of frequency 6×108Hz is propagating through a region of space. Electric field is along positive z-direction and magnetic field is along positive y-direction at some instant. The peak value of electric field is 3 milli-volt/m.
The direction of propagation of wave is
View Solution
