Two bodies having masses m1 and m2 and velocities v1 and v2 colide and form a composite system. If m1v1+m2v2=0(m1≠m2. The velocity of composite system will be
To solve the problem step by step, we will use the principle of conservation of linear momentum.
1. Understand the Problem: We have two bodies with masses m1 and m2 moving with velocities v1 and v2 respectively. After colliding, they form a composite system. We need to find the velocity of this composite system.
2. Apply Conservation of Momentum: According to the conservation of momentum, the total momentum before the collision must equal the total momentum after the collision. The equation can be written as:
m1v1+m2v2=(m1+m2)v
where v is the velocity of the composite system after the collision.
3. Given Condition: The problem states that:
m1v1+m2v2=0
This means that the total momentum before the collision is zero.
4. Substituting the Condition: Substitute the given condition into the momentum equation:
0=(m1+m2)v
5. Solving for v: Since m1 and m2 are not equal to zero (as they are masses), we can divide both sides of the equation by (m1+m2):
v=0
6. Conclusion: The velocity of the composite system after the collision is:
v=0
Final Answer:
The velocity of the composite system is 0.
---
m1v1+m2v2=(m1+m2)v
where v is the velocity of the composite system after the collision.
m1v1+m2v2=0
This means that the total momentum before the collision is zero.
0=(m1+m2)v
v=0
v=0
The velocity of the composite system is 0.
Similar Questions
Two masses, m1 and m2 , are moving with velocities v1 and v2. Find their total kinetic energy in the reference frame of centre of mass.
View SolutionTwo balls A and B of masses m and 2 m are in motion with velocities 2v and v, respectively. Compare: Their momentum.
View SolutionTwo particles of masses m1 and m2 in projectile motion have velocities →v1 and →v2 , respectively , at time t=0. They collide at time t0. Their velocities become →v'1 and →v'2 at time 2t0 while still moving in air. The value of ∣∣∣(m1→v'1+m2→v'2)−(m1→v1+m2→v2)∣∣∣
View SolutionTwo masses mA and mB moving with velocities vA and vB in opposite direction collide elastically after that the masses mA and mB move with velocity vB and vA respectively. The ratio (mA/mB) is
View SolutionTwo balls A and B of masses m and 2 m are in motion with velocities 2v and v, respectively. Compare: Their inertia.
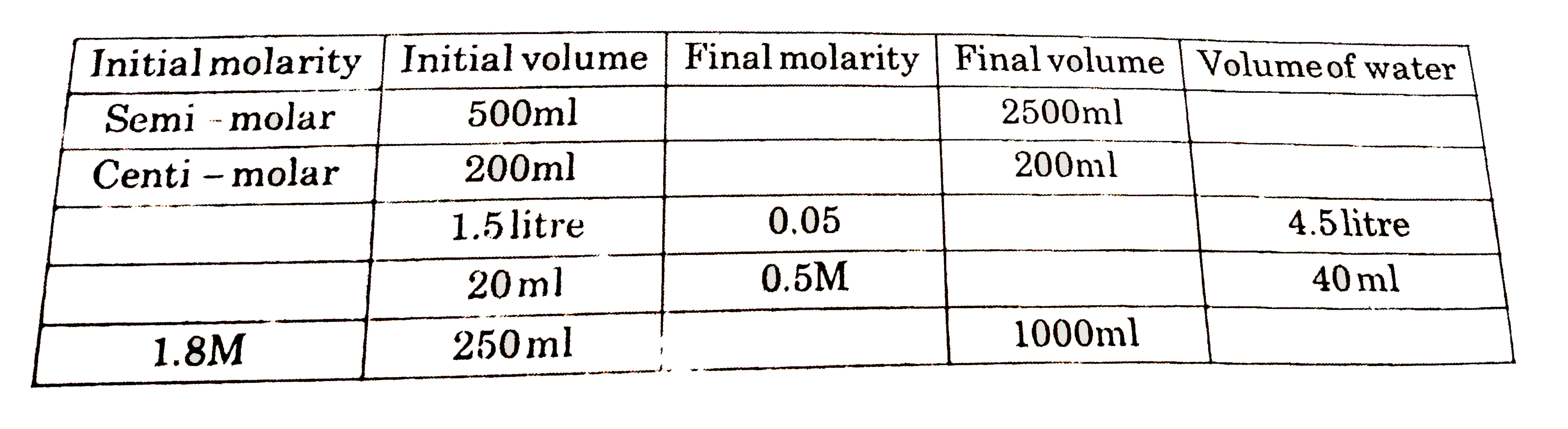
View SolutionUse of dilution formula (M1V1=M2V2)
View SolutionTwo balls with masses m1=3 and m2=5kg have initial velocities v1=v2=5m/s in the directions shown in figure. They collide at the origin.
a. find the velocioty of the CM 3s before the collision.
b. Find the position of the CM 2s after the collision.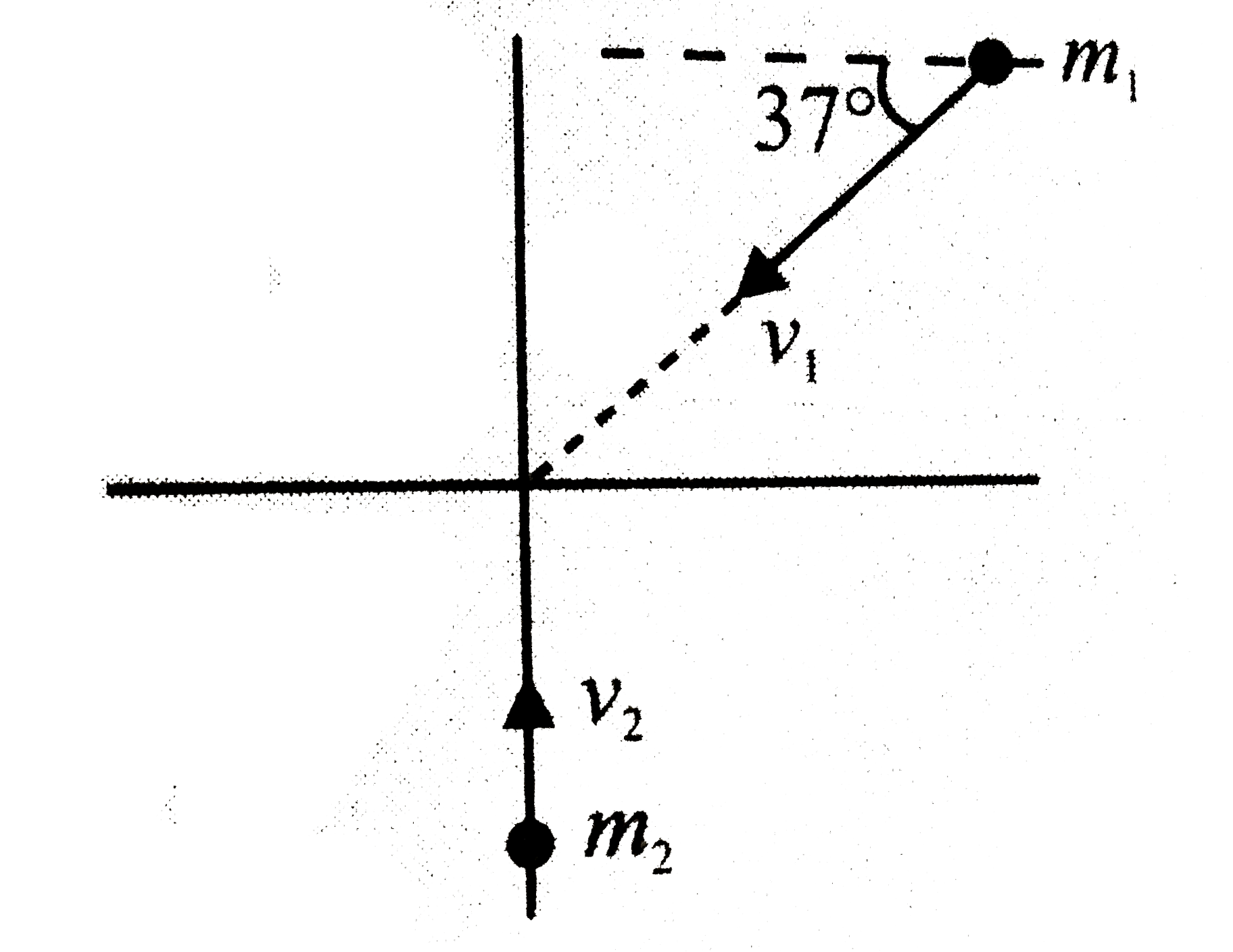
View SolutionTwo bodies of masses (m1) and (m2) are droppded from heithts h1 and h2, respectively. They reach the ground after time t1 and t2 and strike the ground with v1 and v2, respectively Choose the correct relations from the following.
View SolutionTwo balls of masses m1,m2 and speeds v1 and v2 collide at right angle . The maximum amount of kinetic energy loss due ot inelastic collision is ___.

View SolutionTwo particles of mass mA and mB and their velocities are VA and VB respectively collides. After collision they interchanges their velocities, then ratio of mAmB is
View Solution
