Which statement is correct?
dh(dT)P<dE(dT)V
dH(dT)P+dE(dT)V=R
dE(dV)T for ideal gas is zero
All of these
The correct Answer is:C
To solve the question "Which statement is correct?" regarding the relationships between changes in enthalpy (ΔH) and internal energy (ΔE), we will analyze each statement step by step.
1. Understanding the Definitions:
- ΔH (change in enthalpy) and ΔE (change in internal energy) are thermodynamic properties of a system.
- The relationship between these two properties can be expressed in terms of temperature (T), pressure (P), and volume (V).
2. Using the Thermodynamic Relationships:
- At constant pressure, the change in enthalpy with respect to temperature is given by:
dHdT=Cp
- At constant volume, the change in internal energy with respect to temperature is given by:
dEdT=Cv
3. Applying the Relationship Between Cp and Cv:
- The relationship between heat capacities is given by:
Cp−Cv=R
- This implies that Cp>Cv.
4. Analyzing the First Statement:
- The first statement claims that:
dHdT<dEdT
- Since we know Cp>Cv, this statement is incorrect.
5. Analyzing the Second Statement:
- The second statement claims:
dHdT+dEdT=R
- However, we have:
dHdT−dEdT=R
- Therefore, this statement is also incorrect.
6. Analyzing the Third Statement:
- The third statement discusses the derivatives of internal energy and enthalpy concerning volume for an ideal gas:
dUdV=0(at constant temperature)
- This is true because the internal energy of an ideal gas is independent of volume at constant temperature. Thus, this statement is correct.
7. Analyzing the Fourth Statement:
- The fourth statement claims that "all of the above" statements are correct. Since we have found the first two statements to be incorrect, this statement is also incorrect.
Conclusion:
The correct statement is the third one, which states that the change in internal energy with respect to volume for an ideal gas is zero at constant temperature.
Final Answer:
The correct option is C.
---
- ΔH (change in enthalpy) and ΔE (change in internal energy) are thermodynamic properties of a system.
- The relationship between these two properties can be expressed in terms of temperature (T), pressure (P), and volume (V).
- At constant pressure, the change in enthalpy with respect to temperature is given by:
dHdT=Cp
- At constant volume, the change in internal energy with respect to temperature is given by:
dEdT=Cv
- The relationship between heat capacities is given by:
Cp−Cv=R
- This implies that Cp>Cv.
- The first statement claims that:
dHdT<dEdT
- Since we know Cp>Cv, this statement is incorrect.
- The second statement claims:
dHdT+dEdT=R
- However, we have:
dHdT−dEdT=R
- Therefore, this statement is also incorrect.
- The third statement discusses the derivatives of internal energy and enthalpy concerning volume for an ideal gas:
dUdV=0(at constant temperature)
- This is true because the internal energy of an ideal gas is independent of volume at constant temperature. Thus, this statement is correct.
- The fourth statement claims that "all of the above" statements are correct. Since we have found the first two statements to be incorrect, this statement is also incorrect.
The correct statement is the third one, which states that the change in internal energy with respect to volume for an ideal gas is zero at constant temperature.
The correct option is C.
Topper's Solved these Questions
- THERMODYNAMICSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - ASSIGNMENT (Section - C) Previous Years Questions
60 Videos
- THERMODYNAMICSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - ASSIGNMENT (Section -D) Assertion-Reason Type Questions
15 Videos
- THERMODYNAMICSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - ASSIGNMENT (Section - A) Objective Type Questions
55 Videos
- THE SOLID STATE BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - THE SOLID STATE EXERCISE - Assignment (SECTION - D) (ASSERTION-REASON TYPE QUESTION)
20 Videos
Similar Questions
Which statements is correct about mosses?
View SolutionWhich statements is correct regarding the diagonal relationship between the Al and Be?
View SolutionWhich statement are correct about the following compounds ?
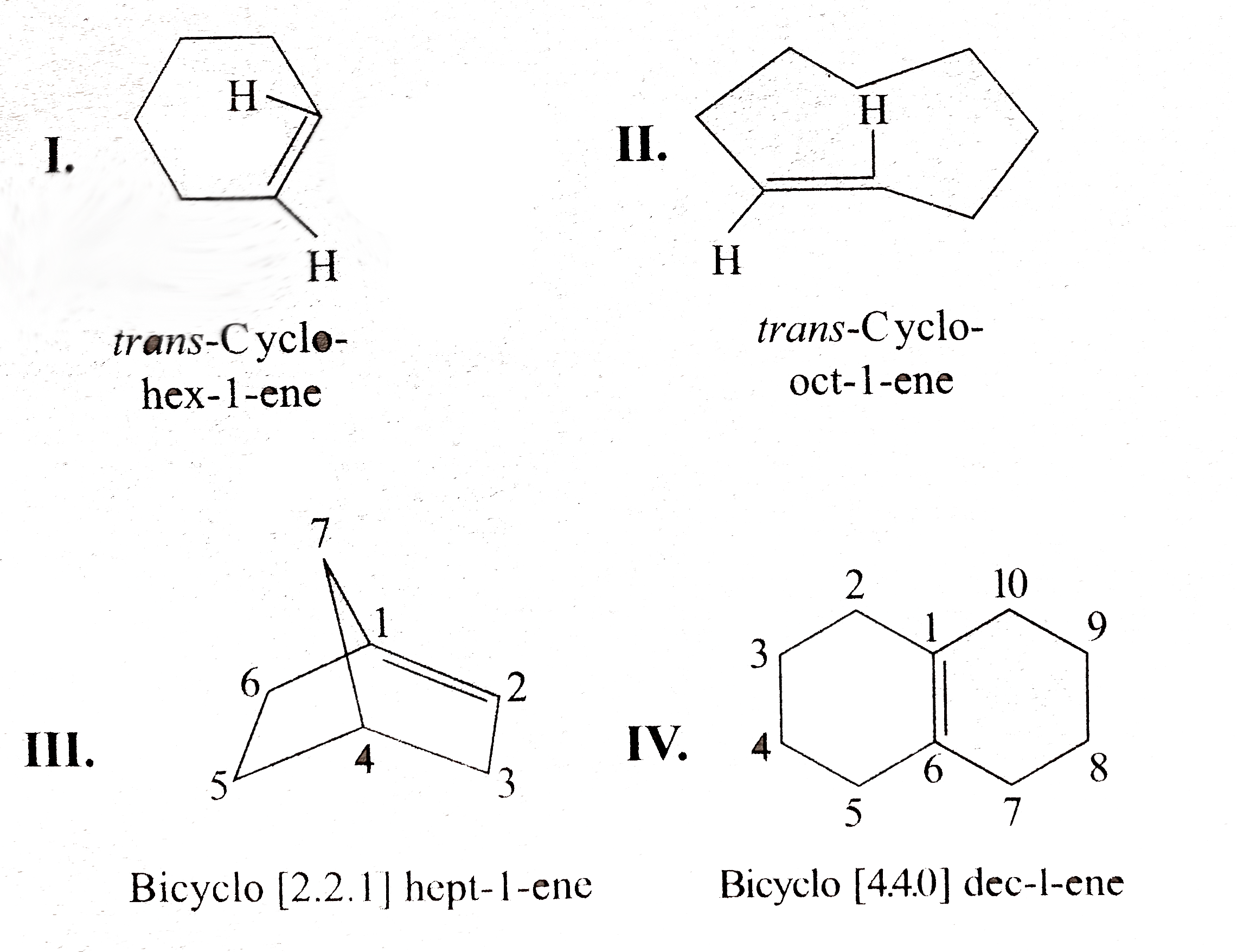
View SolutionWhich statement(s) is correct,
View SolutionWhich statements are correct ?
View SolutionWhich statements are correct:
View SolutionWhich statements are correct for ozone?
View SolutionWhich statements are correct about sucrose?
View SolutionWhich statements are correct about lactose?
View SolutionWhich statements are correct about the following disaccharide?
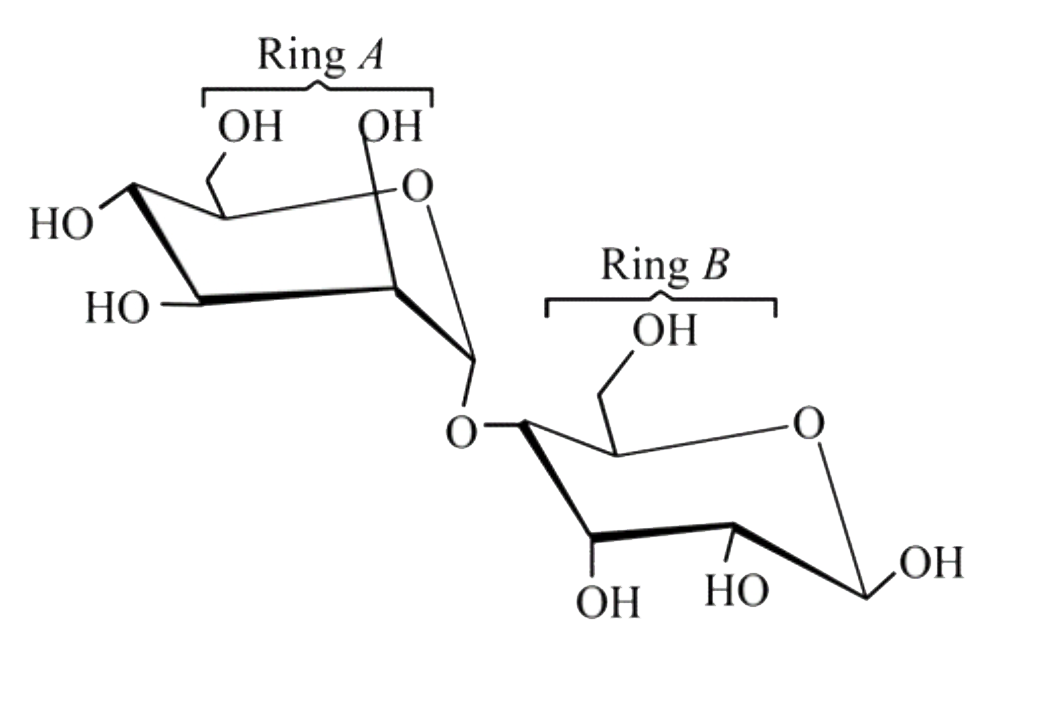
View Solution
