A block is pushed through 2m across is surface offering 25 N resistance. The work done by the resisting force
J
25J
J
J
The correct Answer is:C
To find the work done by the resisting force when a block is pushed across a surface, we can follow these steps:
1. Identify the Given Values:
- Displacement (S) = 2 m
- Resisting Force (F) = 25 N
2. Understand the Direction of Forces:
- The block is pushed in one direction (let's say to the right), while the resisting force acts in the opposite direction (to the left).
3. Use the Work Done Formula:
- The work done (W) by a force is given by the formula:
W=F⋅S⋅cos(θ)
- Where:
- F is the magnitude of the force,
- S is the displacement,
- θ is the angle between the force and the direction of displacement.
4. Determine the Angle:
- Since the resisting force acts in the opposite direction to the displacement, the angle θ is 180 degrees.
5. Calculate the Cosine of the Angle:
- cos(180∘)=−1
6. Substitute the Values into the Formula:
- Now substituting the values into the work done formula:
W=F⋅S⋅cos(180∘)=25N⋅2m⋅(−1)
7. Perform the Calculation:
- Calculate:
W=25⋅2⋅(−1)=−50J
8. Conclusion:
- The work done by the resisting force is −50J.
Final Answer:
The work done by the resisting force is -50 Joules.
---
- Displacement (S) = 2 m
- Resisting Force (F) = 25 N
- The block is pushed in one direction (let's say to the right), while the resisting force acts in the opposite direction (to the left).
- The work done (W) by a force is given by the formula:
W=F⋅S⋅cos(θ)
- Where:
- F is the magnitude of the force,
- S is the displacement,
- θ is the angle between the force and the direction of displacement.
- Since the resisting force acts in the opposite direction to the displacement, the angle θ is 180 degrees.
- cos(180∘)=−1
- Now substituting the values into the work done formula:
W=F⋅S⋅cos(180∘)=25N⋅2m⋅(−1)
- Calculate:
W=25⋅2⋅(−1)=−50J
- The work done by the resisting force is −50J.
The work done by the resisting force is -50 Joules.
Topper's Solved these Questions
Similar Questions
A box is pushed through 4.0 m across floor offering 100 N resistance.How much work is done by the resisting force?
View SolutionA body is pushed through 5 m across a surface offering 75 N resistance How much work is done by the (1) applied force (ii) resisting force ?
View SolutionA force F is applied on a block of mass M. The block is displaced through a distance d in the direction of the force. What is the work done by the force on the block? Does the internal energy change because of this work?
View SolutionA ball of mass 1 kg falls from a height of 5m above the free surface of water. The relative density of the solid ball is s=2/3. The ball travels a distance of 2m under water and becomes stationary. The work done by the resistive forces of water is
View SolutionA ball of mass 1 kg falls from a height of 5 m above the free surface of water. The relative density of the solid ball is s=23. The ball travels a distance of 2 m under water and becomes stationary. The work done by the resistive forces of water is (−10n)J. Find value of n.
View SolutionA ball of mass 5.0 gm and relative density 0.5 strikes the surface of the water with a velocity of 20 m/sec. It comes to rest at a depth of 2m. Find the work done by the resisting force in water: (take g=10m/s2)
View SolutionA block of mass 10 kg slides down on an incline 5 m long and 3 m high. A man pushes up on the ice block parallel to the incline so that it slides down at constant speed. The coefficient of friction between the ice and the incline is 0.1. Find :
(a) the work done by the man on the block.
(b) the work done by gravity on the block.
(c) the work done by the surface on the block.
(d) the work done by the resultant forces on the block.
(e) the change in K.E. of the block.
View SolutionA block Q of mass 2m is placed on a horizontal frictionless plane. A second block of mass m is placed on it and is connected to a spring of spring constant K, the two block are pulled by distance A. Block Q oscillates without slipping. The work done by the friction force on block Q when the spring regains its natural length is:
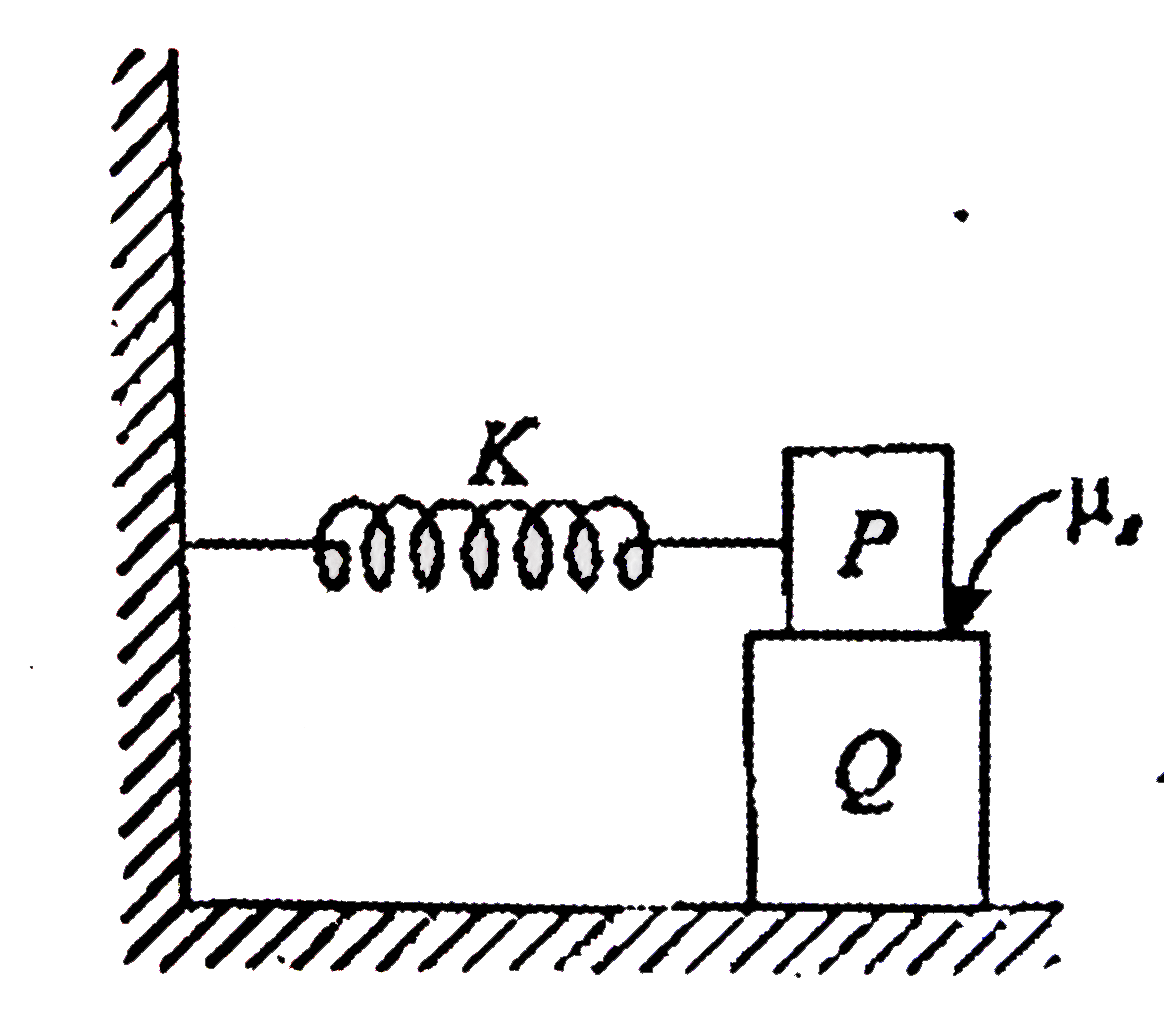
View SolutionA force of 10N is acting on a block of 20kg on horizontal surface with coefficient of friction μ=0.2. Calculate the work done by the force.
View SolutionA spring of spring constasnt 50 N/m is compressed from its natural position through 1 cm. Find the work done by the work done by the spring force on the agency compressing the spring.
View Solution
