The angular momentum of a body is given by L=5t2+2t+1kgm2s.The torque acting on the body at t=1s is
12N−m
10N-m
4N-m
Zero
The correct Answer is:A
To solve the problem, we need to find the torque acting on the body at t=1 second, given the angular momentum L as a function of time t.
1. Identify the given angular momentum function:
The angular momentum L is given by:
L=5t2+2t+1(in kg m2/s)
2. Recall the relationship between torque and angular momentum:
Torque τ is defined as the rate of change of angular momentum with respect to time:
τ=dLdt
3. Differentiate the angular momentum function:
We need to differentiate L with respect to t:
dLdt=ddt(5t2+2t+1)
Using the power rule of differentiation:
- The derivative of 5t2 is 10t.
- The derivative of 2t is 2.
- The derivative of a constant (1) is 0.
Thus, we have:
dLdt=10t+2
4. Substitute t=1 into the derivative:
Now, we will find the torque at t=1 second:
τ=10(1)+2=10+2=12(in N m)
5. Conclusion:
Therefore, the torque acting on the body at t=1 second is:
τ=12N m
Final Answer:
The torque acting on the body at t=1 second is 12N m.
The angular momentum L is given by:
L=5t2+2t+1(in kg m2/s)
Torque τ is defined as the rate of change of angular momentum with respect to time:
τ=dLdt
We need to differentiate L with respect to t:
dLdt=ddt(5t2+2t+1)
Using the power rule of differentiation:
- The derivative of 5t2 is 10t.
- The derivative of 2t is 2.
- The derivative of a constant (1) is 0.
dLdt=10t+2
Now, we will find the torque at t=1 second:
τ=10(1)+2=10+2=12(in N m)
Therefore, the torque acting on the body at t=1 second is:
τ=12N m
The torque acting on the body at t=1 second is 12N m.
Topper's Solved these Questions
Similar Questions
A particle is moving on a straight line x + y = 2. Its angular momentum about origin is L = 3t + 2 (kg m2s−1). Find the force acting on the particle at t = 2 s. (x and y are in metre)
View SolutionThe angular displacement of a body is given by θ=2t2+5t−3. Find the value of the angular velocity and angular acceleration when t = 2s.
View Solution
Knowledge Check
The position time graph of a body of mass 2kg is as given in What is the impulse on the body at t=0 s and t=4s ?
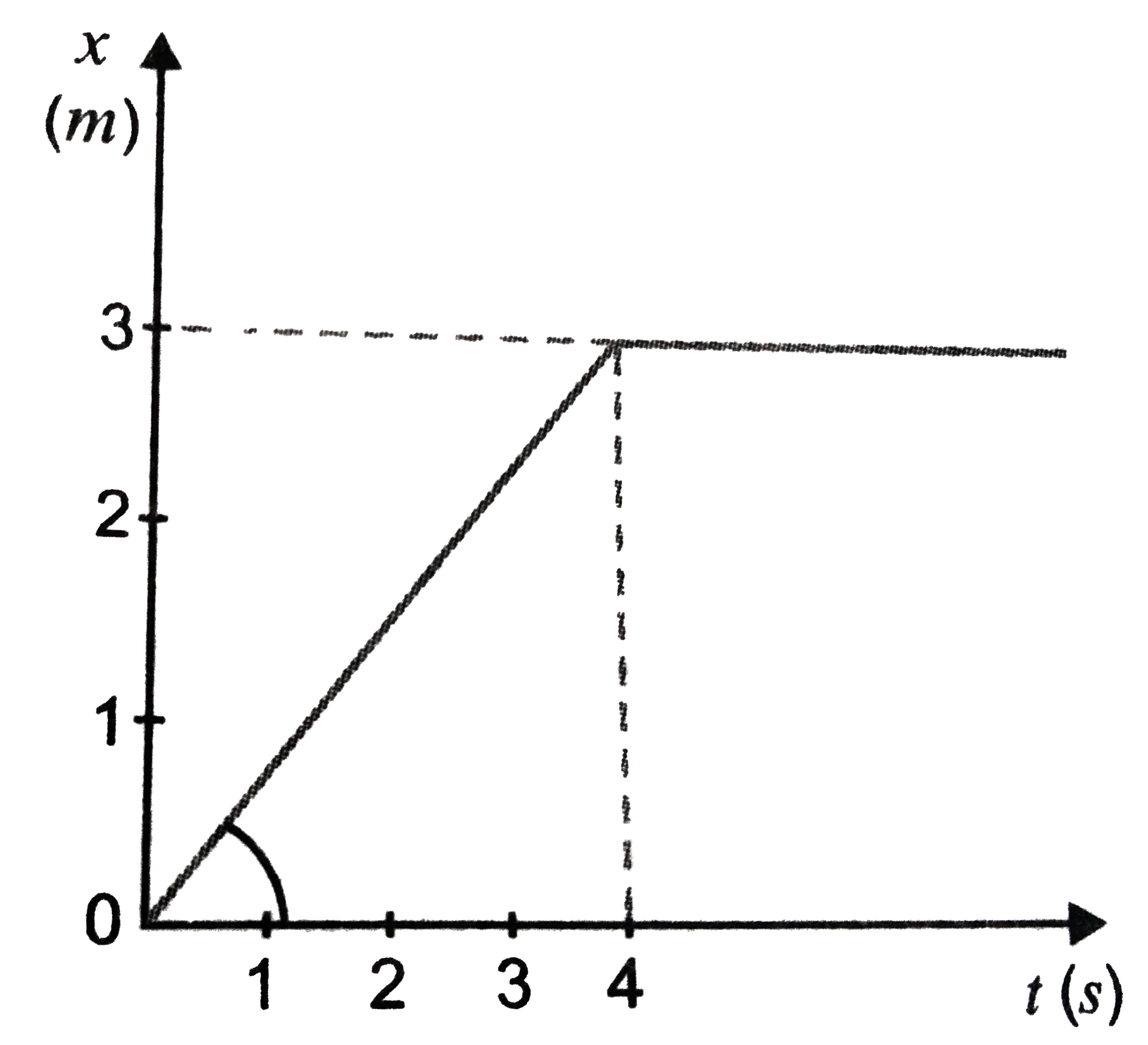 . A23kgms−1B−23kgms−1C32kgms−1D−32kgms−1
. A23kgms−1B−23kgms−1C32kgms−1D−32kgms−1
The momentum of a body in two perpendicular direction at any time 't' are given by Px=2t2+6 and Py=3t22+3. The force acting on the body at t= 2 sec is
View SolutionThe angular displacement of a particle is given by θ=t3+t2+t+1 then, its angular velocity at t = 2sec is ……….. rad s−1
View SolutionThe displacement of a body is given by s=12gt2 where g is acceleration due to gravity. The velocity of the body at any time t is
View SolutionThe position of a body moving along x-axis at time t is given by x=(t2−4t+6)m. The velocity of the body at time t = 3s is
View SolutionAt time t, the position of a body moving along the x - axis is x=t3−6t2+9tm The deceleration of the body at 1 s is
View SolutionA body of mass 2kg travels according to the law x(t) = pt+qt2+rt3 where , q = 4ms−1 , p = 3ms−1 and r=5ms−1. The force acting on the body at t = 2s is
View SolutionA body of mass 2kg travels according to the law x(t) = pt+qt2+rt3 where , q = 4ms−2 , p = 3ms−1 and r=5ms−3. The force acting on the body at t = 2s is
View Solution