The acceleration of a particle which starts from rest varies with time according to relation a=2t+3. The velocity of the particle at time t would be
2t3+3
t2+3t
2t2+3t
zero
The correct Answer is:B
To find the velocity of a particle whose acceleration varies with time according to the relation a=2t+3, we can follow these steps:
Step 1: Understand the relationship between acceleration and velocity
Acceleration a is defined as the rate of change of velocity with respect to time. Mathematically, this is expressed as:
a=dvdt
Step 2: Substitute the given acceleration into the equation
From the problem, we have:
a=2t+3
So we can write:
dvdt=2t+3
Step 3: Rearrange the equation for integration
We can rearrange this equation to isolate dv:
dv=(2t+3)dt
Step 4: Integrate both sides
Now we integrate both sides. The left side integrates with respect to v and the right side with respect to t:
∫dv=∫(2t+3)dt
The left side gives:
v
The right side can be integrated as follows:
∫(2t+3)dt=∫2tdt+∫3dt=t2+3t+C
where C is the constant of integration.
Step 5: Determine the constant of integration
Since the particle starts from rest, we know that at t=0, the velocity v=0:
v(0)=0=02+3(0)+C⟹C=0
Step 6: Write the final expression for velocity
Substituting C back into the equation gives us:
v=t2+3t
Conclusion
Thus, the velocity of the particle at time t is:
v(t)=t2+3t
---
Acceleration a is defined as the rate of change of velocity with respect to time. Mathematically, this is expressed as:
a=dvdt
From the problem, we have:
a=2t+3
So we can write:
dvdt=2t+3
We can rearrange this equation to isolate dv:
dv=(2t+3)dt
Now we integrate both sides. The left side integrates with respect to v and the right side with respect to t:
∫dv=∫(2t+3)dt
v
∫(2t+3)dt=∫2tdt+∫3dt=t2+3t+C
where C is the constant of integration.
Since the particle starts from rest, we know that at t=0, the velocity v=0:
v(0)=0=02+3(0)+C⟹C=0
Substituting C back into the equation gives us:
v=t2+3t
Thus, the velocity of the particle at time t is:
v(t)=t2+3t
Topper's Solved these Questions
Similar Questions
The acceleration of a'particle starting from rest, varies with time according to the relation a=kt+c. The velocity of the particle after time t will be :
View SolutionThe acceleration a of a particle starting from rest varies with time according to relation, a=αt+β. Find the velocity of the particle at time instant t.
Strategy : a=dvdtView SolutionA particle starts from rest and moves with an acceleration of a={2+|t−2|}m/s2 The velocity of the particle at t=4 sec is
View SolutionA particle starts from rest and moves with an acceleration of a={2+|t−2|}m/s2 The velocity of the particle at t=4 sec is
View SolutionStarting from rest, the acceleration of a particle is a=2(t-1). The velocity of the particle at t=5s is :-
View SolutionThe acceleration of a particle varies with time t seconds according to the relation a=6t+6ms−2. Find velocity and position as funcitons of time. It is given that the particle starts from origin at t=0 with velocity 2ms−1.
View SolutionA particle starts from rest at t=0 and moves in a straight line with an acceleration as shown below. The velocity of the particle at t=3 s is
 View Solution
View SolutionTangental acceleration of a particle moving in a circle of radius 1 m varies with time t as shown in figure (initial velocity of the particle is zero). Time after which total acceleration of particle makes an angle of 30∘ with radial acceleration is
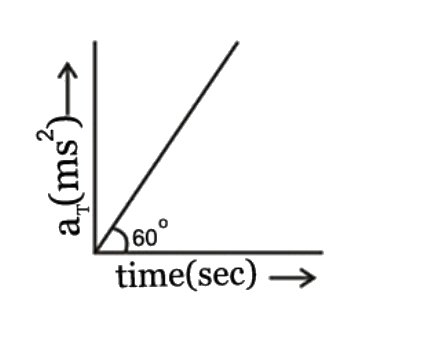 View Solution
View SolutionThe acceleratin of a particle increases linearly with time t as 6t. If the initial velocity of the particles is zero and the particle starts from the origin, then the distance traveled by the particle in time t will be
View SolutionThe position x of a particle varies with time t according to the relation x=t3+3t2+2t. Find the velocity and acceleration as functions of time.
View Solution

