If a moving particle have linear momentum ¯¯¯P and position vector ¯r then choose the correct relation between ¯r,¯p and angular momentum ¯¯¯L of particle about the origin.
¯r.¯¯¯L=0
∣∣¯r.¯¯¯L∣∣=0
¯¯¯L.¯¯¯P≠0
∣∣¯¯¯L.¯¯¯P∣∣=0
The correct Answer is:A
To solve the problem, we need to establish the relationship between the position vector ¯r, linear momentum ¯p, and angular momentum ¯L of a particle about the origin.
1. Understanding Angular Momentum:
The angular momentum ¯L of a particle about a point (in this case, the origin) is defined as:
¯L=¯rׯp
where ¯r is the position vector of the particle and ¯p is the linear momentum of the particle.
2. Properties of Cross Product:
The cross product ¯rׯp has a special property: it is always perpendicular to both ¯r and ¯p. This means that:
¯L⋅¯r=0
This indicates that the angular momentum vector is orthogonal to the position vector.
3. Dot Product with Angular Momentum:
Since ¯L=¯rׯp, we can also express the relationship in terms of the dot product:
¯r⋅¯L=¯r⋅(¯rׯp)=0
This confirms that the dot product of the position vector ¯r with the angular momentum ¯L is zero.
4. Conclusion:
From the above steps, we can conclude that the correct relation between the position vector ¯r, linear momentum ¯p, and angular momentum ¯L is:
¯r⋅¯L=0
Therefore, the correct answer from the options provided is:
Option A: ¯r⋅¯L=0
The angular momentum ¯L of a particle about a point (in this case, the origin) is defined as:
¯L=¯rׯp
where ¯r is the position vector of the particle and ¯p is the linear momentum of the particle.
The cross product ¯rׯp has a special property: it is always perpendicular to both ¯r and ¯p. This means that:
¯L⋅¯r=0
This indicates that the angular momentum vector is orthogonal to the position vector.
Since ¯L=¯rׯp, we can also express the relationship in terms of the dot product:
¯r⋅¯L=¯r⋅(¯rׯp)=0
This confirms that the dot product of the position vector ¯r with the angular momentum ¯L is zero.
From the above steps, we can conclude that the correct relation between the position vector ¯r, linear momentum ¯p, and angular momentum ¯L is:
¯r⋅¯L=0
Therefore, the correct answer from the options provided is:
Option A: ¯r⋅¯L=0
Similar Questions
Knowledge Check
Consider a particle of mass m having linear momentum →p at position →r relative to the origin O. Let →L be the angular momentum of the particle with respect to the origin. Which of the following equations correctly relate(s) →r, →p and →L?
Ad→Ldt+→r×d→pdt=0Bd→Ldt+d→rdt×→p=0Cd→Ldt−d→rdt×→p=0Dd→Ldt−→r×d→pdt=0
What is the relation between wavelength and momentum of moving particles ?
View SolutionA particle has a linear momentum p and position vector r. the angular momentum of this particle about the origin will not be zero under the conditions
View SolutionA particle with the position vector r has linear momentum p. Which of the following statements is true is respect of its angular momentum L about the origin ?
View SolutionA particle moves in space such that its position vector varies as →r=2tˆi+3t2ˆj. If mass of particle is 2 kg then angular momentum of particle about origin at t=2 sec is
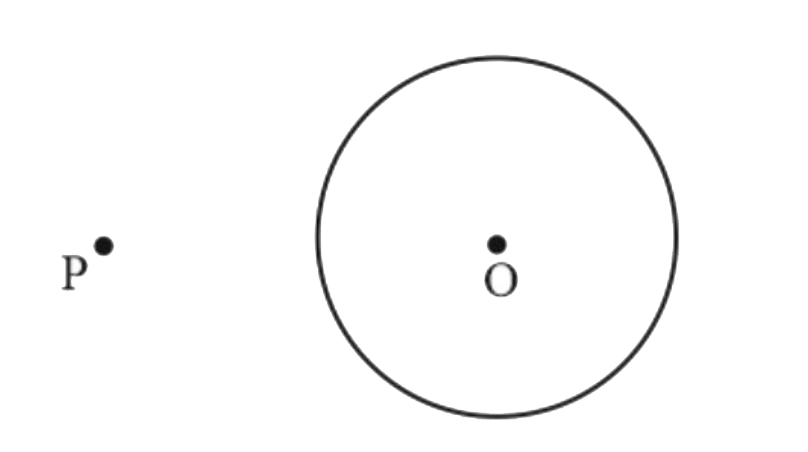
View SolutionConsider a particle , moving in a circle with constant speed . P is a point outside the circle , in the same plane . Then , the angular momentum of the particle about the point P is
View SolutionThe z component of the angular momentum of a particle whose position vector is
View SolutionIf A and B are two mutually exclusive events, then the relation between P(¯¯¯A)andP(B) is
View Solution