What is the relation between wavelength and momentum of moving particles ?
To find the relation between the wavelength and momentum of moving particles, we can start with the de Broglie hypothesis, which states that every moving particle or object has an associated wave-like nature.
1. Understanding the Concepts:
- Wavelength (λ) is the distance between successive peaks of a wave.
- Momentum (P) of a particle is defined as the product of its mass (m) and velocity (v):
P=mv
2. De Broglie's Hypothesis:
- According to de Broglie, the wavelength associated with a moving particle is inversely proportional to its momentum. This can be expressed mathematically as:
λ=hP
where h is Planck's constant (approximately 6.626×10−34Js).
3. Substituting Momentum:
- We can substitute the expression for momentum into the equation:
λ=hmv
This shows that as the momentum increases (either by increasing mass or velocity), the wavelength decreases.
4. Conclusion:
- The relationship indicates that for particles with higher momentum, the associated wavelength is shorter, and for particles with lower momentum, the wavelength is longer. This relationship is fundamental in quantum mechanics and illustrates the wave-particle duality of matter.
Final Relation:
Thus, the relation between wavelength (λ) and momentum (P) of moving particles is given by:
λ=hP
- Wavelength (λ) is the distance between successive peaks of a wave.
- Momentum (P) of a particle is defined as the product of its mass (m) and velocity (v):
P=mv
- According to de Broglie, the wavelength associated with a moving particle is inversely proportional to its momentum. This can be expressed mathematically as:
λ=hP
where h is Planck's constant (approximately 6.626×10−34Js).
- We can substitute the expression for momentum into the equation:
λ=hmv
This shows that as the momentum increases (either by increasing mass or velocity), the wavelength decreases.
- The relationship indicates that for particles with higher momentum, the associated wavelength is shorter, and for particles with lower momentum, the wavelength is longer. This relationship is fundamental in quantum mechanics and illustrates the wave-particle duality of matter.
Thus, the relation between wavelength (λ) and momentum (P) of moving particles is given by:
λ=hP
Similar Questions
What is relation between
_S01_028_Q01.png)
View SolutionTwo particle are initially moving with angular momentum →L1 and →L2 in a region of space with no external torque. A constant external torque →τ then acts on one particle, but not on the other particle, for a time interval Δt. What is the change in the total angular momentum of the two particles ?
View SolutionDerive the relation between the wavelength (λ) of the de broglie wave and kinetic energy (E) of a moving particle
View SolutionIf the maximum particle velocity is 4 times of the wave velocity then relation between wavelength and amplitude is
View SolutionIf a moving particle have linear momentum ¯¯¯P and position vector ¯r then choose the correct relation between ¯r,¯p and angular momentum ¯¯¯L of particle about the origin.
View Solution(i) State how de - Broglie wavelength (λ) of moving particles varies with their linear momentum (p).
(ii) State any one phenomenon in which moving particles exhibit wave nature.
View SolutionTwo particles of equal masses moving with same momentum collide perfectly inelastically. After the collision the combined mass moves with half of the speed of the individual masses. The angle between the initial momenta of individual particle is
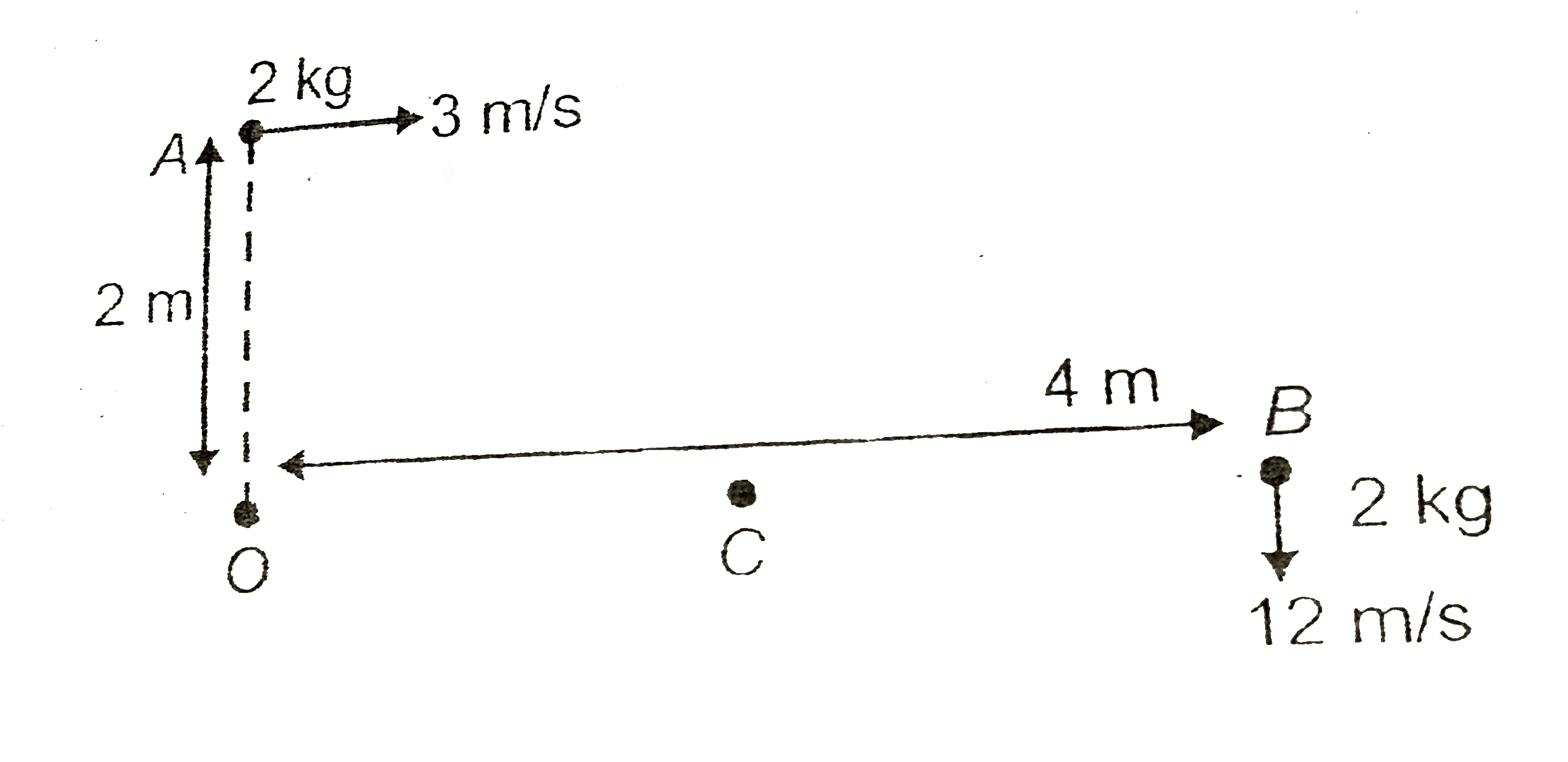
View SolutionThree particles are moving as shown in the figure, Calculate the angular momentum of the third particle C, so that the angular momentum of system about point O becomes zero.
View SolutionThe relation between wavelength (λ) and angular wave number (k) of a wave is
View SolutionA test particle is moving in a circular orbit in the gravitational field produced by a mass density ρ(r)=Kr2. Indentify the correct relation between the radius R of the particle's orbit and its period T :
View Solution
