A spherical shell of mass m and radius R is rolling up without slipping on a rough inclined plane as shown in the figure. The direction of static friction acting on the shell is
Downwards along the inclined plane
Upward along the inclined plane
May be upwards or downward along the inclined plane
Static friction will not act
The correct Answer is:A
To determine the direction of static friction acting on a spherical shell rolling up an inclined plane, we can follow these steps:
1. Identify the Motion of the Shell:
The spherical shell is rolling up the inclined plane. This means that it is moving in the upward direction along the incline.
2. Understand the Forces Acting on the Shell:
The forces acting on the shell include:
- The gravitational force (mg), which can be resolved into two components:
- Perpendicular to the incline: mgcos(θ)
- Parallel to the incline (down the slope): mgsin(θ)
- The normal force (N) acting perpendicular to the incline.
- The static friction force (f) acting along the incline.
3. Determine the Direction of Acceleration:
Since the shell is rolling up the incline, it is decelerating in the upward direction. This means that the net force acting on the shell must be directed down the incline.
4. Apply Newton's Second Law:
For the shell to roll up without slipping, the static friction must provide the necessary torque to prevent slipping. The direction of static friction must oppose the motion of the center of mass of the shell.
5. Analyze the Torque:
The static friction force must act in the direction that produces a clockwise torque about the center of mass. Since the shell is rolling up, the friction must act down the incline to create a clockwise rotation.
6. Conclusion:
Therefore, the direction of static friction acting on the shell is downwards along the inclined plane.
Final Answer:
The direction of static friction acting on the shell is downwards along the inclined plane.
---
The spherical shell is rolling up the inclined plane. This means that it is moving in the upward direction along the incline.
The forces acting on the shell include:
- The gravitational force (mg), which can be resolved into two components:
- Perpendicular to the incline: mgcos(θ)
- Parallel to the incline (down the slope): mgsin(θ)
- The normal force (N) acting perpendicular to the incline.
- The static friction force (f) acting along the incline.
Since the shell is rolling up the incline, it is decelerating in the upward direction. This means that the net force acting on the shell must be directed down the incline.
For the shell to roll up without slipping, the static friction must provide the necessary torque to prevent slipping. The direction of static friction must oppose the motion of the center of mass of the shell.
The static friction force must act in the direction that produces a clockwise torque about the center of mass. Since the shell is rolling up, the friction must act down the incline to create a clockwise rotation.
Therefore, the direction of static friction acting on the shell is downwards along the inclined plane.
The direction of static friction acting on the shell is downwards along the inclined plane.
Similar Questions
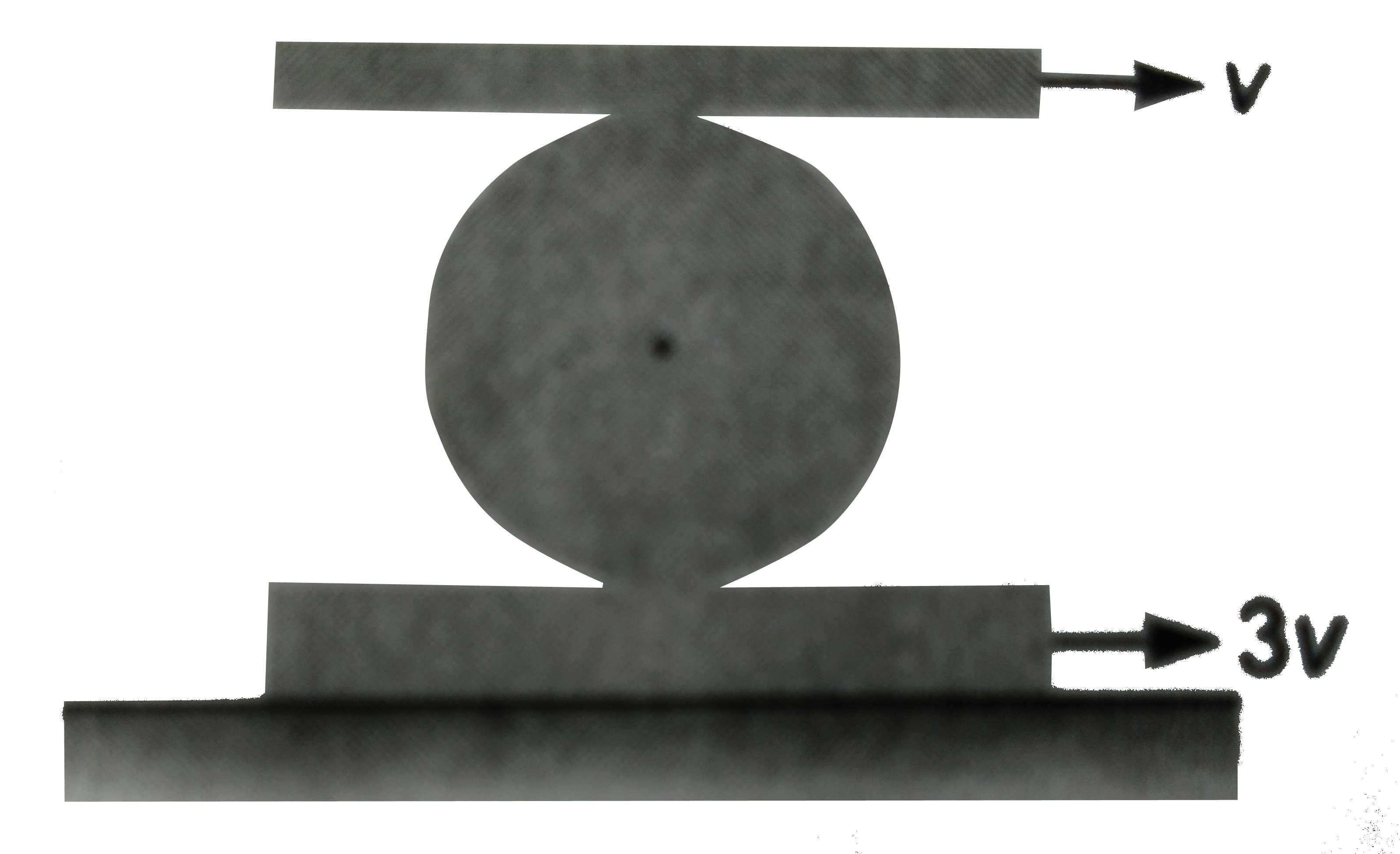
A cylinder of radius R is to roll without slipping between two planks as shown in the figure. Then
View SolutionA uniform thin spherical shell of mass m and radius R rolls down without slipping over an inclined plane of angle θ with the horizontal. The ratio of rotational kinetic energy to the total kinetic energy of the shell will be
View Solution
Knowledge Check
A solid cylinder of mass M and radius R rolls without slipping down an inclined plane making an angle 6 with the horizontal. Then its acceleration is.
A1/3 g sinθB2/3 g sinθC2/5 g sinθD2/7 g sinθ
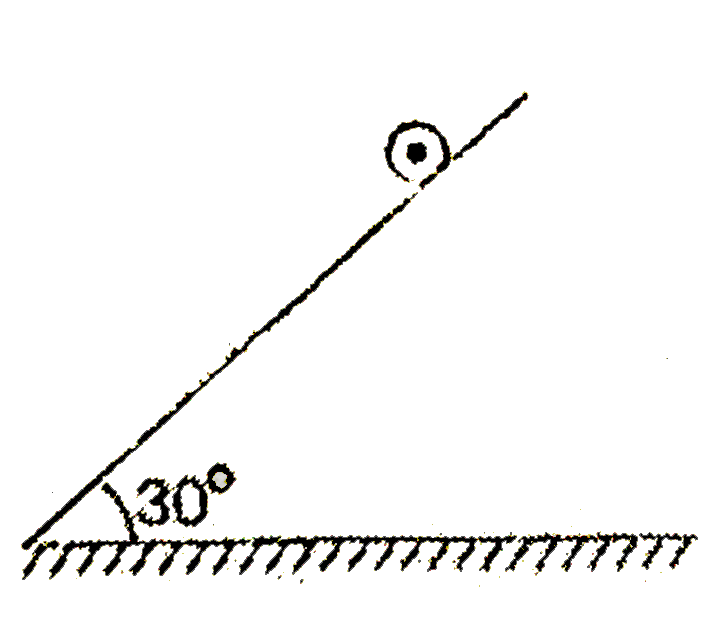
An uniform spherical shell of mass m and radius R starts from rest with pure rolling on long inclined plane as shown in figure. The angular momentum of shell about point of contact after 1 s of its starting is KmR. Determine the value of k(g=10m/s2).
View SolutionA drum of radius R and mass M, rolls down without slipping along an inclined plane of angle θ. The frictional force-
View SolutionA disc of mass m and radius R is rolling without slipping as shown in the figure. The magnitude of net velocity of the point P is (OP=R2)
View SolutionA hollow sphere of mass m and radius R is rolling downdard on a rough inclined plane of inclination θ. If the coefficient of friction between the hollow sphere and incline is μ, then
View SolutionA 1 Kg solid sphere rolls without slipping on a rough horizontal suface as shown in figure. Select incorrect alternative
View SolutionA sphere of mass m rolls without slipping on an inclined plane of inclination θ. Find the linear acceleration of the sphere and the force of friction acting on it. What should be the minimum coefficient of static friction to support pure rolling?
View Solution
A solid sphere of mass m and radius R is rolling without slipping as shown in figure. Find angular momentum of the sphere about z-axis.
View Solution
