A positive charge q is enclosed by a Guassian spherical surface of radius 'a' . If its radius is increased to 4a then the net outward flux will
Become four time
Become two times
Become sixteen times
Remain same
To solve the problem, we will use Gauss's law, which states that the electric flux (Φ) through a closed surface is directly proportional to the charge (Q) enclosed within that surface. The mathematical expression for Gauss's law is:
Φ=Qε0
where:
- Φ is the electric flux,
- Q is the charge enclosed,
- ε0 is the permittivity of free space.
1. Identify the charge enclosed:
- In this problem, we have a positive charge q enclosed by a Gaussian surface.
2. Calculate the electric flux for the initial radius:
- For the Gaussian surface of radius a, the electric flux is given by:
Φa=qε0
3. Consider the change in the radius of the Gaussian surface:
- When the radius of the Gaussian surface is increased to 4a, the charge q is still enclosed within this new surface.
4. Calculate the electric flux for the new radius:
- For the Gaussian surface of radius 4a, the electric flux remains:
Φ4a=qε0
5. Compare the two flux values:
- Since both calculations yield the same result, we conclude that the electric flux does not depend on the size of the Gaussian surface but only on the charge enclosed.
6. Final conclusion:
- Therefore, the net outward flux when the radius is increased to 4a remains the same as when the radius was a:
Φ4a=Φa=qε0
Final Answer:
The net outward flux will remain the same.
- Φ is the electric flux,
- Q is the charge enclosed,
- ε0 is the permittivity of free space.
- In this problem, we have a positive charge q enclosed by a Gaussian surface.
- For the Gaussian surface of radius a, the electric flux is given by:
Φa=qε0
- When the radius of the Gaussian surface is increased to 4a, the charge q is still enclosed within this new surface.
- For the Gaussian surface of radius 4a, the electric flux remains:
Φ4a=qε0
- Since both calculations yield the same result, we conclude that the electric flux does not depend on the size of the Gaussian surface but only on the charge enclosed.
- Therefore, the net outward flux when the radius is increased to 4a remains the same as when the radius was a:
Φ4a=Φa=qε0
The net outward flux will remain the same.
Similar Questions
A charge Q is enclosed by a Gaussian spherical surface of radius R. If the radius is doubled, then the outward electric flux will
View SolutionDefine electric flux. Write its SI unit. A charge q is enclosed by a spherical surface of radius R. If the radius is reduced to half, how would the electric flux through the surface change ?
View SolutionA charge Q is enclosed by a Gaussian surface. If surface area is doubled and charge Q is tripled then the outward electric flux will
View SolutionConsider a Gaussian spherical surface covering a dipole of charge q and -q, then

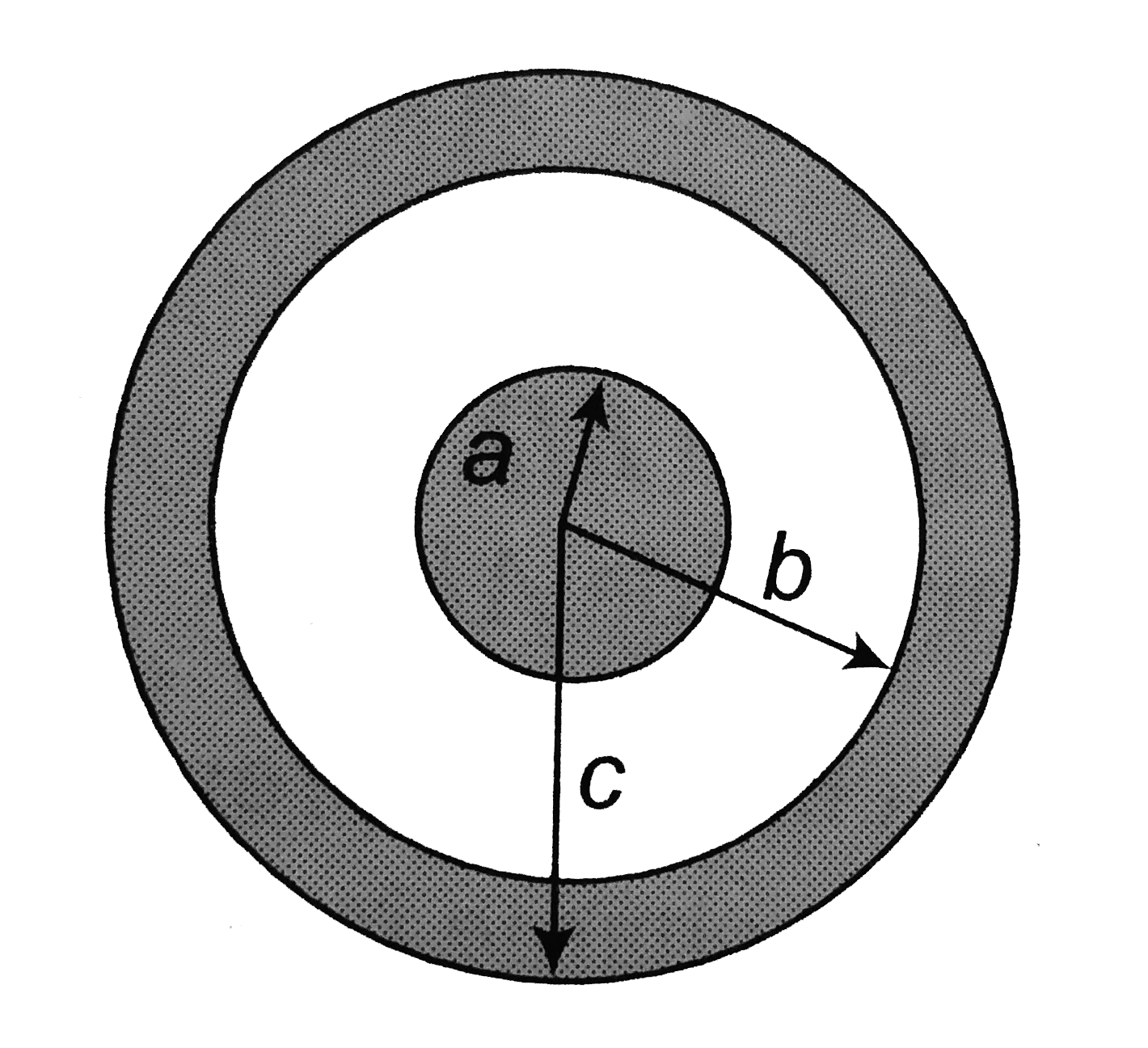
View SolutionA solid conducting sphere of radius a has a net positive charge 2Q. A conducting spherical shell of inner radius b and outer radius c is concentric with the solid sphere and has a net charge −Q. The surface charge density on the inner and outer surfaces of the spherical shell will be
View SolutionA point charge +q is placed at the centre of a conducting spherical shell of inner radius a and outer radius b. Find the charge appearing on the inner and outer surface of the shell.
View SolutionHow does the electric flux due to a point charge enclosed by a spherical Gaussian surface get a affected when its radius is increased ?
View SolutionA small sphere of radius r1 and charge q1 is enclosed by a spherical shell of radius r2 and charge q2. Show that if q1 is positive, charge will necessarily flow from the sphere to the shell (when the two are connected by a wire) no matter what the charge q2 on the shell is [Fig]
View SolutionA charge 'Q' is placed at the centre of a hemispherical surface of radius 'R'. The flux of electric field due to charge 'Q' through the surface of hemisphere is
View SolutionAn infinite, uniformly charged sheet with surface charge density σ cuts through a spherical Gaussian surface of radius R at a distance X from its center, as shown in the figure. The electric flux Φ through the Gaussian surface is .

View Solution
