In a simple Atwood's machine, two unequal masses m1=5kg , m2=2kg are connected by a string going over a clamped light smooth pulley. Now a constant force F = 1 N is applied on each mass in vertically downward direction. The ratio of acceleration of either block before and after applying the force will be
717
25
1
34
To solve the problem, we need to analyze the two scenarios: before and after applying the constant force F=1N on both masses.
Step 1: Analyze the system before applying the force.
Let m1=5kg and m2=2kg. The acceleration due to gravity is g.
- For mass m1 (5 kg):
Net force=m1g−T=m1a
This gives us:
5g−T=5a(Equation 1)
- For mass m2 (2 kg):
Net force=T−m2g=m2a
This gives us:
T−2g=2a(Equation 2)
Step 2: Solve the equations to find acceleration a before applying the force.
Adding Equation 1 and Equation 2:
(5g−T)+(T−2g)=5a+2a
This simplifies to:
5g−2g=7a
3g=7a⟹a=3g7
Step 3: Analyze the system after applying the force.
Now, a downward force F=1N is applied to both masses.
- For mass m1 (5 kg):
Net force=m1g+F−T=m1a
This gives us:
5g+1−T=5a(Equation 3)
- For mass m2 (2 kg):
Net force=T−(m2g+F)=m2a
This gives us:
T−(2g+1)=2a(Equation 4)
Step 4: Solve the equations to find acceleration a after applying the force.
Adding Equation 3 and Equation 4:
(5g+1−T)+(T−2g−1)=5a+2a
This simplifies to:
5g−2g+1−1=7a
3g=7a⟹a=3g7
Step 5: Calculate the ratio of accelerations.
The acceleration before applying the force is a1=3g7 and after applying the force is a2=3g7.
Thus, the ratio of the accelerations is:
Ratio=a1a2=3g73g7=1
Final Answer:
The ratio of acceleration of either block before and after applying the force is 1.
---
Net force=m1g−T=m1a
This gives us:
5g−T=5a(Equation 1)
Net force=T−m2g=m2a
This gives us:
T−2g=2a(Equation 2)
(5g−T)+(T−2g)=5a+2a
This simplifies to:
5g−2g=7a
3g=7a⟹a=3g7
Net force=m1g+F−T=m1a
This gives us:
5g+1−T=5a(Equation 3)
Net force=T−(m2g+F)=m2a
This gives us:
T−(2g+1)=2a(Equation 4)
(5g+1−T)+(T−2g−1)=5a+2a
This simplifies to:
5g−2g+1−1=7a
3g=7a⟹a=3g7
Ratio=a1a2=3g73g7=1
The ratio of acceleration of either block before and after applying the force is 1.
Similar Questions
Two unequal masses are connected by a very light string over a clamped light smooth pulley. Find the acceleration of the system and the tension in the string.
View SolutionIn a simple Atwood machine, two unequal masses m1andm2 are connected by string going over a clamped light smooth pulley . In a typical arrangement m1=300g and m2=600g. The system is released from rest. (a) Find the distance travelled by the first block in first two seconds. (b) Find the tension in the string. (c) Find the force exerted by clamp on the pulley.
View SolutionTwo unequal masses of 1kg and 2kg are connected by a string going over a clamed light smooth pulley as shown in figure . The system is released from rest .The larger mass is stopped for a moment 1.0s after the system is set in motion.Find the time elapsed before the string is tight again.

View SolutionTwo unequal masses of 1kg and 2kg are connected by an inextensible light string passing over a smooth pulley as shown in figure. A force F=20N is applied on 1kg block find the acceleration of either block (g=10m/s2).

View SolutionTwo bodies of masses 1 kg and 2 kg are connected by a very light string passed over a clamped light smooth pulley. If the system is released from rest, find the acceleration of the two masses and the tension in the string
View SolutionTwo blocks of masses 2 kg and 4 kg are connected by a massles string which is just taut. Now two forces 3 N and 14 N are applied on blocks. The tension in the string is
View SolutionTwo balls of masses m1=5kg,m2=10kg are connected by a light inextensible string. A horizontal force F = 15 N is acting on the ball m2 along the line joining two balls as shown. What is the (a) acceleration of the balls (b) tension in the string?

View SolutionTwo blocks of masses m1=4kg and m2=2kg are connected to the ends of a string which passes over a massless, frictionless pulley. The total downwards thrust on the pulley is nearly

View SolutionTwo bodies of masses m1andm2 are connected by a light string going over a smooth lilght pulley at the end of an incline. The mass1 lies on the incline m2 hangs vertically. The system is t rest. Find the angle of the incline and the fore exerted by the incline on the body of mass m_1.
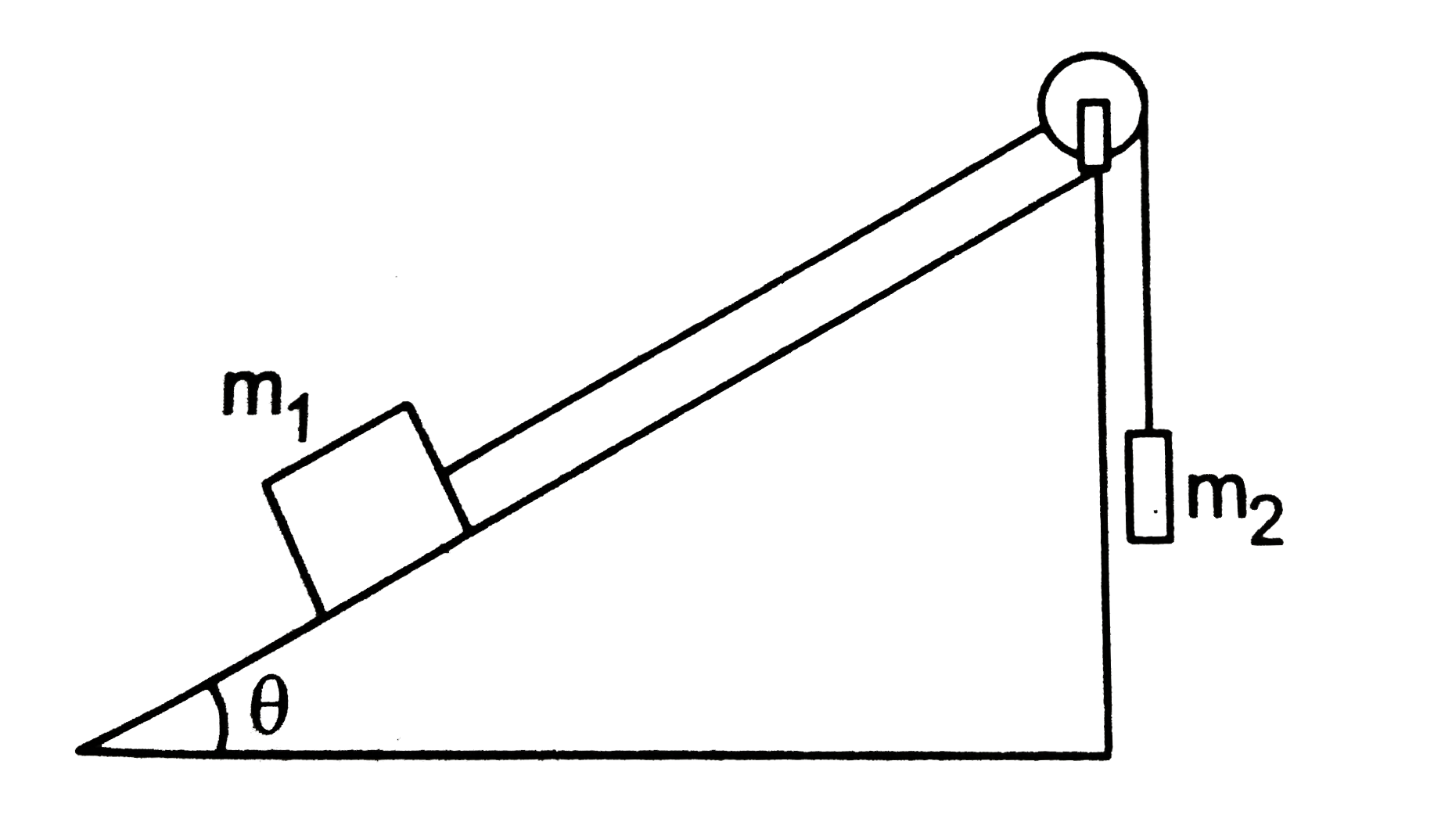
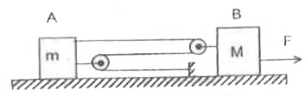
View SolutionTwo blocks A and B of masses m and M are attached with ideal string and pulley, and placed on smooth horizontal surface. Force F is applied horizontally as shown in figure. Find the ratio of the acceleration of A to that of B.
View Solution
