Four pointed charges are placed on vertices of a square.If all the charges are brought to any one of the vertices.The change in potential at the centre of square due to this would be
Dependent on polarity of charges
A non-zero constant
Zero
Infinite
The correct Answer is:C
To solve the problem, we need to determine the change in electric potential at the center of a square when four point charges located at the vertices are moved to one vertex.
1. Identify the Charges and Configuration:
- Let the four charges be Q1,Q2,Q3, and Q4.
- The charges are initially located at the vertices of a square with side length a.
2. Calculate the Initial Potential at the Center:
- The distance from each vertex of the square to the center is given by a√2.
- The potential V at the center due to a point charge Q is given by the formula:
V=kQr
where k is Coulomb's constant and r is the distance from the charge to the point where the potential is being calculated.
- Therefore, the total potential at the center due to all four charges is:
Vinitial=kQ1a√2+kQ2a√2+kQ3a√2+kQ4a√2
- Simplifying this, we have:
Vinitial=k√2a(Q1+Q2+Q3+Q4)
3. Rearranging Charges to One Vertex:
- Now, we move all charges Q1,Q2,Q3, and Q4 to one vertex of the square.
- The distance from this vertex to the center remains the same, a√2.
4. Calculate the New Potential at the Center:
- The potential at the center due to the charges now located at one vertex is:
Vnew=kQ1a√2+kQ2a√2+kQ3a√2+kQ4a√2
- This simplifies to:
Vnew=k√2a(Q1+Q2+Q3+Q4)
5. Determine the Change in Potential:
- Since both the initial and new potential expressions are identical:
Vinitial=Vnew
- Therefore, the change in potential ΔV is:
ΔV=Vnew−Vinitial=0
Conclusion:
The change in potential at the center of the square when all charges are brought to one vertex is zero.
- Let the four charges be Q1,Q2,Q3, and Q4.
- The charges are initially located at the vertices of a square with side length a.
- The distance from each vertex of the square to the center is given by a√2.
- The potential V at the center due to a point charge Q is given by the formula:
V=kQr
where k is Coulomb's constant and r is the distance from the charge to the point where the potential is being calculated.
- Therefore, the total potential at the center due to all four charges is:
Vinitial=kQ1a√2+kQ2a√2+kQ3a√2+kQ4a√2
- Simplifying this, we have:
Vinitial=k√2a(Q1+Q2+Q3+Q4)
- Now, we move all charges Q1,Q2,Q3, and Q4 to one vertex of the square.
- The distance from this vertex to the center remains the same, a√2.
- The potential at the center due to the charges now located at one vertex is:
Vnew=kQ1a√2+kQ2a√2+kQ3a√2+kQ4a√2
- This simplifies to:
Vnew=k√2a(Q1+Q2+Q3+Q4)
- Since both the initial and new potential expressions are identical:
Vinitial=Vnew
- Therefore, the change in potential ΔV is:
ΔV=Vnew−Vinitial=0
The change in potential at the center of the square when all charges are brought to one vertex is zero.
Similar Questions
Four point charges −Q,−q,2q and 2Q are placed, one at each corner of the square. The relation between Q and q for which the potential at the centre of the square is zero is
View SolutionFour point charges −Q,−q,2q and 2Q are placed, one at each corner of the square. The relation between Q and q for which the potential at the centre of the square is zero is
View Solution
Knowledge Check
A hexagon of side 8 cm has a charge 4μC at each of its vertices. The potential at the centre of the hexagon is
A2.7×106VB7.2×1011VC2.5×1012VD3.4×104VFour particles each of mass m are placed at the vertices of a square of side l. the potential at the centre of square is
A−√2Gm2l(2−1√2)B−2Gm2l(2+1√2)C−√2Gm2l(√2−1√2)D−2Gm2l(√2−1√2)
Four point charges are placed at the corners of a square of side l. Calculate potential at the centre of square.

View SolutionFour point charges are placed at the corners of a square ABCD of side 10 cm, as shown in figure. The force on a charge of 1μC placed at the centre of square is

View SolutionFour particles each of mass 'm' are placed at the four vertices of a square 'a' .Find net force on any one the particle.
View SolutionFour equal charges are kept fixed on the vertices of a square of side 'a'. A charge q1 is kept at the centre of the square as shown in the figure . Then
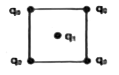
View SolutionFour point charges each +q is placed on the circumference of a circle of diameter 2d in such a way that they form a square. The potential at the centre is
View SolutionCharges are placed on the vertices of a square as shown. Let E r be the electric field and V the potential at the centre. If the charges on A and B are interchanged with those on D and C respectively, then :-
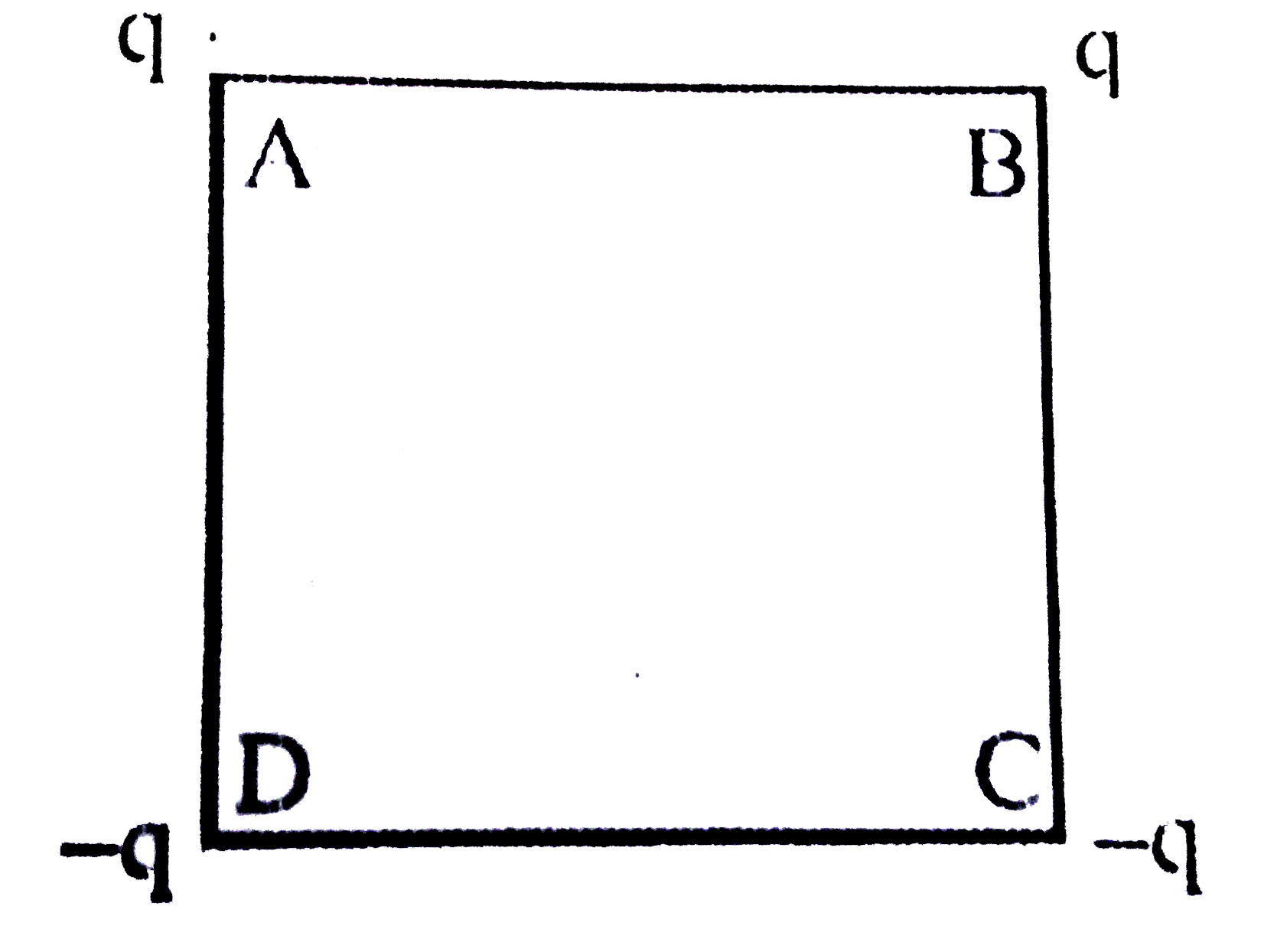
View Solution
