Two immiscible liquid of densities rho and 3ρ are put in a container. The height of each liquid is h. A solid cylinder of length L and density d is put in this container. The cylinder floats completely inside liquids with its axis vertical and length L3 in the denser liquid. The density d is equal to
5ρ3
3ρ5
2ρ3
4ρ
To solve the problem, we need to apply the principles of buoyancy and the concept of equilibrium for the floating solid cylinder in two immiscible liquids.
1. Understanding the Setup:
- We have two immiscible liquids: one with density ρ (less dense) and the other with density 3ρ (denser).
- Each liquid has a height h.
- A solid cylinder of length L and density d is placed in the container, floating vertically.
2. Identifying the Lengths:
- The cylinder is submerged such that L3 is in the denser liquid (density 3ρ).
- Therefore, the remaining length submerged in the less dense liquid is L−L3=2L3.
3. Calculating the Buoyant Force:
- The buoyant force acting on the cylinder is equal to the weight of the liquid displaced by the submerged part of the cylinder.
- The volume of the part submerged in the denser liquid is:
V1=Area×L3
- The buoyant force from the denser liquid is:
Fb1=V1×Densitydenser×g=(Area×L3)×(3ρ)×g=Area×L×ρ×g
- The volume of the part submerged in the less dense liquid is:
V2=Area×2L3
- The buoyant force from the less dense liquid is:
Fb2=V2×Densityless dense×g=(Area×2L3)×ρ×g=Area×2L3×ρ×g
4. Total Buoyant Force:
- The total buoyant force Fb acting on the cylinder is the sum of the buoyant forces from both liquids:
Fb=Fb1+Fb2=Area×L×ρ×g+Area×2L3×ρ×g
- Simplifying gives:
Fb=Area×g×ρ×(L+2L3)=Area×g×ρ×5L3
5. Weight of the Cylinder:
- The weight of the cylinder W is given by:
W=Volume×Density×g=Area×L×d×g
6. Applying Equilibrium Condition:
- For the cylinder to float, the buoyant force must equal the weight of the cylinder:
Area×g×ρ×5L3=Area×L×d×g
- Canceling Area and g from both sides gives:
ρ×53=d
7. Final Result:
- Therefore, the density d of the cylinder is:
d=5ρ3
- We have two immiscible liquids: one with density ρ (less dense) and the other with density 3ρ (denser).
- Each liquid has a height h.
- A solid cylinder of length L and density d is placed in the container, floating vertically.
- The cylinder is submerged such that L3 is in the denser liquid (density 3ρ).
- Therefore, the remaining length submerged in the less dense liquid is L−L3=2L3.
- The buoyant force acting on the cylinder is equal to the weight of the liquid displaced by the submerged part of the cylinder.
- The volume of the part submerged in the denser liquid is:
V1=Area×L3
- The buoyant force from the denser liquid is:
Fb1=V1×Densitydenser×g=(Area×L3)×(3ρ)×g=Area×L×ρ×g
V2=Area×2L3
- The buoyant force from the less dense liquid is:
Fb2=V2×Densityless dense×g=(Area×2L3)×ρ×g=Area×2L3×ρ×g
- The total buoyant force Fb acting on the cylinder is the sum of the buoyant forces from both liquids:
Fb=Fb1+Fb2=Area×L×ρ×g+Area×2L3×ρ×g
- Simplifying gives:
Fb=Area×g×ρ×(L+2L3)=Area×g×ρ×5L3
- The weight of the cylinder W is given by:
W=Volume×Density×g=Area×L×d×g
- For the cylinder to float, the buoyant force must equal the weight of the cylinder:
Area×g×ρ×5L3=Area×L×d×g
- Canceling Area and g from both sides gives:
ρ×53=d
- Therefore, the density d of the cylinder is:
d=5ρ3
Similar Questions
Two non-mixing liquids of densities ρ and nρ (n>1) are put in a container. The height of each liquid is h. A solid cylinder of length L and density d is put in this container. The cylinder floats with its axis vertical and length pL(p<1) in the denser liquid. The density d is equal to :
View SolutionTwo immiscible liquids of densities ρ and 2ρ and thickness (heights) h and 2h, respectively, push a vertical plate. Find the total side thrust given by the liquids on the vertical plate.
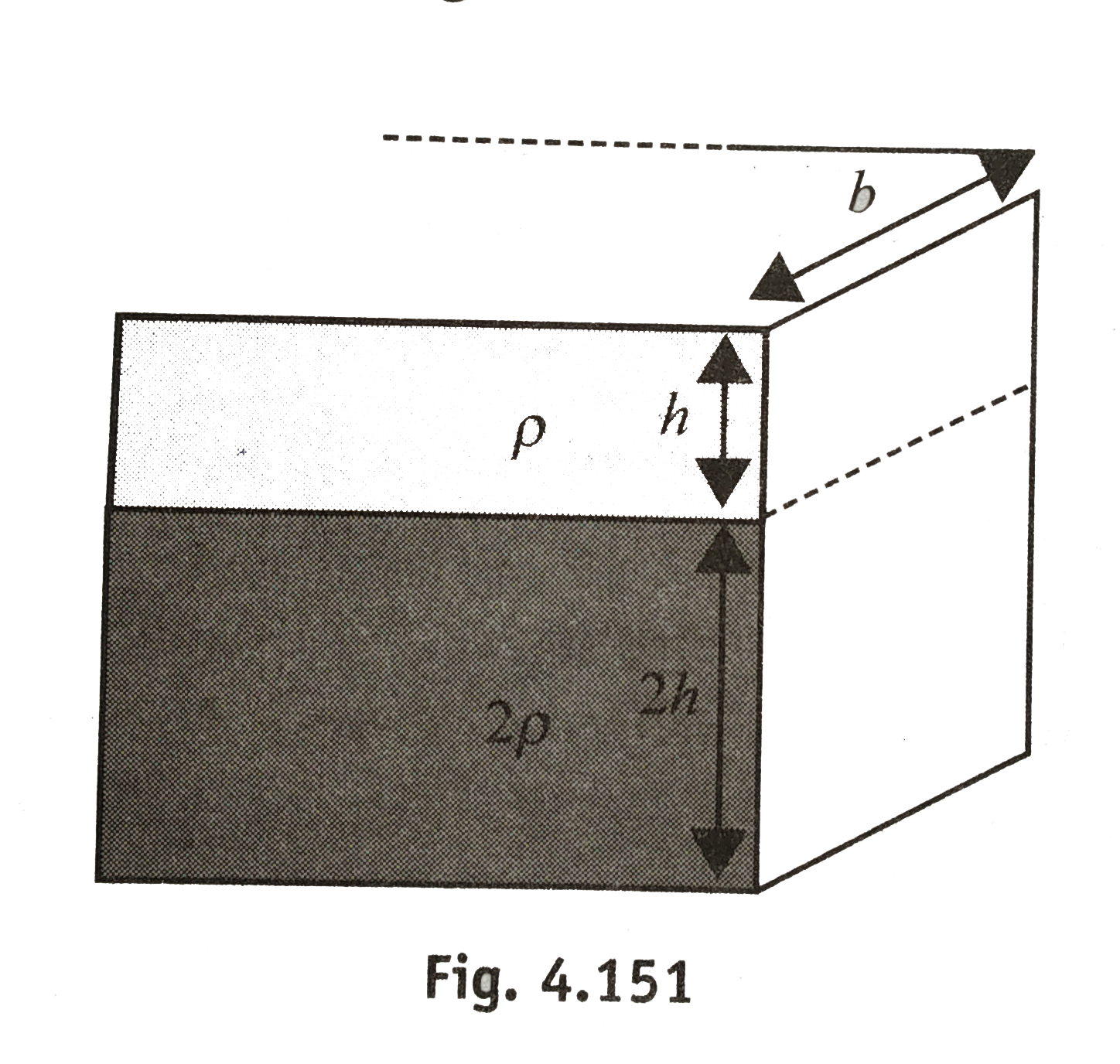
View Solution
Knowledge Check
The pressure inside a liquid of density ρ at a depth h is :
AhρgBhρgChρgDhρ
A container of large uniform cross-sectional area A resting on a horizontal surface, holes two immiscible, non-viscous and incompressible liquids of densities d and 2d each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0. A homogeneous solid cylinder of length L(L<H/2) and cross-sectional area A/5 is immersed such that it floats with its axis vertical at the liquid-liquid interface with length L/4 in the denser liquid,
The density D of the material of the floating cylinder is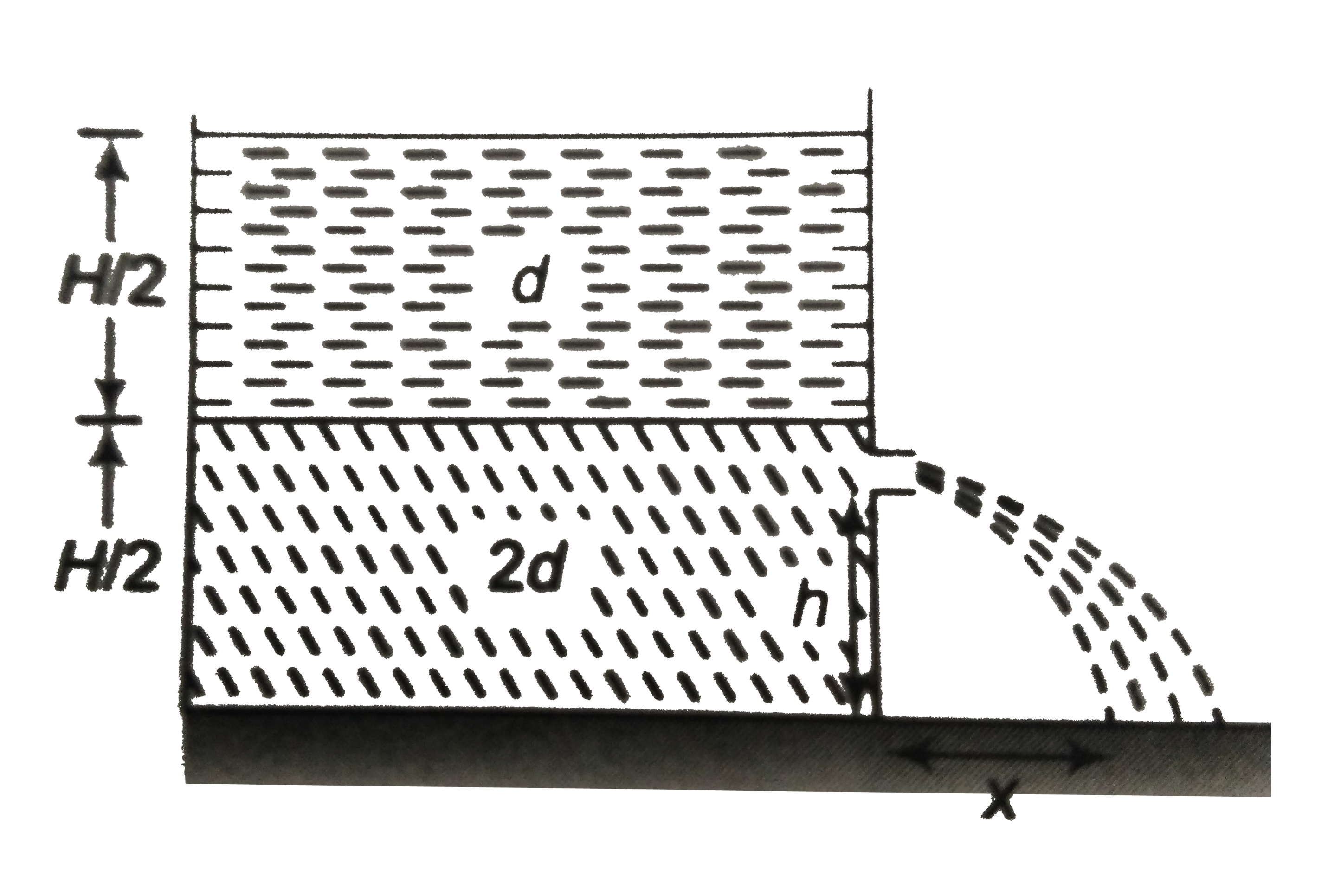
View SolutionIf liquid of density ρ is filled in an open cylindrical container to height h, then pressure at bottom
View SolutionA container of a large uniform cross-sectional area A resting on a horizontal surface holds two immiscible, non viscous and incompressible liquids of densities d and 2d, each of height H/2 as shown in figure. The lower density liquid is open to atmosphere. A homogeneous solid cylinder of length L(L<H2), cross-sectional area A/5 is immersed such that it floats with its axis vertical of the liquid-liquid interface with length L/4 denser liquid. Determine
(a) density D of the solid and
(b) the total pressure at the bottom of the container. (Atmospheric pressure =P0).
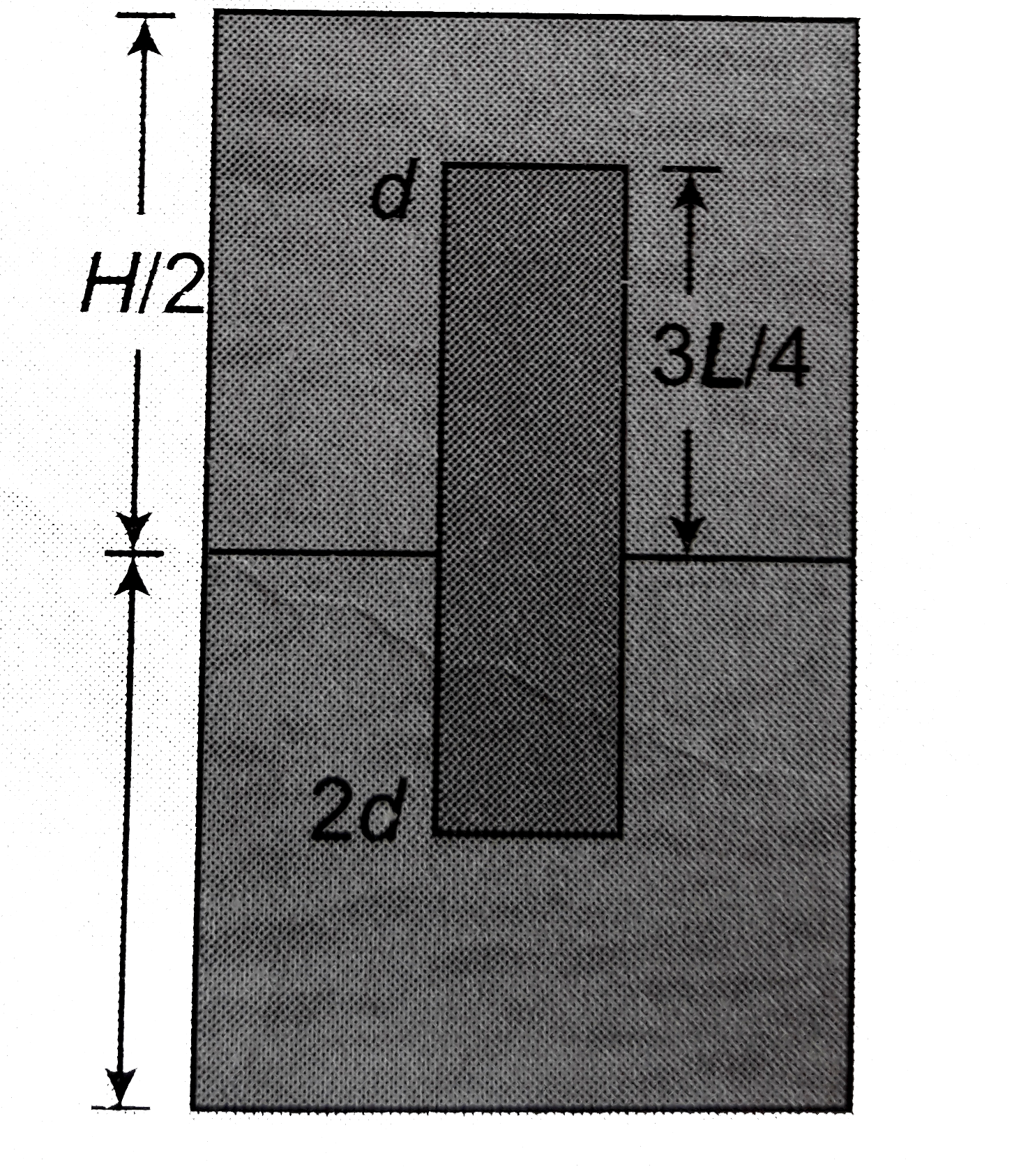 .
.
View SolutionA block of density ρ floats in a liquid with its one third volume immersed. The density of the liquid is
View SolutionA container of large uniform cross-sectional area A resting on a horizontal surface, holes two immiscible, non-viscon and incompressible liquids of densities d and 2d each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0. A homogeneous solid cylinder of length L(L<H/2) and cross-sectional area A/5 is immeresed such that it floats with its axis vertical at the liquid-liquid interface with length L/4 in the denser liquid,
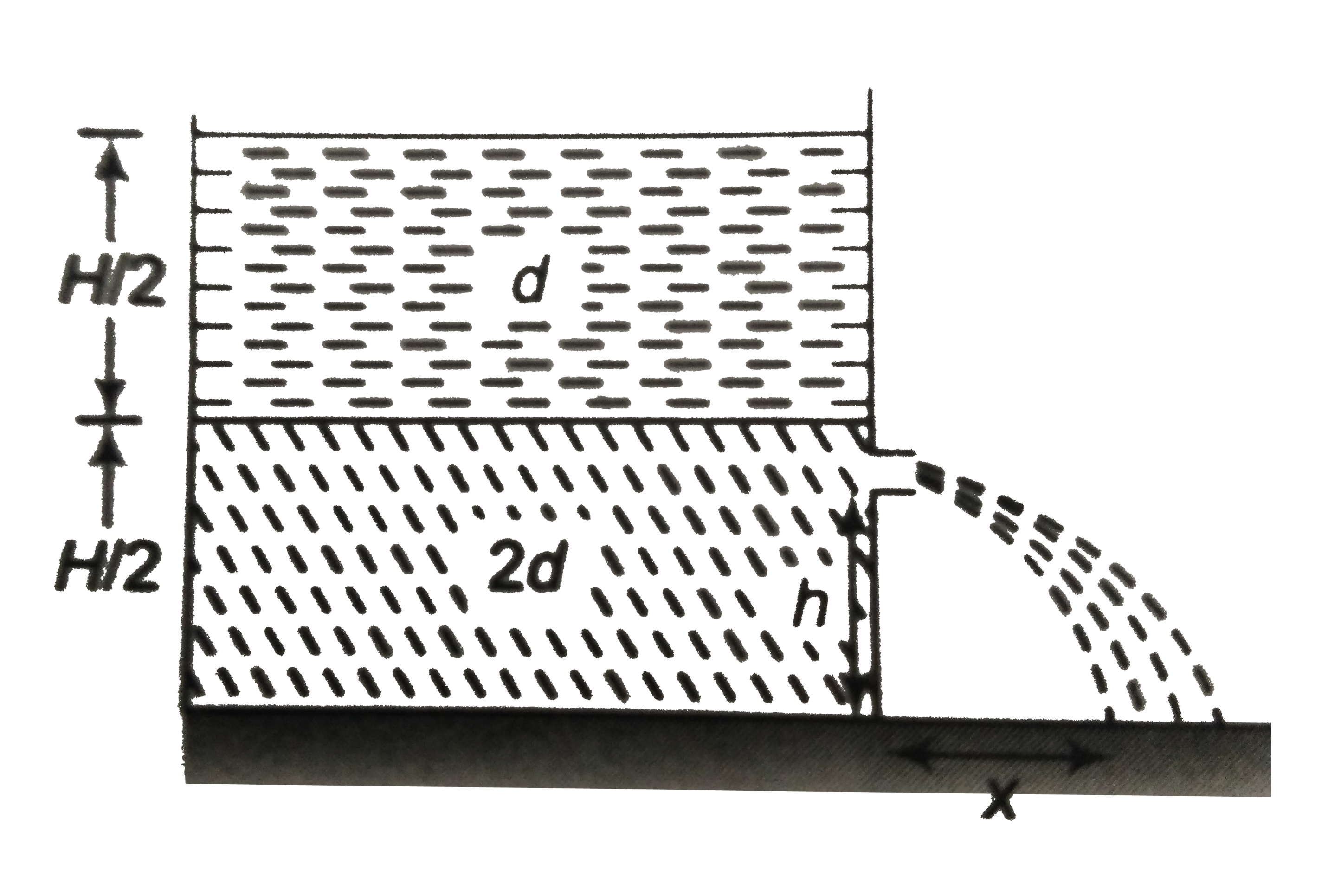
The cylinder is then removed and the original arrangement is restroed. a tiny hole of area s(s<<A) is punched on the vertical side of the container at a height h(h<H/2). As a result of this, liquid starts flowing out of the hole with a range x on the horizontal surface.
The initial speed of efflux without cylinder is
View SolutionA container of large uniform cross-sectional area A resting on a horizontal surface, holes two immiscible, non-viscon and incompressible liquids of densities d and 2d each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0. A homogeneous solid cylinder of length L(L<H/2) and cross-sectional area A/5 is immeresed such that it floats with its axis vertical at the liquid-liquid interface with length L/4 in the denser liquid,
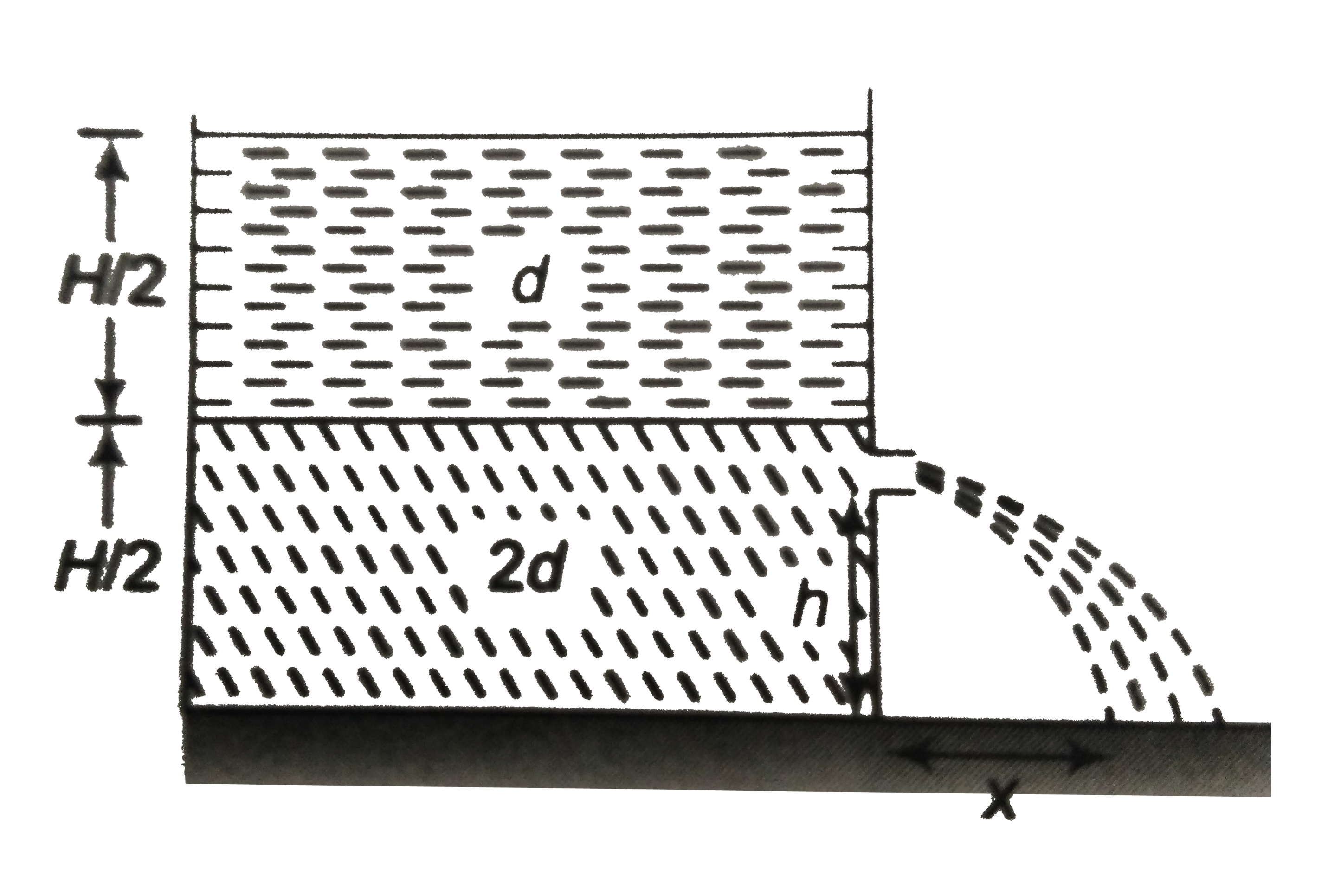
The cylinder is then removed and the original arrangement is restroed. a tiny hole of area s(s<<A) is punched on the vertical side of the container at a height h(h<H/2). As a result of this, liquid starts flowing out of the hole with a range x on the horizontal surface.
The initial value of x is
View SolutionFluids at rest exert a normal force to the walls of the container or to the sruface of the Body immersed in the fluid. The pressure exerted by this force at a point inside the liqid is the sum of atmospheric pressure and a factor which depends on the density of the liquid, the acceleration due to gravity and the height of the liquid, above that point. The upthrust acting on a body immersed in a stationary liquid is the net force acting on the body in the upward direction. A number of phenomenon of liquids in motion can be explain by Bernoulli's theorem which relates the pressure, flow speed and height for flow of an ideal incompressible fluid.
A container of large uniform corss sectional area. A resting on a horizontal surface holds two immiscible, non viscous and incompressile liquids of densities d and 2d, each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0.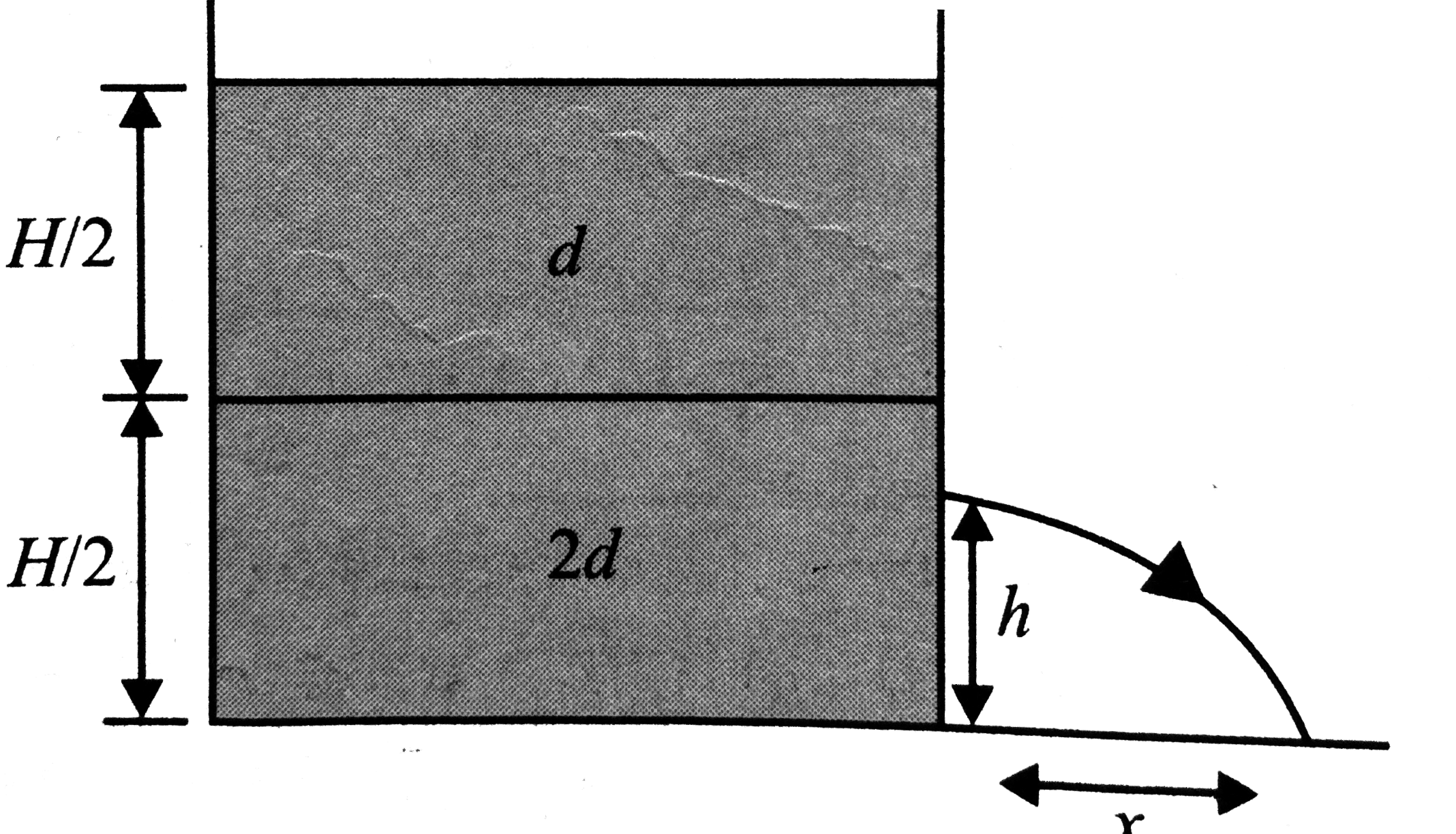
Situation I:
A homogeneous solid cylinder of length L(L<H/2). cross sectional area A/5 is immersed such that it floats with its axis vertical at liquid -liquid interface with lenght L/4 in the denser liquid.
The density of the solid is
View Solution
