In given figure, current I is flowing through straight wire and divided equally in two loops as shown in the figure. The magnitude of magnetic field intensity at the center (O) is
Zero
μ0I8[1r2−1r1
μ0I4π[π2r2−π2r1−1r1]
μ0I4π[π2r2−π2r1+πr1]
To find the magnitude of the magnetic field intensity at the center (O) due to the current flowing through the straight wire and divided into two loops, we can follow these steps:
1. Identify the Configuration:
- We have a straight wire carrying a current I that splits into two semicircular loops at point O. Let the radius of the upper semicircle be r1 and the radius of the lower semicircle be r2.
2. Magnetic Field Due to a Semicircular Loop:
- The magnetic field B at the center of a semicircular loop carrying current I is given by the formula:
B=μ0I4R
- For the upper semicircle (radius r1):
B1=μ0I4r1
- For the lower semicircle (radius r2):
B2=μ0I4r2
3. Magnetic Field Due to the Straight Wire:
- The magnetic field B at a distance R from a long straight wire carrying current I is given by:
B=μ0I2πR
- At point O, the distance from the straight wire is R (which can be taken as the average of r1 and r2 if they are equal).
4. Total Magnetic Field at Point O:
- The total magnetic field at point O is the vector sum of the magnetic fields due to the upper semicircle, lower semicircle, and the straight wire. Since the fields from the semicircles will add up and the field from the straight wire will subtract (due to opposite directions), we can write:
Btotal=B1+B2−Bstraight
5. Substituting the Values:
- Substituting the expressions for B1, B2, and Bstraight:
Btotal=μ0I4r1+μ0I4r2−μ0I2πR
6. Simplifying the Expression:
- Combine the terms and simplify to find the total magnetic field at point O.
7. Final Result:
- After simplifying, we will arrive at a final expression for the magnetic field intensity at point O.
- We have a straight wire carrying a current I that splits into two semicircular loops at point O. Let the radius of the upper semicircle be r1 and the radius of the lower semicircle be r2.
- The magnetic field B at the center of a semicircular loop carrying current I is given by the formula:
B=μ0I4R
- For the upper semicircle (radius r1):
B1=μ0I4r1
- For the lower semicircle (radius r2):
B2=μ0I4r2
- The magnetic field B at a distance R from a long straight wire carrying current I is given by:
B=μ0I2πR
- At point O, the distance from the straight wire is R (which can be taken as the average of r1 and r2 if they are equal).
- The total magnetic field at point O is the vector sum of the magnetic fields due to the upper semicircle, lower semicircle, and the straight wire. Since the fields from the semicircles will add up and the field from the straight wire will subtract (due to opposite directions), we can write:
Btotal=B1+B2−Bstraight
- Substituting the expressions for B1, B2, and Bstraight:
Btotal=μ0I4r1+μ0I4r2−μ0I2πR
- Combine the terms and simplify to find the total magnetic field at point O.
- After simplifying, we will arrive at a final expression for the magnetic field intensity at point O.
Similar Questions
In given figure, the current (/) is flowing through straight wire annd divided equally in two loops as shown in the figure. The magnitude field intensity at center (O) is
View SolutionA current - carrying loop is shown in the figure. The magnitude of the magnetic field produced at a point O is
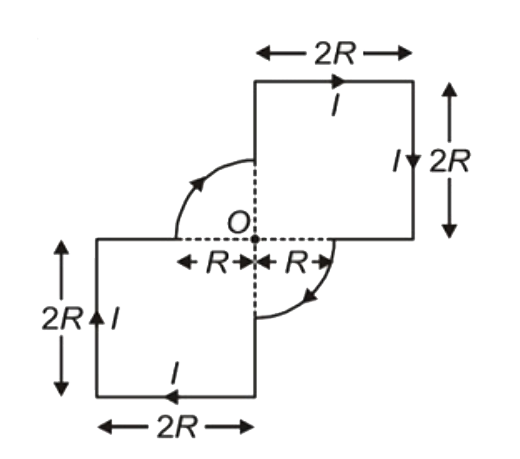
View SolutionA long wire carrying current 'I' is bent into the shape as shown in the figure. The net magnetic field intensity at the centre 'O' is

View SolutionA conducting wire carrying current I is shown in the figure. The magnitude of magnetic field at the point P will be

View SolutionA current I is flowing through the loop , as shown in the figure . The magnetic field at centre O is
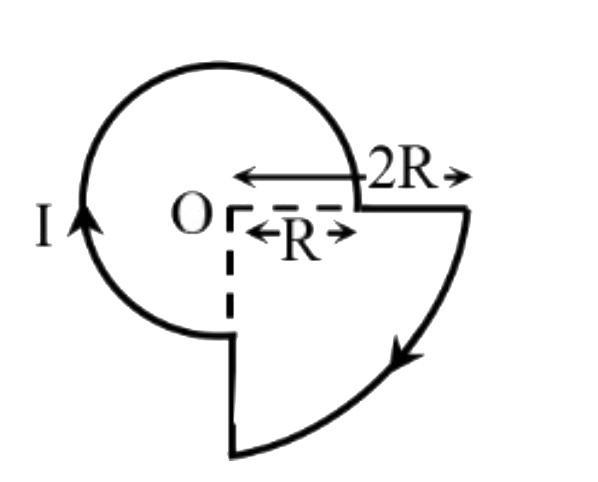
View SolutionA current l flows through a closed loop as shown in figure .The magnetic field at the centre O is
View Solutiona straight wire current element is carrying current 100 A as shown in figure. the magnitude of magnetic field at a point P.

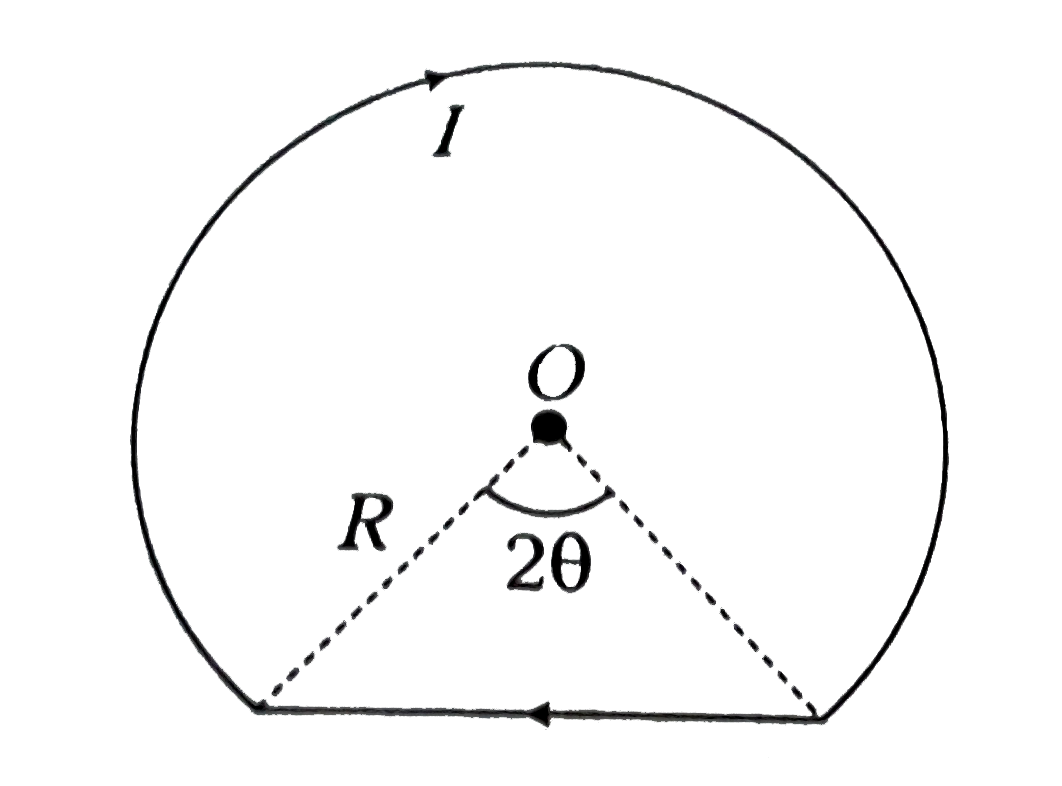
View SolutionThe wire loop shown in figure carries a current as shown. The magnetic field at the centre O is ,

View SolutionA coil having N turns carry a current I as shown in the figure. The magnetic field intensity at point P is
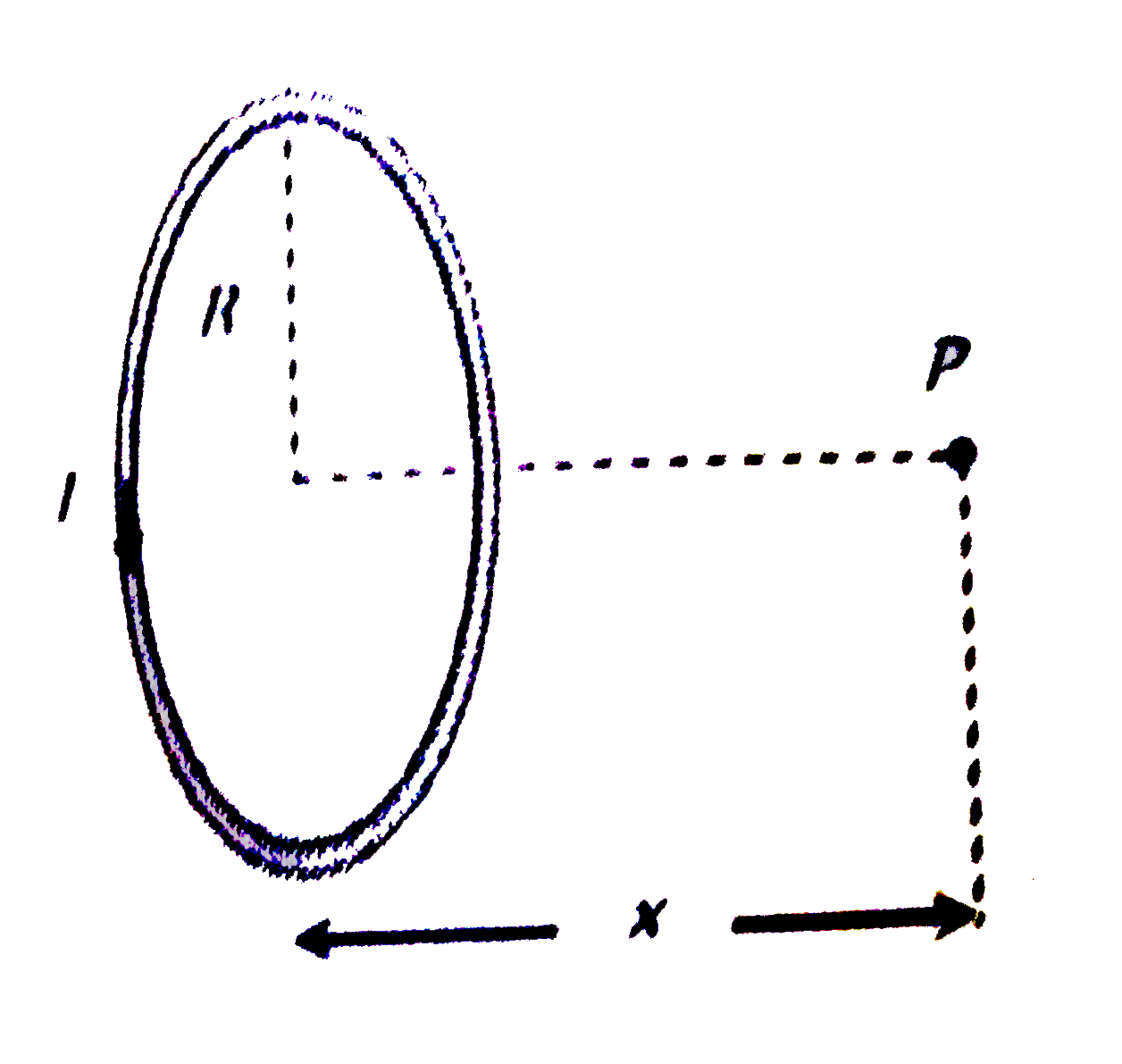
View SolutionA current I is flowing thorugh the loop. The direction of the current and the shpae of the loop are as the shown in the figure. The magnetic fild at the centre of the loop is μ0IR times
(MA=R,MB=2R,∠DMA=90∘)
View Solution
