There is a current carrying straight wire of infinite length having current 5A. If a square loop of side 10 cm is kept coplanar with the wire as shown in figure the magnetic flux to the square loop is
ln(2)×10−8 Wb
5ln(2)×108 Wb
2ln(2)×10−7 Wb
ln(2)×10−7 Wb
To find the magnetic flux through a square loop placed in the vicinity of a straight current-carrying wire, we will follow these steps:
1. Identify the Given Values:
- Current (I) = 5 A
- Side of the square loop (a) = 10 cm = 0.1 m
- Distance from the wire to the loop (d) = 10 cm = 0.1 m
2. Determine the Magnetic Field (B) due to the Infinite Wire:
The magnetic field (B) at a distance (d) from a long straight wire carrying current I is given by the formula:
B=μ0I2πd
where μ0 (the permeability of free space) is approximately 4π×10−7T m/A.
3. Calculate the Magnetic Field at the Distance of the Loop:
Substituting the values into the formula:
B=4π×10−7×52π×0.1
Simplifying this:
B=4×10−7×50.2=20×10−70.2=10×10−6=1×10−5T
4. Calculate the Area (A) of the Square Loop:
The area of the square loop is given by:
A=a2=(0.1)2=0.01m2
5. Determine the Magnetic Flux (Φ):
The magnetic flux (Φ) through the loop is given by:
Φ=B⋅A
Substituting the values:
Φ=1×10−5×0.01=1×10−7Wb
6. Final Result:
The magnetic flux through the square loop is:
Φ=1×10−7Wb
- Current (I) = 5 A
- Side of the square loop (a) = 10 cm = 0.1 m
- Distance from the wire to the loop (d) = 10 cm = 0.1 m
The magnetic field (B) at a distance (d) from a long straight wire carrying current I is given by the formula:
B=μ0I2πd
where μ0 (the permeability of free space) is approximately 4π×10−7T m/A.
Substituting the values into the formula:
B=4π×10−7×52π×0.1
Simplifying this:
B=4×10−7×50.2=20×10−70.2=10×10−6=1×10−5T
The area of the square loop is given by:
A=a2=(0.1)2=0.01m2
The magnetic flux (Φ) through the loop is given by:
Φ=B⋅A
Substituting the values:
Φ=1×10−5×0.01=1×10−7Wb
The magnetic flux through the square loop is:
Φ=1×10−7Wb
Similar Questions
There is a current carrying straight wire of infinite length having current 5 A. If a square loop of side 10 cm is kept coplanar with the wire as shown in figure. The magnetic flux through the squre loop is

View SolutionFor the situation shown in the figure, flux through the square loop is:
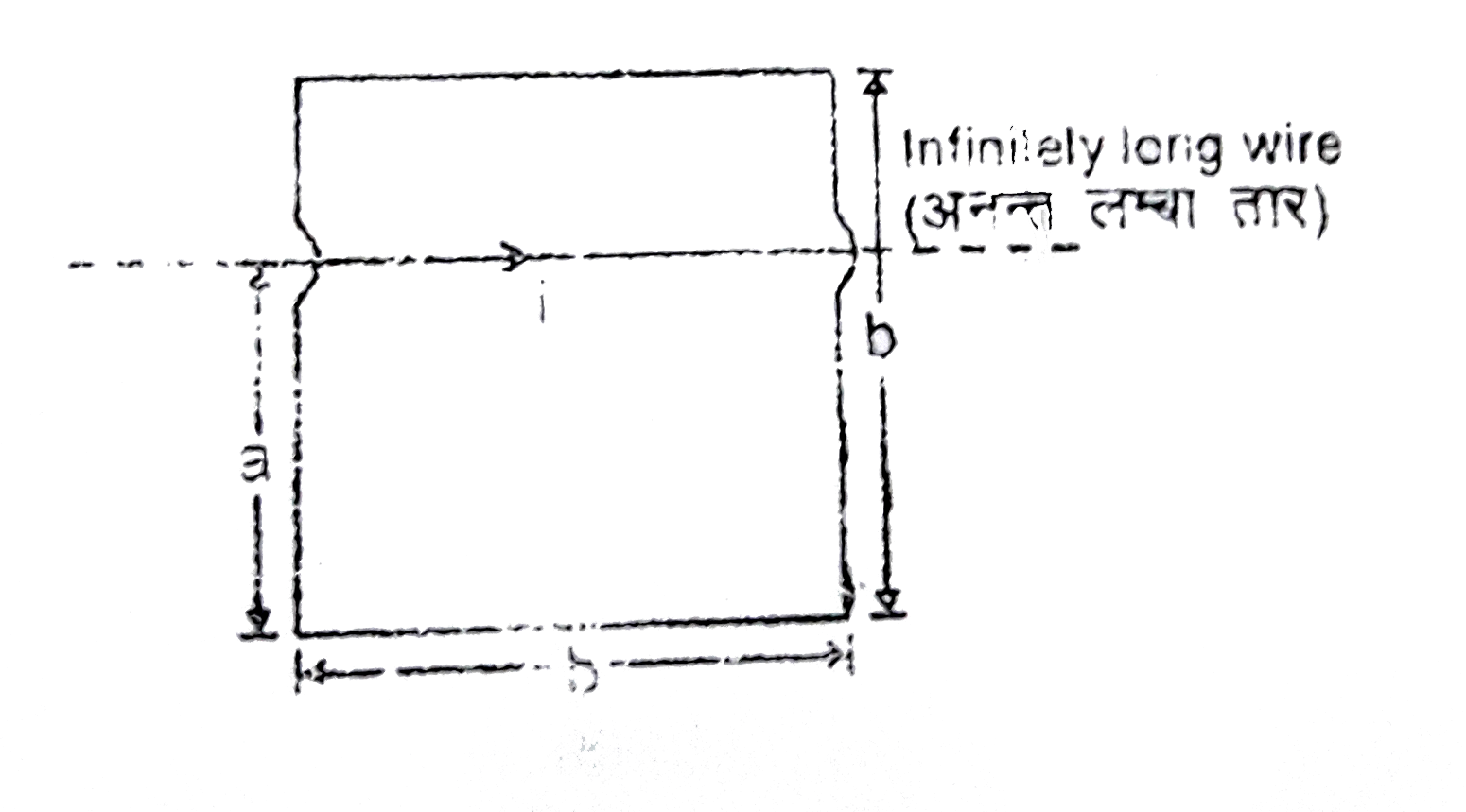
View SolutionA long straight wire is carrying a current of 25 A. A squre loop of side length 10 cm carrying a current of 15 A, is placed 2 cm from the wire as shown in figure. The force on the loop is

View SolutionA square loop OABCO of side of side l carries a current i. It is placed as shown in figure. Find the magnetic moment of loop.

View SolutionA square loop OABCO of side of side l carries a current i. It is placed as shown in figure. Find the magnetic moment of loop.

View SolutionA square loop of length L is made of wire of uniform cross-section carrying current l as shown in figure then magnetic field at centre of square is
View Solution_2019_CBT3_E01_043_Q01.png)
The force between the infinite wire and the square loop is
View SolutionA long wire having a semi-circular loop of radius r carries a current I, as shown in Fig. Find the magnetic field due to entire wire.

View SolutionA current- carrying straight wire is kept along the axis of a circular loop carrying a current. The straight wire
View SolutionA square loop is placed near a long current carrying straight wire as shown in the figure . Match the following table


View Solution