A negatively charged particle enters in a semi-infinite uniform magnetic field vecB, directed out the plane of paper as shown in the figure. Through what angle it is is deviated when it leaves the magnetic field?
45°
0°
135°
90°
To solve the problem of the deviation angle of a negatively charged particle entering a semi-infinite uniform magnetic field, we can follow these steps:
1. Understanding the Setup:
- A negatively charged particle enters a semi-infinite magnetic field directed out of the plane of the paper.
- The magnetic field is uniform, and we need to determine the angle of deviation when the particle exits the magnetic field.
2. Direction of Motion:
- Assume the particle enters the magnetic field at an angle of 45 degrees relative to the boundary of the magnetic field. This means that the initial direction of the particle forms a 45-degree angle with the normal to the boundary of the magnetic field.
3. Lorentz Force:
- The force acting on a charged particle in a magnetic field is given by the Lorentz force equation:
→F=q(→v×→B)
- Here, q is the charge of the particle, →v is the velocity vector of the particle, and →B is the magnetic field vector.
4. Effect of Magnetic Field:
- Since the particle is negatively charged, the direction of the force will be opposite to what it would be for a positively charged particle. The magnetic force will act perpendicular to both the velocity of the particle and the magnetic field.
5. Path of the Particle:
- As the particle moves through the magnetic field, it will experience a centripetal force due to the magnetic field, causing it to move in a circular path. The radius of this path depends on the speed of the particle and the strength of the magnetic field.
6. Exiting the Magnetic Field:
- When the particle exits the semi-infinite magnetic field, it will continue moving in a straight line. The angle at which it exits can be determined by the angle at which it entered the field.
7. Calculating the Deviation Angle:
- Since the particle enters at 45 degrees and the magnetic field causes it to deviate, it will exit at an angle that is the sum of the entry angle and the angle due to the circular motion in the magnetic field.
- The particle will deviate by an additional 45 degrees due to the circular path it follows while in the magnetic field.
8. Final Angle of Deviation:
- Therefore, the total angle of deviation when the particle exits the magnetic field will be:
θ=45∘+45∘=90∘
Conclusion:
The angle through which the negatively charged particle is deviated when it leaves the magnetic field is 90 degrees.
- A negatively charged particle enters a semi-infinite magnetic field directed out of the plane of the paper.
- The magnetic field is uniform, and we need to determine the angle of deviation when the particle exits the magnetic field.
- Assume the particle enters the magnetic field at an angle of 45 degrees relative to the boundary of the magnetic field. This means that the initial direction of the particle forms a 45-degree angle with the normal to the boundary of the magnetic field.
- The force acting on a charged particle in a magnetic field is given by the Lorentz force equation:
→F=q(→v×→B)
- Here, q is the charge of the particle, →v is the velocity vector of the particle, and →B is the magnetic field vector.
- Since the particle is negatively charged, the direction of the force will be opposite to what it would be for a positively charged particle. The magnetic force will act perpendicular to both the velocity of the particle and the magnetic field.
- As the particle moves through the magnetic field, it will experience a centripetal force due to the magnetic field, causing it to move in a circular path. The radius of this path depends on the speed of the particle and the strength of the magnetic field.
- When the particle exits the semi-infinite magnetic field, it will continue moving in a straight line. The angle at which it exits can be determined by the angle at which it entered the field.
- Since the particle enters at 45 degrees and the magnetic field causes it to deviate, it will exit at an angle that is the sum of the entry angle and the angle due to the circular motion in the magnetic field.
- The particle will deviate by an additional 45 degrees due to the circular path it follows while in the magnetic field.
- Therefore, the total angle of deviation when the particle exits the magnetic field will be:
θ=45∘+45∘=90∘
The angle through which the negatively charged particle is deviated when it leaves the magnetic field is 90 degrees.
Similar Questions
If a charged particle enters perpendicular in the uniform magnetic field then
View SolutionWhen a charged particle enters a uniform magnetic field its kinetic energy
View SolutionA charged particle enters a uniform magnetic field perpendicular to it. The magnetic field
View SolutionThe counductor AD moves to the right in a uniform magnetic field directed into the plane of the paper.
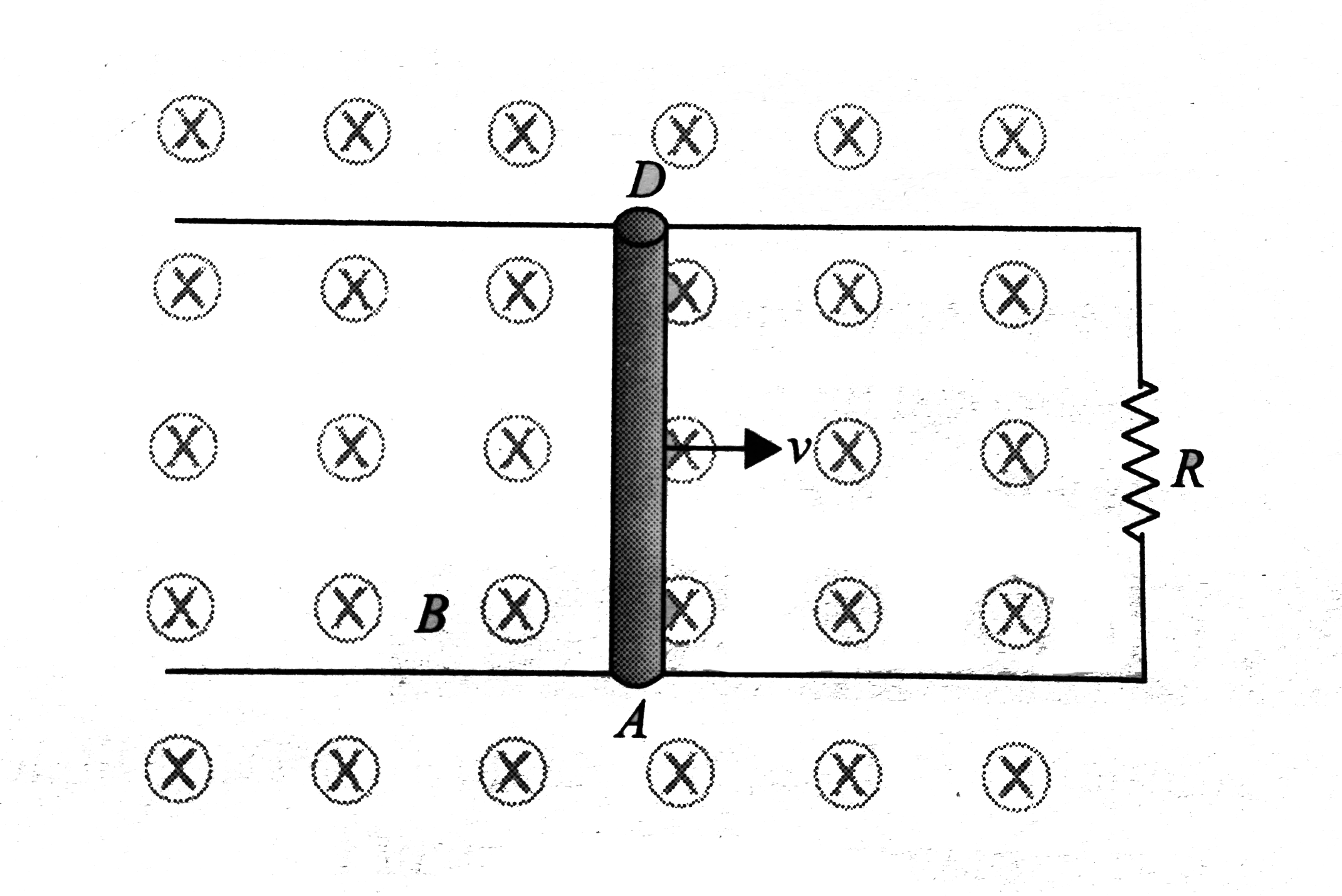
View SolutionA charged particle enters in a uniform magnetic field perpendicular to it. Now match the following two columns.

View SolutionA positively charged particle moving due east enters a region of uniform magnetic field directed vertically upwards. The partical will be
View SolutionThe path of a charged particle moving in a uniform steady magnetic field cannot be a
View SolutionA charged particle is moving in a circular path in uniform magnetic field. Match the following.

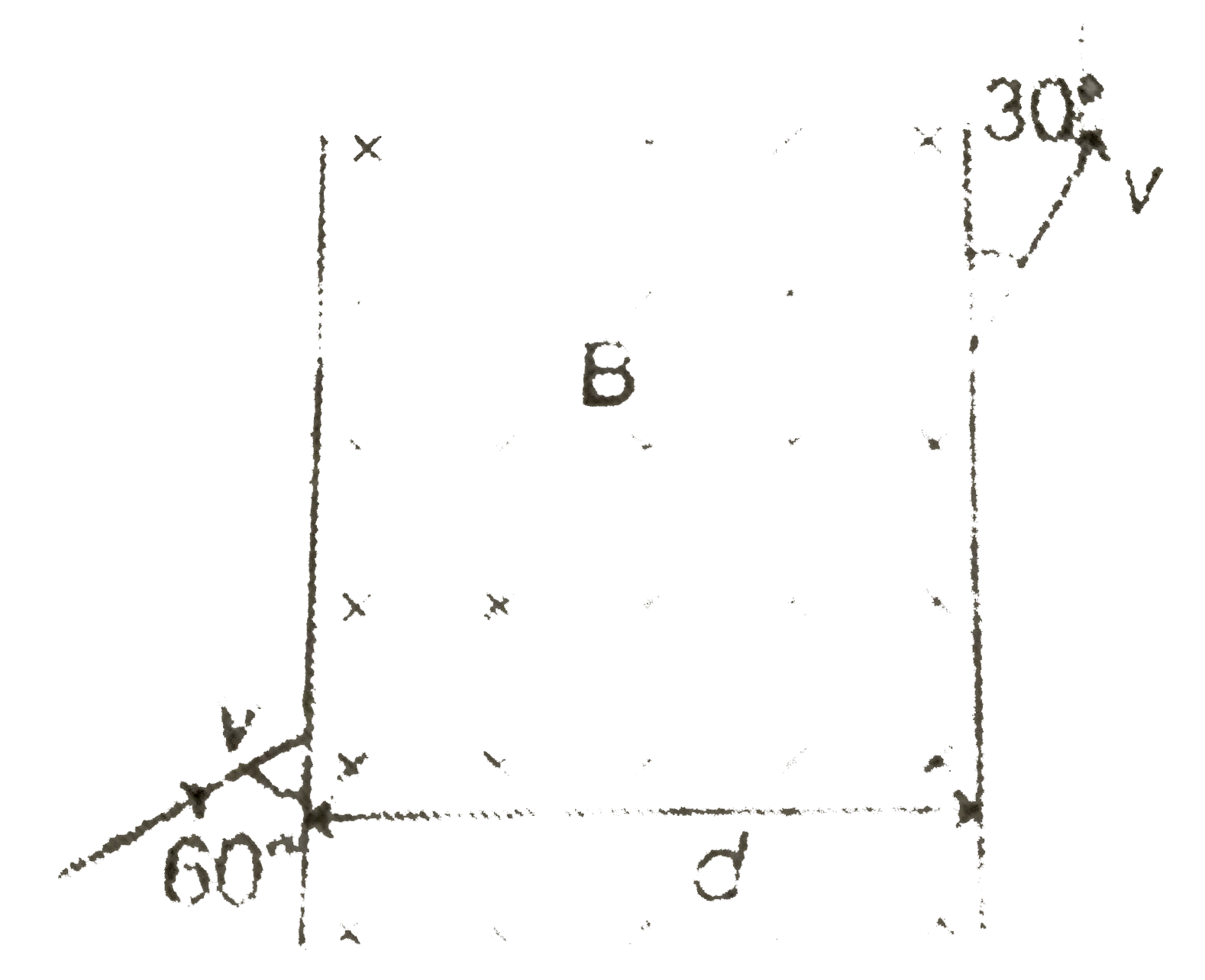
View SolutionA charge particle of charge 'q' and mass 'm' enters in a given magnetic field 'B' and perpendicular to the magnetic field as shown in the figure. It enters at an angle of 60∘ with the boundary surface of magnetic field and comes out at an angle of 30∘ with the boundary surface of the magnetic field as shown in the figure. Find width 'd' in which magnetic field exist.
View SolutionIf a charged particle is a plane perpendicular to a uniform magnetic field with a time period T Then
View Solution
