A verical thin rod is hinged at point P, due to slight push rod starts to rotate.The velocity of centre of mass of rod just after turning angle 0 is [L is length of rod] (## AAK_TST_09_NEET_19_PHY_E09_041_Q001 ##)
0/2
0/2
theta/2
The correct Answer is:B
To solve the problem of finding the velocity of the center of mass of a vertical thin rod hinged at point P after it has been pushed slightly and rotated through an angle θ, we can follow these steps:
1. Identify the System:
The system consists of a vertical thin rod of length L, hinged at point P. When the rod is pushed, it rotates about the hinge.
2. Change in Potential Energy:
When the rod rotates through an angle θ, the center of mass (which is at a distance L/2 from the hinge) rises. The change in height (h) of the center of mass can be expressed as:
h=L2(1−cosθ)
Therefore, the change in potential energy (ΔPE) is given by:
ΔPE=mg⋅h=mg⋅L2(1−cosθ)
3. Kinetic Energy:
The change in potential energy is converted into kinetic energy (KE) of the rod. The moment of inertia (I) of the rod about the hinge is:
I=13mL2
The kinetic energy of the rod when it rotates with angular velocity ω is:
KE=12Iω2=12⋅13mL2⋅ω2=16mL2ω2
4. Equating Potential Energy and Kinetic Energy:
Setting the change in potential energy equal to the kinetic energy gives:
mg⋅L2(1−cosθ)=16mL2ω2
We can cancel m from both sides:
g⋅L2(1−cosθ)=16L2ω2
5. Solving for ω:
Rearranging the equation to solve for ω²:
ω2=3g(1−cosθ)L
6. Velocity of the Center of Mass:
The velocity (v) of the center of mass of the rod can be expressed in terms of ω:
v=ω⋅L2
Substituting ω² into this equation:
v2=⎛⎝√3g(1−cosθ)L⎞⎠2⋅(L2)2=3g(1−cosθ)L⋅L24=3gL4(1−cosθ)
7. Using the Trigonometric Identity:
We can use the identity 1−cosθ=2sin2(θ2) to express the velocity:
v2=3gL4⋅2sin2(θ2)=3gL2sin2(θ2)
Therefore, the final expression for the velocity of the center of mass is:
v=√3gL2⋅sin(θ2)
Final Answer:
The velocity of the center of mass of the rod just after turning an angle θ is:
v=√3gL2⋅sin(θ2)
The system consists of a vertical thin rod of length L, hinged at point P. When the rod is pushed, it rotates about the hinge.
When the rod rotates through an angle θ, the center of mass (which is at a distance L/2 from the hinge) rises. The change in height (h) of the center of mass can be expressed as:
h=L2(1−cosθ)
Therefore, the change in potential energy (ΔPE) is given by:
ΔPE=mg⋅h=mg⋅L2(1−cosθ)
The change in potential energy is converted into kinetic energy (KE) of the rod. The moment of inertia (I) of the rod about the hinge is:
I=13mL2
The kinetic energy of the rod when it rotates with angular velocity ω is:
KE=12Iω2=12⋅13mL2⋅ω2=16mL2ω2
Setting the change in potential energy equal to the kinetic energy gives:
mg⋅L2(1−cosθ)=16mL2ω2
We can cancel m from both sides:
g⋅L2(1−cosθ)=16L2ω2
Rearranging the equation to solve for ω²:
ω2=3g(1−cosθ)L
The velocity (v) of the center of mass of the rod can be expressed in terms of ω:
v=ω⋅L2
Substituting ω² into this equation:
v2=⎛⎝√3g(1−cosθ)L⎞⎠2⋅(L2)2=3g(1−cosθ)L⋅L24=3gL4(1−cosθ)
We can use the identity 1−cosθ=2sin2(θ2) to express the velocity:
v2=3gL4⋅2sin2(θ2)=3gL2sin2(θ2)
Therefore, the final expression for the velocity of the center of mass is:
v=√3gL2⋅sin(θ2)
The velocity of the center of mass of the rod just after turning an angle θ is:
v=√3gL2⋅sin(θ2)
Similar Questions
A rod is hinged at point P, vertically standing upward, Due to slight jerk rod starts to come down. The angular velocity of rod just after turning through angle e is (hinge is smooth)
View SolutionTwo uniform thin rods each of length L and mass m are joined as shown in the figure. Find the distance of centre of mass the system from point O

View SolutionA uniform thin rod of mass m and length l is standing on a smooth horizontal suface. A slight disturbance causes the lower end to slip on the smooth surface and the rod starts falling. Find the velocity of centre of mass of the rod at the instant when it makes an angle θ with horizontal.
View SolutionA homogenous rod of length l=ηx and mass M is lying on a smooth horizontal floor. A bullet of mass m hits the rod at a distance x from the middle of the rod at a velocity v0 perpendicular to the rod and comes to rest after collision. If the velocity of the farther end of the rod just after the impact is in the opposite direction of v0, then:
View SolutionThe density of a thin rod of length l varies with the distance x from one end as ρ=ρ0x2l2. Find the position of centre of mass of rod.
View SolutionA uniform rod of mass m and length L is hinged about one end and can freely rotate in a vertical plane. The angular velocity of the rod, when it falls from position P to Q. through an angle α starting from rest, is

View SolutionA thin rod of mass m and length l is hinged at the lower end to a level floor and stands vertically. Then its upper end will strike the floor with a velocity given by:
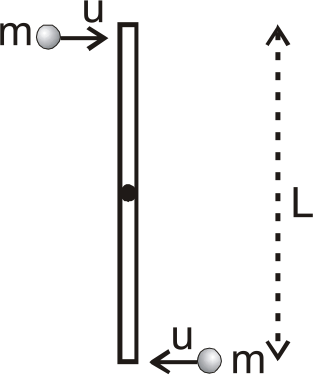
View SolutionA uniform rod of mass 200 grams and length L = 1m is initially at rest in vertical position. The rod is hinged at centre such that it can rotate freely without friction about a fixed horizontal axis passing through its centre. Two particles of mass m = 100 grams each having horizontal velocity of equal magnitude u = 6 m/s strike the rod at top and bottom simultaneously as shown and stick to the rod. Find the angular speed (in rad/sec.) of rod when it becomes horizontal.
View SolutionFind the electric field at a point P on the perpendicular bisector of a uniformly charged rod. The length of the rod is L, the charge on it is Q and the distance of P from the centre of the rod is a.
View SolutionA uniform rod of mass M and length a lies on a smooth horizontal plane. A particle of mass m moving at a speed v perpendicular to the length of the rod strikes it at a distance a4 from the centre and stops after the collision. Find a. the velocity of the cente of the rod and b. the angular velocity of the rod abut its centre just after the collision.
View Solution
