A particles moves in a straight line with uniform acceleration. Its velocity at time t = 0 is v1 and at time t = t is v2. The average velocity of the particle in this time interval is (v1+v2)/2. Is this correct ? Substantiate your answer.
Consider a particle moving with uniform acceleration a.
At t = 0, the (initial) velocity = v1
At t = 0, the (final) velocity = v2
time = t
Acceleration, a=v2−v1t−0 [∵a=v2−v1t2−t1]
Displacement S=v1t+12at2 (∵S=ut+12at2) (##VIKQBPHYXIC03E05002_S01.png width=80%><br>S=v_(1)t+1/2[(v_(2)-v_(1))/t]t^(2)<br>S=t[v_(1)+v_(2)/2-v_(1)/2]=t[(v_(1)+v_(2))/2]S/t=[(v_(1)+v_(2))/2]<br>Avera≥velocity=S/t=(v_(1)+v_(2))/2<br>Hencevalue=(v_(1)+v_(2))/2<br>therefore The given statement is true.
Similar Questions
A particle moves in a straight line with uniform acceleration. Its velocity at time t=0 is v1 and at time t=t is v2. The average velocity of the particle in this time interval is v1+v22 . Is this statement true or false?
View SolutionA particle moves in a straight line with uniform acceleration. Its velocity at time t=0 is v1 and at time t=t is v2. The average velocity of the particle in this time interval is v1+v22 . Is this statement true or false?
View Solution
Knowledge Check
- Question 1 - Select One
For body moving with uniform acceleration a, initial and final velocities in a time interval t are u and v respectively. Then its average velocity in the time interval t is
A(v+at)B(v−at2)C(v−at)D(u−at2) - Question 2 - Select One or More
A particle is moving in a straight line according to graph given below : (a is acceleration, v is velocity, x is position, t is time) :
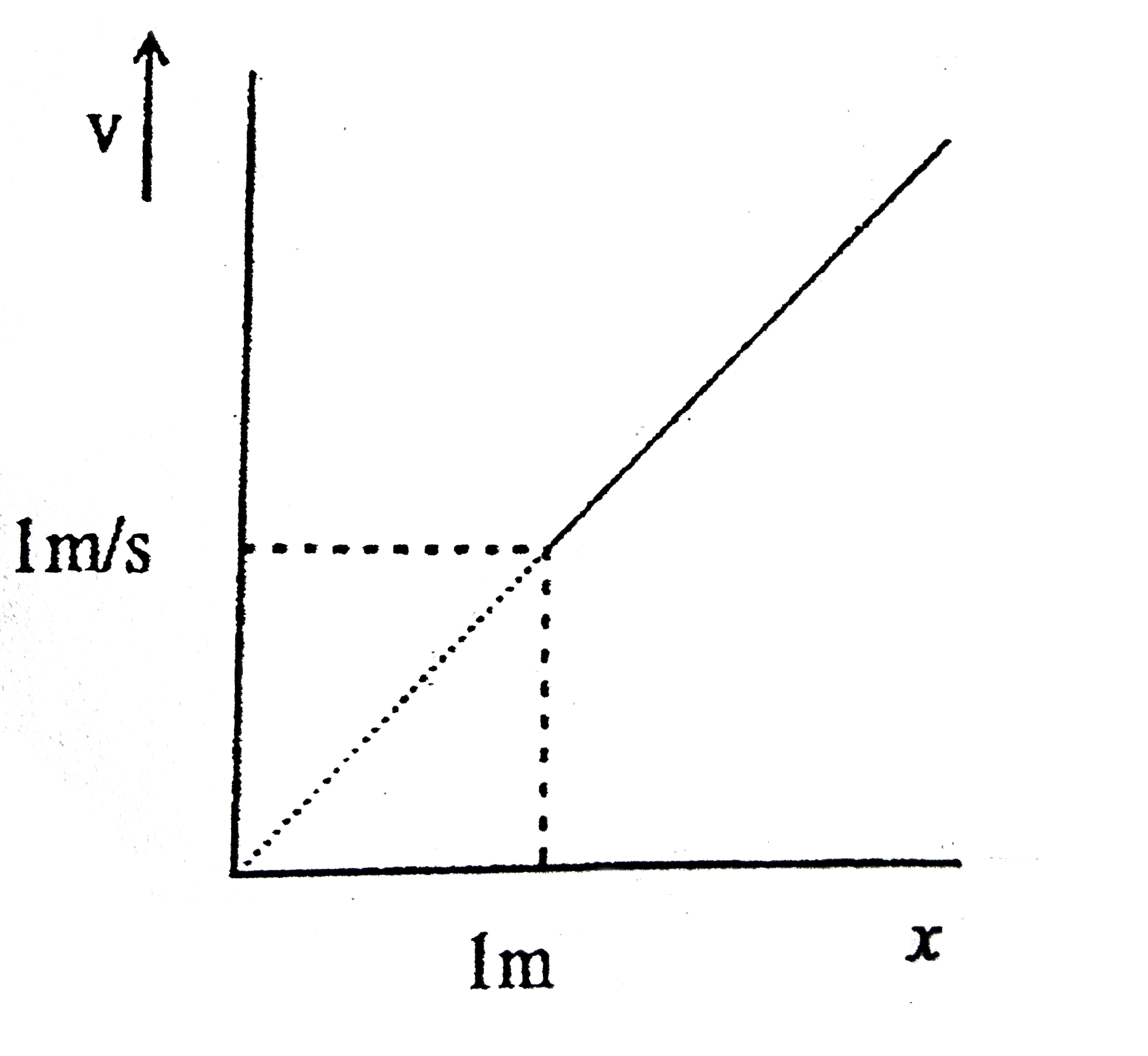 A
A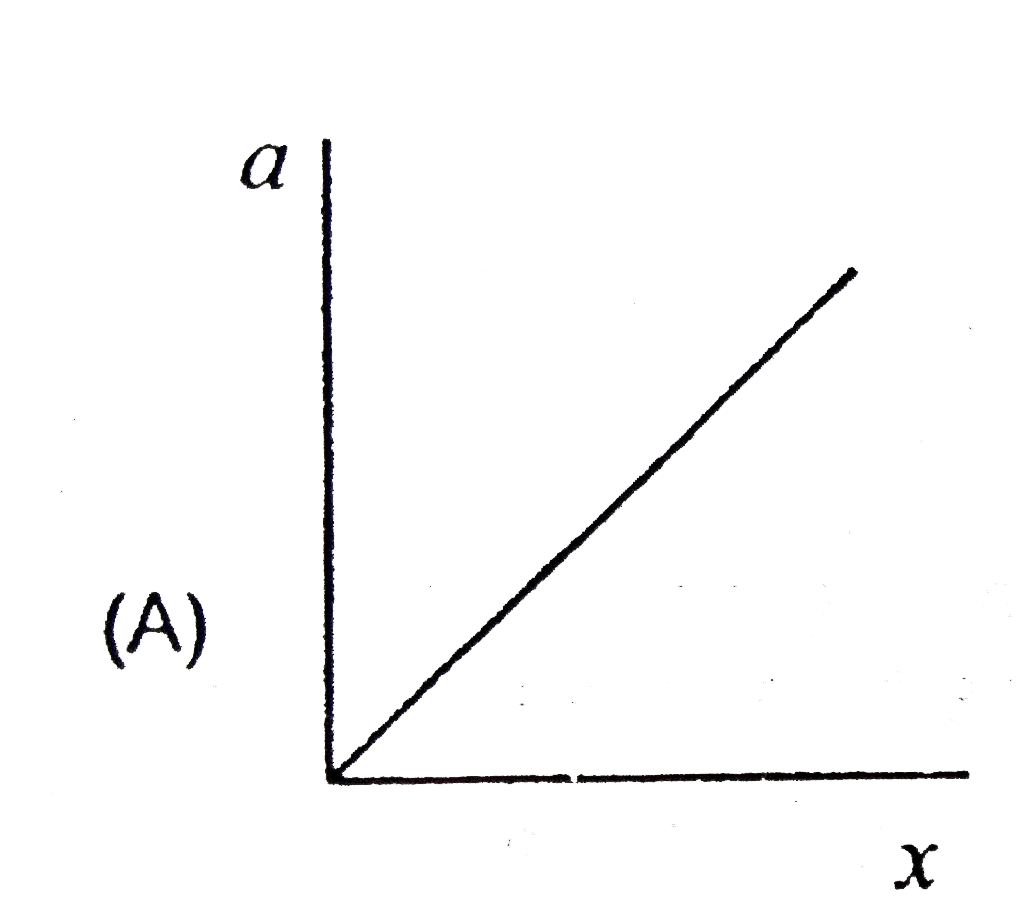 B
B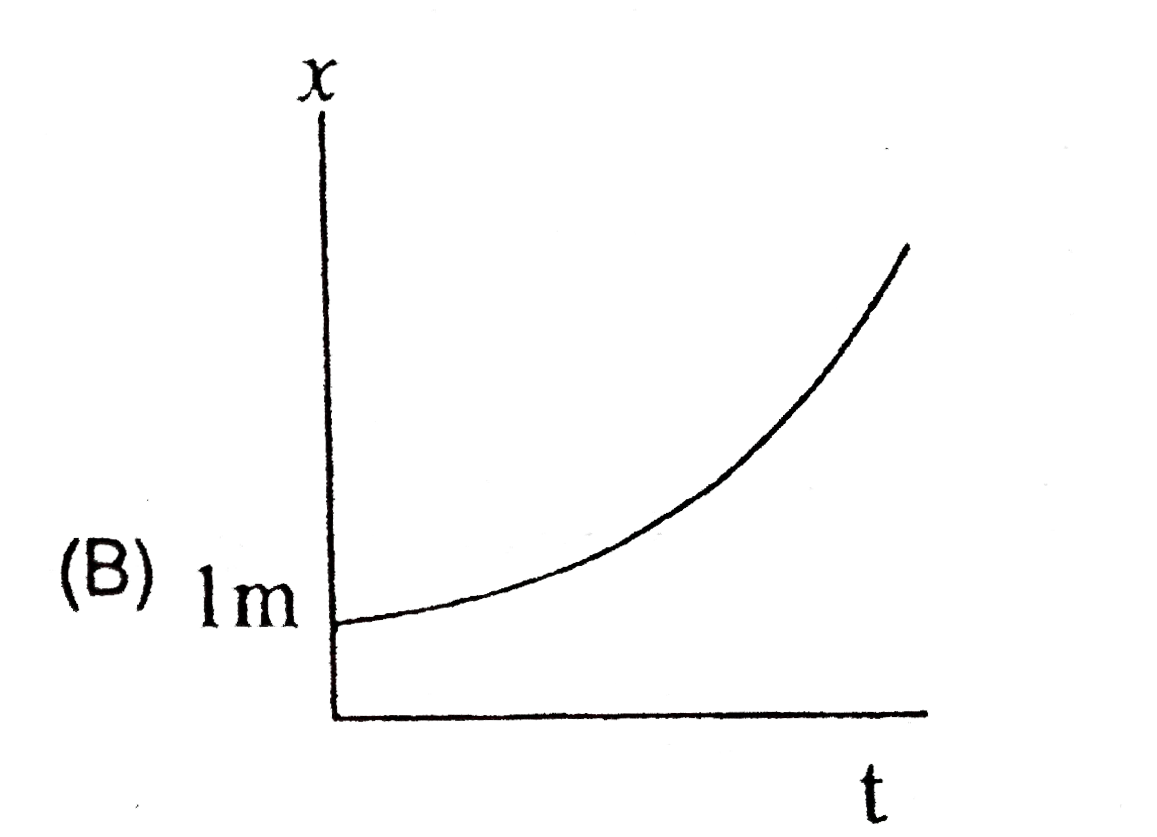 C
C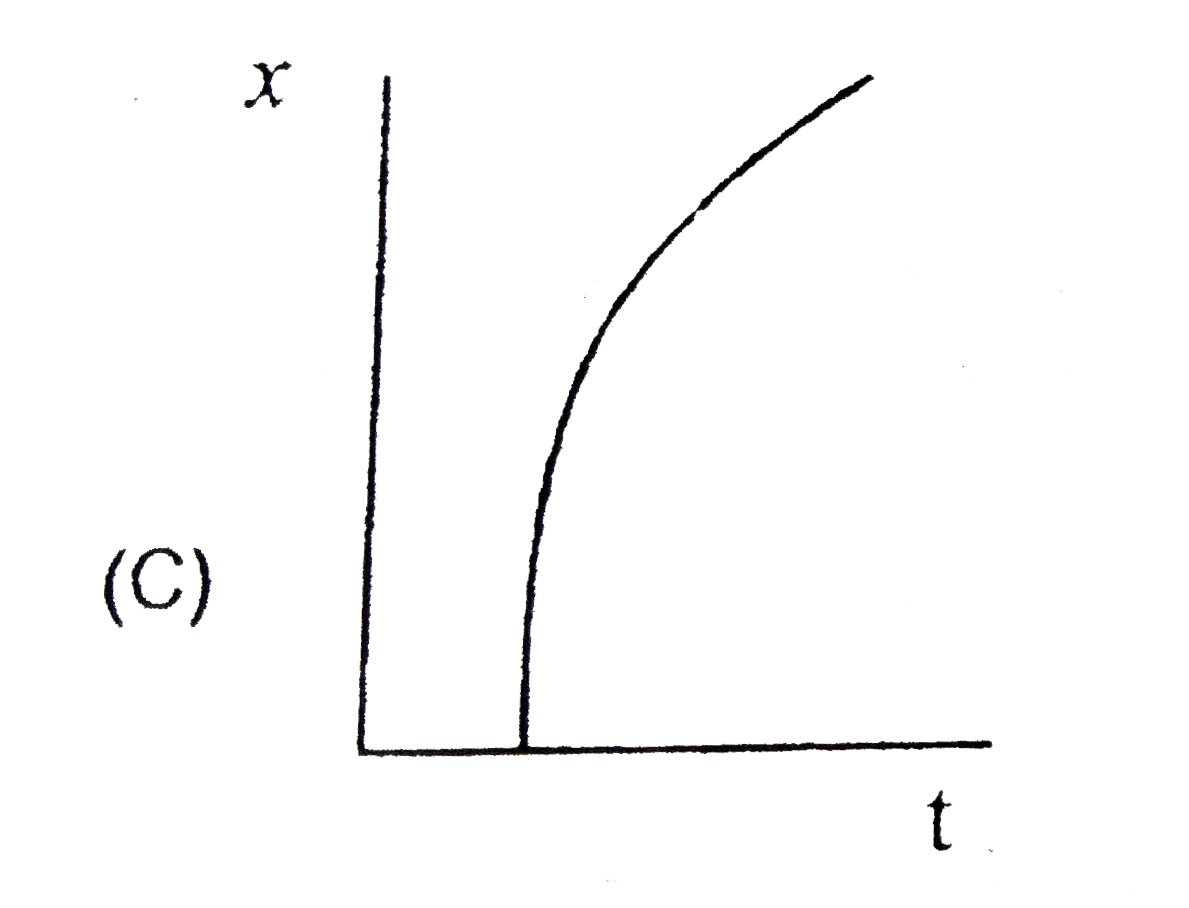 D
D
- Question 3 - Select One or More
A particle moves along a straight line its velocity dipends on time as v=4t−t2. Then for first 5s:
AAverage velcotu is 25/3ms−1BAverage speed is 10ms−1.CAverage velcotu is 5/3ms−1DAcceleration is 4ms−2 at t=o
A particle moves in a straight line with uniform acceleration. Its velocity at time t=0 is v1 and at time t=t is v2. The average velocity of the particle in this time interval is v1+v22 . Is this statement true or false?
View SolutionA particle is moving in a straight line according to graph given below : (a is acceleration, v is velocity, x is position, t is time) :
 View Solution
View SolutionA particle moves along a straight line its velocity dipends on time as v=4t−t2. Then for first 5s:
View SolutionA particle moves with a velocity v(t)=(12)kt2 along a straight line . Find the average speed of the particle in a time t.
View SolutionA particle moves in a straight line with an acceleration a ms−2 at time 't' seconds where a =−1t2. When t = 1 the particle has a velocity of 3ms−1 then the velocity when t = 4 is v4. Find the value of v.
View Solution

