Find the moment of inertia of a sphere about a tangent to the sphere, given the moment of inertia of the sphere about any of its diameters to be 2MR2/5. Where M is the mass of the sphere and R is the radius of the sphere.
Moment of inertia of sphere about any diameter = 25MR2
Applying theorem of parallel axes,
Moment of inertia of sphere about a tangent to the sphere = 25MR2+M(R)2
=75MR2.
Similar Questions
Find the moment of inertia of a sphere about a tangent to the sphere, while the mass of the sphere is M and the radius of the sphere is R.
View SolutionFind the moment of inertia of a sphere about a tangent to the sphere, while the mass of the sphere is M and the radius of the sphere is R.
View Solution
Knowledge Check
Find the moment of inertia of a sphere about a tangent to the sphere, while the mass of the sphere is M and the radius of the sphere is R.
A25MR2B65MR2C45MR2D75MR2If temperature increases, the moment of inertia of a solid sphere about its diameter
AincreasesBdecreasesCremains unchangedDbecomes negativeWith the increase in temperate, moment of inertia of a solid sphere about the diameter.
AdecreasesBincreasesCdoes not changeDnone of these
What is moment of inertia of a solid sphere about its diameter ?
View SolutionThe moment of inertia of a soild sphere about a tangent is 53mr2. What would be its moment of inertia about its diameter.
View SolutionThe moment of inertia of a sphere is 40 kg-m2 about its diametric axis. Determine the moment of inertia about any tangent.
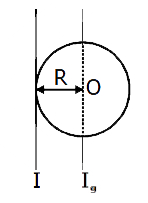
View SolutionFind the moment of inertia of solid sphere of mass M about a diameter as shown in Fig.
View SolutionThe moment of inertia of a sphere is 20 kg-m2 about the diameter. The moment of inertia about any tangent is
View Solution
