In exercise, let us take the position of mass when the spring is unstreched as x=0, and the direction from left to right as the positive direction of x-axis. Give x as a function of time t for the oscillating mass if at the moment we start the stopwatch (t=0), the mass is
a} at the mean position
b} at the maximum stretched position and
c} at the maximum compressed position In what way do these functions for SHM differ from each other, in frequency, in amplitude or the initial phase ?
Here, a=20cm,ω=√km=√12003=20s−1
a) As time is noted from the mean position, hence using x=asinωt, we have x=2sin20t.
b) At maximum stretched position, the body is the extreme right position, with an initial phase of π2 rad. Then
x=asin(ωt+π2)
=acosωt=2cos20t
c) At maximum compressed position, the body is at the extreme left position, with an initial phase of 3π2 rad.
Then x=asin(ωt+3π2)
=−acosωt
=−2cos20t
Similar Questions
In exercise 14.9 let us take the position of mass when the spring is unstretched as x = 0, and the direction from left to right as the positive direction of X-axis. Give x as a function of time t for the oscillating mass if at the moment we start the stopwatch (t = 0), the mass is
(a) at the mean position.
(b) at the maximum stretched position, and
( c ) at the maximum compressed position.
IN what way do these functions for SHM differ from each other, in frequency in amplitude or the initial phase ?
View SolutionIn example 34, let us take the position of the mass, when the spring is unstretched, as x=0, and the direction from left to right as the positive direction of X-axis. Give x as a function of time t for the oscillating mass, if at the moment we start the stop watch (t=0), the mass is (i) at the mean position (ii) at the maximum stretched position (iii) at the maximum compressed position.
In what do these different functions of SHM differ ? Frequency, amplitude or initial phase ?
View Solution
Knowledge Check
The inclination of the line x−y+3=0 with the positive direction of x-axis is
A45∘B135∘C−45∘D−135∘The inclination of the line x−y+3=0 with the positive direction of x-axis is :
A45∘B135∘C−45∘D−135∘This is the position graph of a mass on a spring what can you say about the velocity and the force at the instant indicated by the dashed line ? Positive direction is to right
 AVelocity is zero , force is to the rightBVelocity is positive , force is to the rightCVelocity is negative , force is to the rightDVelocity is zero , force is to the left
AVelocity is zero , force is to the rightBVelocity is positive , force is to the rightCVelocity is negative , force is to the rightDVelocity is zero , force is to the left
An impulsive force gives an initial velocity of- 1.0ms−1 to the mass in the unstretched spring position [sec Fig. 16.2]. What is the amplitude of motion ? Give x as a function of time t for the oscillating mass. Given m=3 kg and k=1200Nm−1.
View SolutionA particle A of mass 10/7kg is moving in the positive direction of x-axis. At initial position x=0, its velocity is 1ms−1, then its velocity at x=10m is (use the graph given)

View SolutionA car is moving on a straight road. The velocity of the car varies with time as shown in the figure. Initially (at t=0), the car was at x=0, where, x is the position of the car at any time 't'.
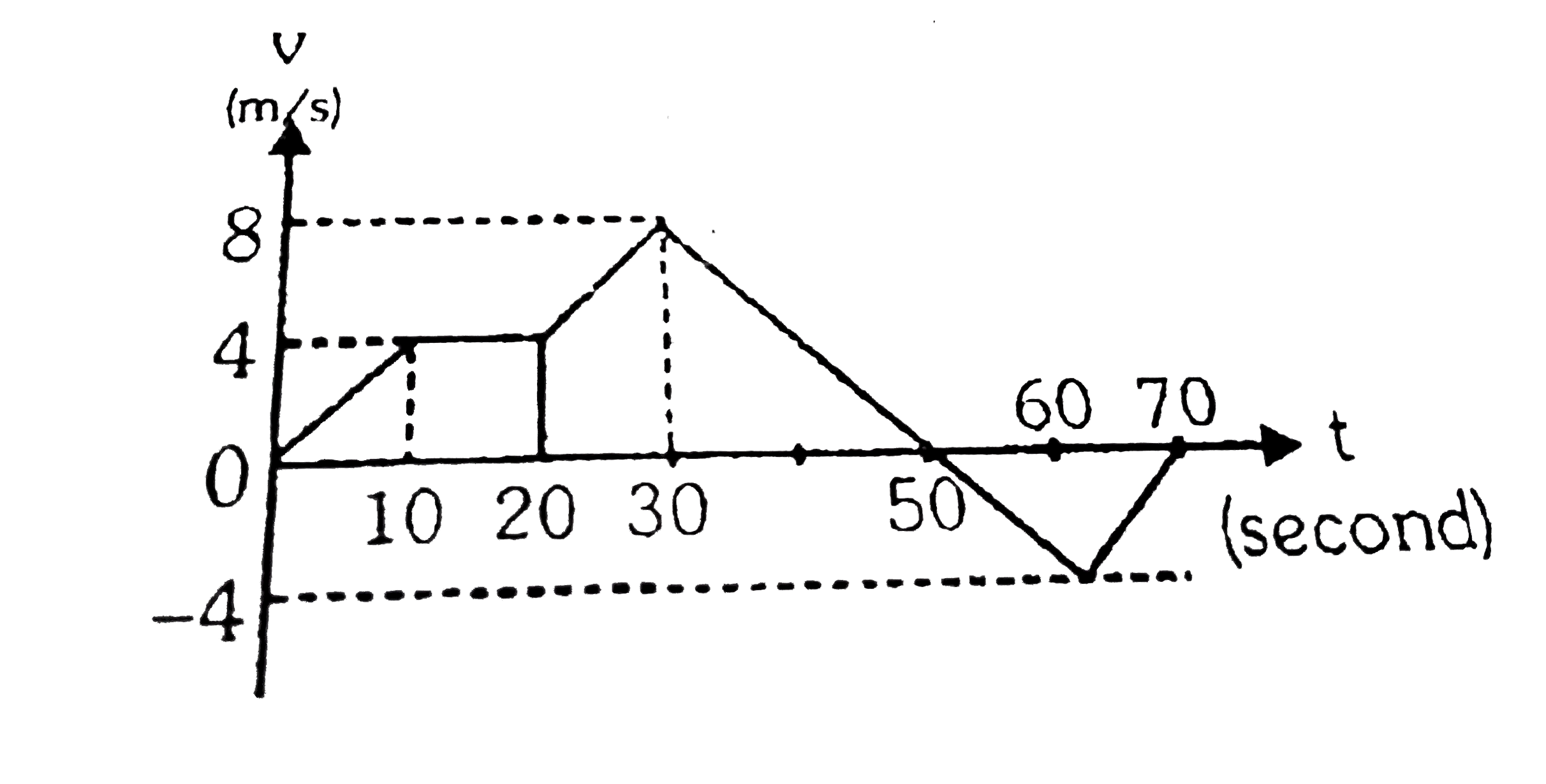
The maximum displacement from the starting position will be :-
View SolutionA car is moving on a straight road. The velocity of the car varies with time as shown in the figure. Initially (at t=0), the car was at x=0, where, x is the position of the car at any time 't'.

The maximum displacement from the starting position will be :-
View SolutionA particle A of mass 10/7kg is moving in the positive direction of x−aξs. At initial position x=0, its velocity is 1ms−1, then its velocity at x=10m is (use the graph given)

View Solution
