Evaluate : (xqxr)1qr×(xrxp)1rp×(xpxq)1pq
The correct Answer is:1
Step by step video, text & image solution for Evaluate : ((x^(q))/(x^(r )))^((1)/(qr))xx((x^(r ))/(x^(p)))^((1)/(rp))xx((x^(p))/(x^(q)))^((1)/(pq)) by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Solve : 1p+1q+1x=1x+p+q
View SolutionIf Pr is the coefficient of x® in the expansion of
(1+x)2(1+x2)2(1+x22)2(1+x23)2... prove that
Pr=22(2r−1)(Pr−1+Pr−2)andP4=1072315 .
View Solution∫pxp+2q−1−qxq−1x2p+2q+2xp+q+1dx is equal to
(1) −xpxp+q+1+C
(2) xqxp+q+1+C
(3) −xqxp+q+1+C
(4) xpxp+q+1+C
View Solution∫x132(1+x52)12dx=P(1+x52)72+Q(1+x52)52+R(1+x52)32+C then P, Q ,R are
View SolutionConsider
∫x3+3x2+2x+1√x2+x+1dx
=(ax2+bx+c)√x2+x+1+λ∫dx√x2+x+1
Now, match the following lists and then choose the correct code.
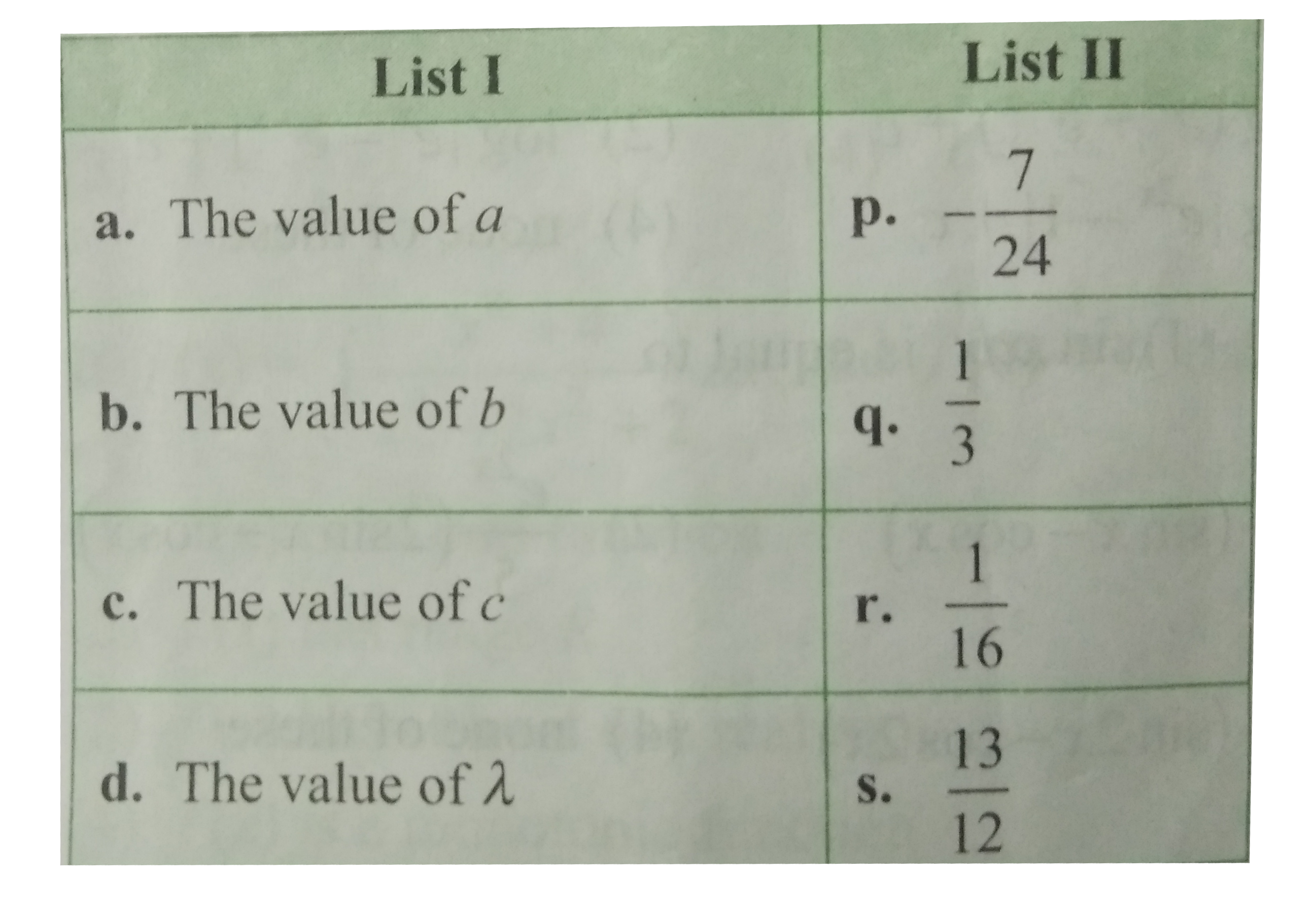
Codes:
abcd(1)qpsr(2)spqr(3)rqps(4)qspr
View Solution(3p2qr3)×(−4p3q2r2)×(7pq2r) is equal to
View SolutionThe roots of the equation (q−r)x2+(r−p)x+p−q=0 are (A) r−pq−r,1 (B) p−qq−r,1 (C) q−rp−q,1 (D) r−pp−q,1
View SolutionIf p>q>0 and pr<−1<qr, then find the value of tan−1(p−q1+pq)+tan−1(q−r1+qr)+tan−1(r−p1+rp)
View SolutionThe lines 1)(p−q)x+(q−r)y+(r−p)=0,2)(q−r)x+(r−p)y+(p−q)=0,3)(r−p)x+(p−q)y+(q−r)=0
View SolutionIf logxq−r=logyr−p=logzp−q prove that xq+r.yr+p.zp+q=xp.yq.zr
View Solution
