M and N are mid point on sides QR and PQ respectively of △PQR, right-anggled at Q. Prove that :
4PM2=4PQ2+QR2.
Step by step video & image solution for M and N are mid point on sides QR and PQ respectively of /_\ PQR, right-anggled at Q. Prove that : 4PM^(2)= 4PQ^(2)+QR^(2). by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PYTHAGORAS THEORAMBOOK - ICSECHAPTER - PYTHAGORAS THEORAMEXERCISE - QUESTIONS
9 Videos
- PYTHAGORAS THEORAMBOOK - ICSECHAPTER - PYTHAGORAS THEORAMEXERCISE - QUESTIONS
9 Videos
- MID-POINT THEOREMBOOK - ICSECHAPTER - MID-POINT THEOREMEXERCISE - 4 MARKS QUESTION
15 Videos
- PYTHAGORAS THEOREMBOOK - ICSECHAPTER - PYTHAGORAS THEOREMEXERCISE - 4 MARKS QUESTIONS
9 Videos
Similar Questions
M and N are mid- point on sides QR and PQ respectively of △PQR, right-anggled at Q. Prove that :
PM2+RN2=5MN2.
View SolutionM and N are point on sides QR and PQ respectively of △PQR, right-angled at Q. Prove that :
PM2+RN2=PR2+MN2
View SolutionM and N are the mid-points of the sides QR and PQ respectively of a ΔPQR, right-angled at Q. Prove that :
(i) PM2+RN2=5MN2
(ii) 4PM2=4PQ2+QR2
(iii) 4RN2=PQ2+4QR2
(iv) 4(PM2+RN2)=5PR2
View SolutionS and T are points on the sides PQ and PR, respectively of ΔPQR, such that PT = 2 cm, TR = 4 cm and ST is parallel to QR. Find the ratio of the areas of ΔPST and ΔPQR.
View SolutionABCD is a square. P, Q and Rare the points on AB, BC and CD respectively, such that AP = BQ = CR. Prove that:
PQ = QR
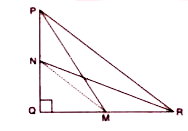
View SolutionIn the adjoining figure, QX and RX are the bisectors of the angles Q and R respectively of the triangle PQR.
If XS⊥QRandXT⊥PQ, prove that :
ΔXTQ≅ΔXSQ
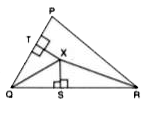
View SolutionIn figure A, B and C are points on OP, OQ and OR respectively such that AB || PQ and AC || PR. Show that BC || QR.
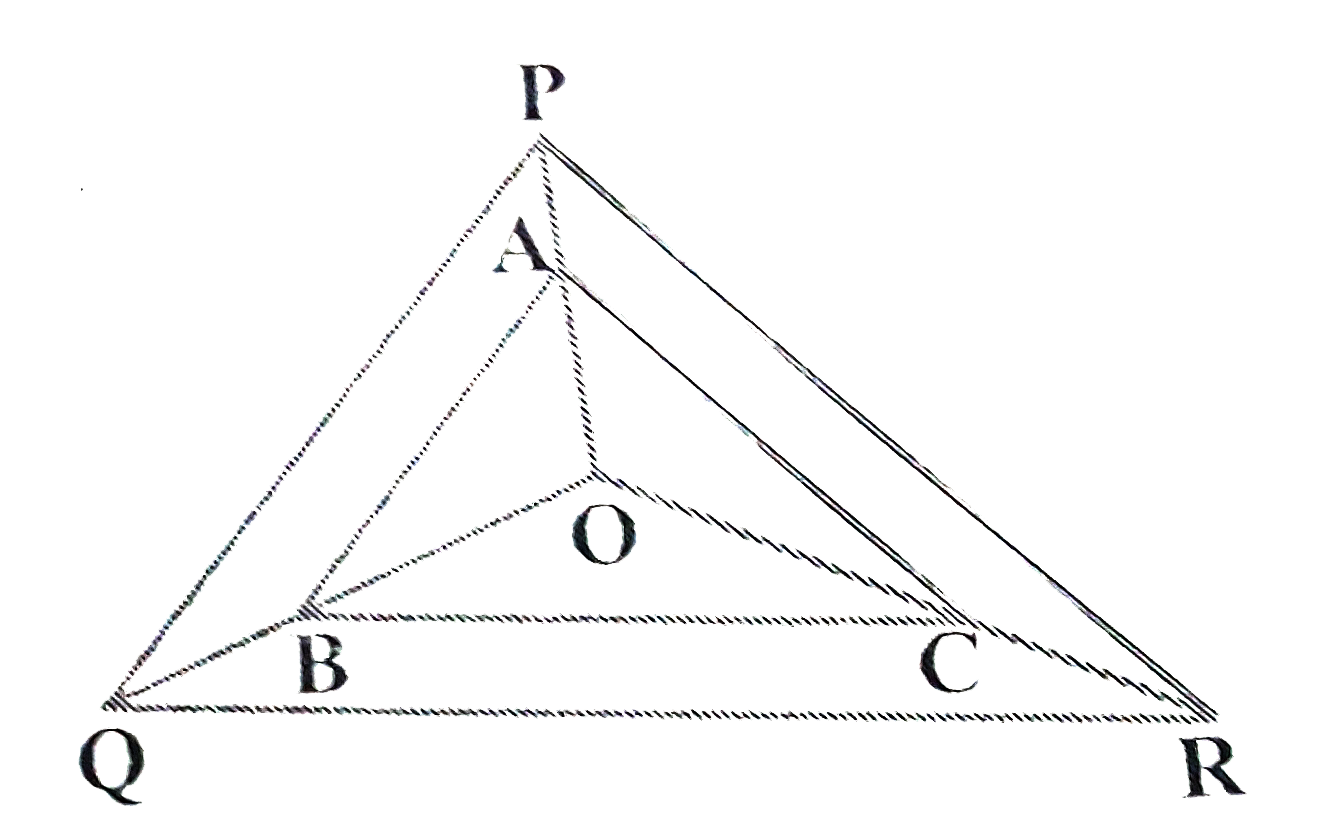
View SolutionIn the adjoining figure, ΔPQR is formed by the sides PQ, QR and RP which are drawn parallel to sides AB,BC and CA respectively of ΔABC. Prove that
PQ+QR+RP=2(AB+BC+CA).

View SolutionIn A PQR, if PQ=QR and L, M and N are the mid-points of the sides PQ, PR and RP respectively. Prove that LN=MN
View SolutionIn a triangle PQR, L and M are two points on the base QR, such that ∠LPQ=∠QRP and ∠RPM=∠RQP. Prove that:
PQ2=QR×QL
View Solution
