ABCD is a square. P, Q and Rare the points on AB, BC and CD respectively, such that AP = BQ = CR. Prove that:
PQ = QR
To prove that PQ=QR in the square ABCD with points P,Q,R on sides AB,BC, and CD respectively, where AP=BQ=CR, we can follow these steps:
1. Label the Square and Points:
- Let the square ABCD have vertices A(0,0), B(1,0), C(1,1), and D(0,1).
- Let AP=BQ=CR=x (where x is the length of the segments).
2. Determine Coordinates of Points:
- Since P is on AB, its coordinates are P(x,0).
- Since Q is on BC, its coordinates are Q(1,x).
- Since R is on CD, its coordinates are R(1−x,1).
3. Calculate Length PQ:
- The distance PQ can be calculated using the distance formula:
PQ=√(x−1)2+(0−x)2
Simplifying this:
PQ=√(x−1)2+x2=√x2−2x+1+x2=√2x2−2x+1
4. Calculate Length QR:
- The distance QR can also be calculated:
QR=√(1−(1−x))2+(x−1)2
Simplifying this:
QR=√(x)2+(x−1)2=√x2+(x2−2x+1)=√2x2−2x+1
5. Conclude that PQ=QR:
- From the calculations, we see that:
PQ=√2x2−2x+1=QR
- Therefore, we conclude that PQ=QR.
- Let the square ABCD have vertices A(0,0), B(1,0), C(1,1), and D(0,1).
- Let AP=BQ=CR=x (where x is the length of the segments).
- Since P is on AB, its coordinates are P(x,0).
- Since Q is on BC, its coordinates are Q(1,x).
- Since R is on CD, its coordinates are R(1−x,1).
- The distance PQ can be calculated using the distance formula:
PQ=√(x−1)2+(0−x)2
Simplifying this:
PQ=√(x−1)2+x2=√x2−2x+1+x2=√2x2−2x+1
- The distance QR can also be calculated:
QR=√(1−(1−x))2+(x−1)2
Simplifying this:
QR=√(x)2+(x−1)2=√x2+(x2−2x+1)=√2x2−2x+1
- From the calculations, we see that:
PQ=√2x2−2x+1=QR
- Therefore, we conclude that PQ=QR.
Topper's Solved these Questions
- CHAPTERWISE REVISION (STAGE 3) BOOK - ICSECHAPTER - CHAPTERWISE REVISION (STAGE 3) EXERCISE - ISOSCELES TRIANGLES
2 Videos
- CHAPTERWISE REVISION (STAGE 3) BOOK - ICSECHAPTER - CHAPTERWISE REVISION (STAGE 3) EXERCISE - INEQUALITIES
7 Videos
- CHAPTERWISE REVISION (STAGE 3) BOOK - ICSECHAPTER - CHAPTERWISE REVISION (STAGE 3) EXERCISE - LOGARITHM
9 Videos
- CHAPTERWISE REVISION (STAGE 1) BOOK - ICSECHAPTER - CHAPTERWISE REVISION (STAGE 1) EXERCISE - Graphical solution
10 Videos
- CIRCLEBOOK - ICSECHAPTER - CIRCLEEXERCISE - EXERCISE 17(D)
12 Videos
Similar Questions
ABCD is a square. P, Q and Rare the points on AB, BC and CD respectively, such that AP = BQ = CR. Prove that:
PB = QC
View SolutionABCD is a square. P, Q and Rare the points on AB, BC and CD respectively, such that AP = BQ = CR. Prove that:
If angle PQR is a rt. Angle find angle PRQ
View SolutionABCD is a square, X and Y are points on sides AD and BC respectively such that AY=BX. Prove that BY=AX and ∠BAY=∠ABX.
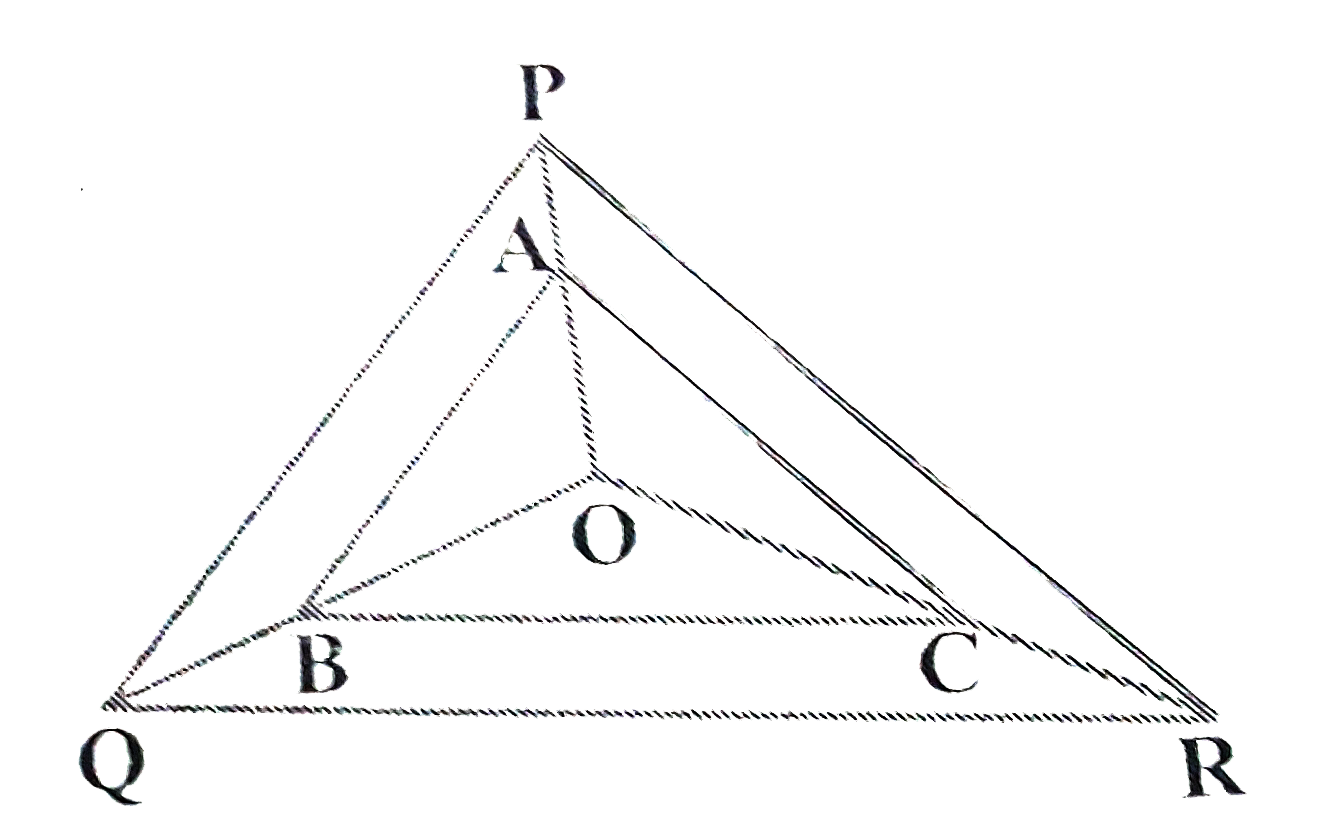
View SolutionIn figure A, B and C are points on OP, OQ and OR respectively such that AB || PQ and AC || PR. Show that BC || QR.
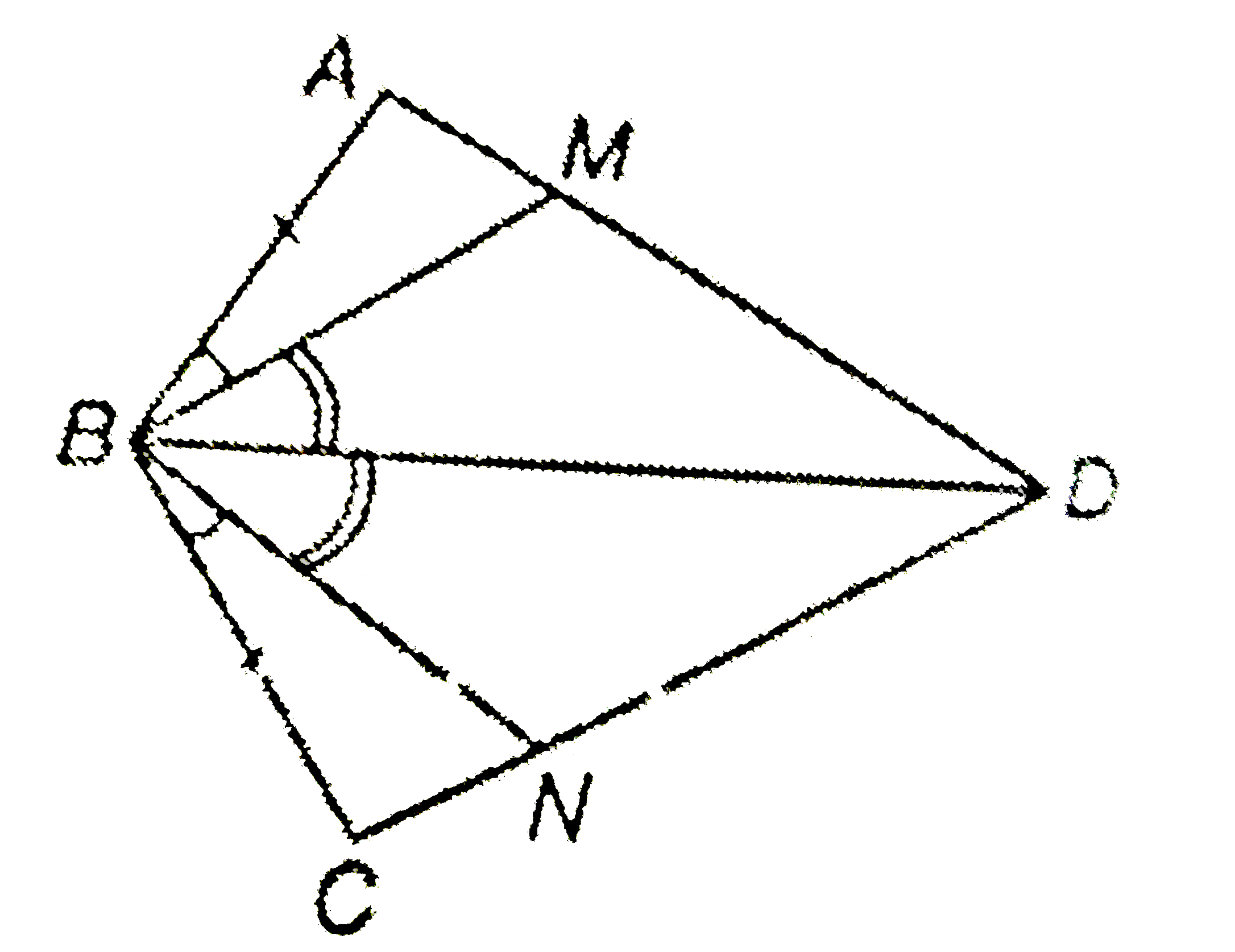
View SolutionIn the adjoining figure, ABCD is a quadrilateral. M and N are the points on AD and CD respectively such that AB=BC,∠ABM=∠CBN and ∠MBD=∠NBD. Prove that BM=BN.
View SolutionABCD is a rectangle measuring 60 cm by 50 cm P,Q,and R are the points on AB, AD , and BC, respectively, such that AP=40cm,AQ=20cm,andBR=40cm. Find the area of the polygon QPBCD.
View SolutionIn the given figure A, B and C are points on OP, OQ and OR respectively such that AB||PQ and BC||QR. Show that AC||PR.

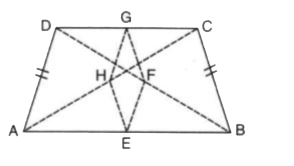
View SolutionABCD is a quadrilateral in which AD = BC. E, F, G and H are the mid-points of AB, BD, CD and AC respectively. Prove that EFGH is a rhombus.
View SolutionABCD is a parallelogram. Two points P and Q are taken on sides AD and BC respectively such that AP 13ADandCQ=13BC. Prove that □AQCP is a parallelogram.
View SolutionIn trapezium ABCD, AB is parallel to DC. P and Q are the mid-points of AD and BC respectively. BP produced meets CD produced at point E. Prove that :
PQ is parallel to AB.
View Solution
