Solve the following inequation and write the solution set :
Represent the solution on a real number line.
To solve the given inequation 13x−5<15x+4<7x+12, we will break it into two parts and solve each part step by step.
Step 1: Solve the first part of the inequation
We start with the first part:
13x−5<15x+4
Rearranging the inequality:
1. Subtract 13x from both sides:
−5<2x+4
2. Subtract 4 from both sides:
−9<2x
3. Divide both sides by 2:
−92<x
or
x>−92
Step 2: Solve the second part of the inequation
Now we solve the second part:
15x+4<7x+12
Rearranging the inequality:
1. Subtract 7x from both sides:
8x+4<12
2. Subtract 4 from both sides:
8x<8
3. Divide both sides by 8:
x<1
Step 3: Combine the results
We have two inequalities:
1. x>−92
2. x<1
Combining these, we get:
−92<x<1
Step 4: Write the solution set
The solution set in interval notation is:
(−92,1)
This means x can take any value between −92 and 1, not including the endpoints.
Step 5: Represent the solution on a real number line
To represent this on a number line:
- Draw a horizontal line.
- Mark the points −92 (which is -4.5) and 1.
- Use open circles at both points to indicate that these endpoints are not included in the solution.
- Shade the region between −4.5 and 1.
Final Answer
The solution set is:
(−92,1)
And the representation on the number line shows the interval between −4.5 and 1 with open circles.
---
We start with the first part:
13x−5<15x+4
1. Subtract 13x from both sides:
−5<2x+4
2. Subtract 4 from both sides:
−9<2x
3. Divide both sides by 2:
−92<x
or
x>−92
Now we solve the second part:
15x+4<7x+12
1. Subtract 7x from both sides:
8x+4<12
2. Subtract 4 from both sides:
8x<8
3. Divide both sides by 8:
x<1
We have two inequalities:
1. x>−92
2. x<1
−92<x<1
The solution set in interval notation is:
(−92,1)
This means x can take any value between −92 and 1, not including the endpoints.
To represent this on a number line:
- Draw a horizontal line.
- Mark the points −92 (which is -4.5) and 1.
- Use open circles at both points to indicate that these endpoints are not included in the solution.
- Shade the region between −4.5 and 1.
The solution set is:
(−92,1)
And the representation on the number line shows the interval between −4.5 and 1 with open circles.
Topper's Solved these Questions
- LINEAR INEQUATIONSBook:ICSEChapter:LINEAR INEQUATIONSExercise:QUESTIONS
Explore 22 Videos
- LINEAR INEQUATIONSBook:ICSEChapter:LINEAR INEQUATIONSExercise:Multiple Choice Questions
Explore 47 Videos
- LINEAR INEQUATIONSBook:ICSEChapter:LINEAR INEQUATIONSExercise:Competency Based Questions
Explore 15 Videos
- HEIGHTS AND DISTANCES Book:ICSEChapter:HEIGHTS AND DISTANCES Exercise:Exercise 22 C
Explore 28 Videos
- LOCI (LOCUS AND ITS CONSTRUCTIONS)Book:ICSEChapter:LOCI (LOCUS AND ITS CONSTRUCTIONS)Exercise:EXERCISE 16(B)
Explore 49 Videos
Similar Questions
Knowledge Check
If 2x−3<x+1<4x+7,x∈R then the solution set of x on the number line is:
A B
B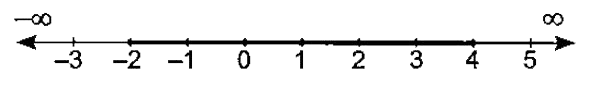 C
C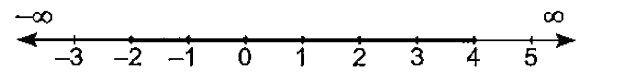 D
D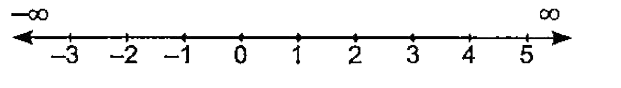
If −5<2x−3≤x+2,x∈R, then the solution set of x on the number line is
A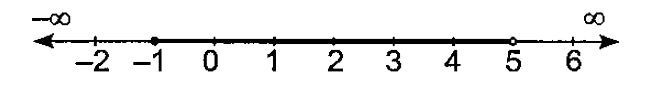 B
B C
C D
D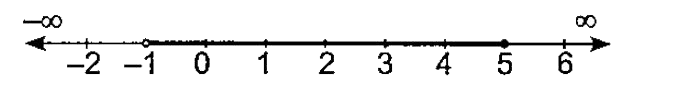
Solve the following inequation and graph the solution set on the number line :
2x−3<x+2≤3x+5,x∈R.
View SolutionSolve the inequation 3x−7<5x−3 If x ∈ R and represent the solution on the number line
View SolutionSolve the inequation 3x−2<5 If (i) x∈ N (ii) x ∈ R and represent the solution on the number line
View SolutionSolve the inequation 5x−5≤−5 If (i) x ∈ z (ii) xin R and represent the solution on the number line
View SolutionList the solution set of 50 - 3 (2x - 5) < 25, given that x∈W. Also, represent the solution set obtained on a number line.
View SolutionSolve the inequation 12x<50 If (i) x ∈ Z (ii) x ∈ R and represent the solution on the number line
View Solution