Solve the following ineqaution and write the solution set :
13x−5<15x+4<7x+12,x∈R
Represent the solution on a real number line
To solve the inequality 13x−5<15x+4<7x+12, we will break it down into two separate inequalities and solve each one step by step.
Step 1: Solve the first inequality
The first part of the compound inequality is:
13x−5<15x+4
Rearranging the inequality:
1. Subtract 13x from both sides:
−5<15x−13x+4
This simplifies to:
−5<2x+4
2. Next, subtract 4 from both sides:
−5−4<2x
This simplifies to:
−9<2x
3. Finally, divide both sides by 2:
−92<x
or
x>−4.5
Step 2: Solve the second inequality
The second part of the compound inequality is:
15x+4<7x+12
Rearranging the inequality:
1. Subtract 7x from both sides:
15x−7x+4<12
This simplifies to:
8x+4<12
2. Next, subtract 4 from both sides:
8x<12−4
This simplifies to:
8x<8
3. Finally, divide both sides by 8:
x<1
Step 3: Combine the results
From the two inequalities, we have:
1. x>−4.5
2. x<1
Combining these gives us:
−4.5<x<1
Step 4: Write the solution set
The solution set in set-builder notation is:
{x∈R∣−4.5<x<1}
Step 5: Represent the solution on a real number line
To represent this on a number line:
- Draw a horizontal line.
- Mark the points −4.5 and 1.
- Use open circles at −4.5 and 1 to indicate that these points are not included in the solution.
- Shade the region between −4.5 and 1.
The first part of the compound inequality is:
13x−5<15x+4
1. Subtract 13x from both sides:
−5<15x−13x+4
This simplifies to:
−5<2x+4
−5−4<2x
This simplifies to:
−9<2x
−92<x
or
x>−4.5
The second part of the compound inequality is:
15x+4<7x+12
1. Subtract 7x from both sides:
15x−7x+4<12
This simplifies to:
8x+4<12
8x<12−4
This simplifies to:
8x<8
x<1
From the two inequalities, we have:
1. x>−4.5
2. x<1
−4.5<x<1
The solution set in set-builder notation is:
{x∈R∣−4.5<x<1}
To represent this on a number line:
- Draw a horizontal line.
- Mark the points −4.5 and 1.
- Use open circles at −4.5 and 1 to indicate that these points are not included in the solution.
- Shade the region between −4.5 and 1.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
The solution set of 6≤−3(2x−4)<12,x∈R is
A(0,1]B[1,0)C(0,1)D[0,1] - Question 2 - Select One
If −5<2x−3≤x+2,x∈R, then the solution set of x on the number line is
A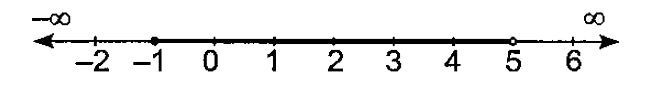 B
B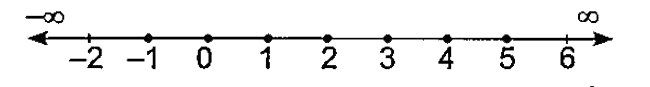 C
C D
D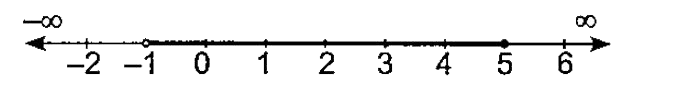
- Question 3 - Select One
If 2x−3<x+1<4x+7,x∈R then the solution set of x on the number line is:
A B
B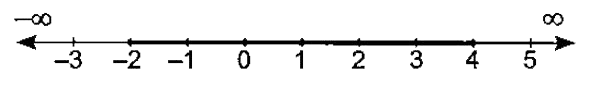 C
C D
D
Solve the inequation 2x+5>−5x+12 If x ∈ R and represent the solution on the number line
View SolutionSolve the inequation 3x−7<5x−3 If x ∈ R and represent the solution on the number line
View SolutionFind the solution set of 2x−7<8,x∈N. Represent the solution set on the number line.
View SolutionSolve the inequation 5x−2≥3x−1 If x ∈ R and represent the solution on the number line
View SolutionSolve the inequation 12x<50 If (i) x ∈ Z (ii) x ∈ R and represent the solution on the number line
View Solution

