If a−2b−3c+4da+2b−3c−4d=a−2b+3c−4da+2b+3c+4d,showtˆ:2ad = 3bc.
To solve the equation
a−2b−3c+4da+2b−3c−4d=a−2b+3c−4da+2b+3c+4d,
we will apply the Componendo and Dividendo rule, which states that if
pq=rs,
then
p+qp−q=r+sr−s.
Step 1: Apply Componendo and Dividendo
Using the Componendo and Dividendo rule, we rewrite the equation as follows:
(a−2b−3c+4d)+(a+2b−3c−4d)(a−2b−3c+4d)−(a+2b−3c−4d)=(a−2b+3c−4d)+(a+2b+3c+4d)(a−2b+3c−4d)−(a+2b+3c+4d).
Step 2: Simplify the Numerators and Denominators
Now, let’s simplify both sides:
Left Side:
- Numerator:
(a−2b−3c+4d)+(a+2b−3c−4d)=2a−6c
- Denominator:
(a−2b−3c+4d)−(a+2b−3c−4d)=−4b+8d=−4(b−2d)
So, the left side becomes:
2a−6c−4(b−2d).
Right Side:
- Numerator:
(a−2b+3c−4d)+(a+2b+3c+4d)=2a+6c
- Denominator:
(a−2b+3c−4d)−(a+2b+3c+4d)=−4b−8d=−4(b+2d)
So, the right side becomes:
2a+6c−4(b+2d).
Step 3: Set the Two Sides Equal
Now we have:
2a−6c−4(b−2d)=2a+6c−4(b+2d).
Step 4: Cross Multiply
Cross multiplying gives:
(2a−6c)(−4(b+2d))=(2a+6c)(−4(b−2d)).
Step 5: Expand Both Sides
Expanding both sides:
−8a(b+2d)+24c(b+2d)=−8a(b−2d)−24c(b−2d).
Step 6: Rearranging Terms
Rearranging terms leads to:
−8ab−16ad+24bc+48cd=−8ab+16ad−24bc+48cd.
Step 7: Cancel Common Terms
Cancelling common terms results in:
−16ad+24bc=16ad−24bc.
Step 8: Combine Like Terms
Combining like terms gives:
−32ad=−48bc.
Step 9: Simplify
Dividing through by -16 yields:
2ad=3bc.
This is the required result.
- Numerator:
(a−2b−3c+4d)+(a+2b−3c−4d)=2a−6c
- Denominator:
(a−2b−3c+4d)−(a+2b−3c−4d)=−4b+8d=−4(b−2d)
2a−6c−4(b−2d).
- Numerator:
(a−2b+3c−4d)+(a+2b+3c+4d)=2a+6c
- Denominator:
(a−2b+3c−4d)−(a+2b+3c+4d)=−4b−8d=−4(b+2d)
2a+6c−4(b+2d).
2a−6c−4(b−2d)=2a+6c−4(b+2d).
(2a−6c)(−4(b+2d))=(2a+6c)(−4(b−2d)).
−8a(b+2d)+24c(b+2d)=−8a(b−2d)−24c(b−2d).
−8ab−16ad+24bc+48cd=−8ab+16ad−24bc+48cd.
−16ad+24bc=16ad−24bc.
−32ad=−48bc.
2ad=3bc.
Topper's Solved these Questions
- RATIO AND PROPORTION (INCLUDING PROPERTIES AND USES)BOOK - ICSECHAPTER - RATIO AND PROPORTION (INCLUDING PROPERTIES AND USES)EXERCISE - Exercise 7(D)
27 Videos
- RATIO AND PROPORTION (INCLUDING PROPERTIES AND USES)BOOK - ICSECHAPTER - RATIO AND PROPORTION (INCLUDING PROPERTIES AND USES)EXERCISE - QUESTIONS
30 Videos
- RATIO AND PROPORTION (INCLUDING PROPERTIES AND USES)BOOK - ICSECHAPTER - RATIO AND PROPORTION (INCLUDING PROPERTIES AND USES)EXERCISE - Exercise 7(B)
17 Videos
- RATIO AND PROPORTIONBOOK - ICSECHAPTER - RATIO AND PROPORTIONEXERCISE - MULTIPLE CHOICE QUESTION
61 Videos
- REFLECTION BOOK - ICSECHAPTER - REFLECTION EXERCISE - QUESTION
1 Videos
Similar Questions
If a:b=c:d, prove that :
(6a+7b)(3c−4d)=(6c+7d)(3a−4b).
View SolutionIf (a−2b−3c+4d)(a+2b+3c+4d)=(a+2b−3c−4d)(a−2b+3c−4d) then 2ad=
(a) 3bc (b) bc (c) 5bc (d) 2bc
View Solutionif a:b=e:d, show that : 3a+2b:3a−2b=3c+2d:3c−2d.
View Solution(i) If a, b, c are in continued proportion, show that : a2+b2b(a+c)=b(a+c)b2+c2.
(ii) If a, b, c are in continued proportion and a(b−c)=2b, prove that :
a−c=2(a+b)a.
(iii) If ab=cd show that :
a3c+ac3b3d+bd3=(a+c)4(b+d)4.
View SolutionMatch List I ( Sub-structures) with List II (Functions and select the CORRECT answer using the codes given below the lists :
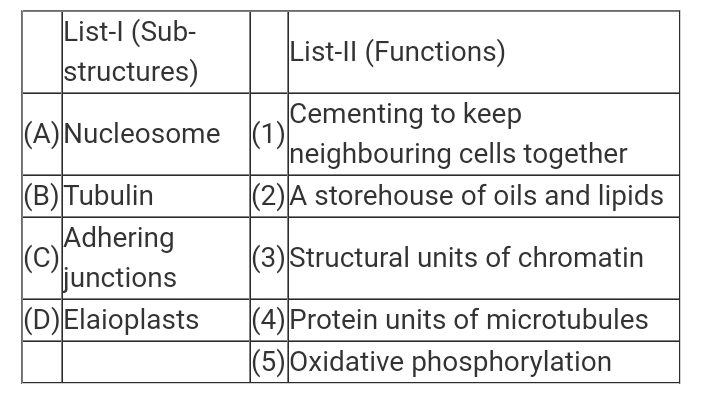
(a) A - 2, B - 5 , C - 4, D - 3
(b) A - 3, B - 4 , C - 1, D - 2
(c) A - 3, B - 5 , C - 4, D - 2
(d) A - 2, B - 4 , C - 1, D - 3
View SolutionIf a, b, c, d satisfy the equation a+7b+3c+5d=0, 8a+4b+6c+2d=−16, 2a+6b+4c+8d=16 and 5a+3b+7c+d=−16 then the value of (a+d)(b+c) =______
View SolutionIf 7a+8b7c+8d=7a−8b7c−8d, prove that :
(i)a:b=c:d
(ii) 4a−5b4c−5d=3a+4b3c+4d
View SolutionIf a:b=2:3,b:c=4:5andc:d=6:7, find : a:b:c:d.
View SolutionIf real numbers a, b, c, d, e satisfy a+1=b + 2 = c+ 3 = d + 4 = e + 5 = a + b + c + d + e + 3 then find the value of a2+b2+c2+d2+e2
View SolutionIf a:b=3:4, then 4a:3b=
(a)4:3
(b) 3:4
(c)1:1
(d) None of these
View Solution
