Match the equations A, B, C, D and E with the lines , whose graphs are roughly drawn in the given diagram.
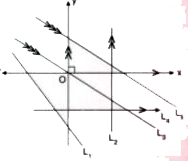
,
The correct Answer is:
Step by step video, text & image solution for Match the equations A, B, C, D and E with the lines L_1, L_2, L_3, L_4 and L_5, whose graphs are roughly drawn in the given diagram. A -= 2x + y = 0, " " B -= 2x + y = 20 C -= x = 8, D = y = -12 " " E = 2x + 3y + 12 = 0 by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
- BOOK - ICSECHAPTER - EQUATION OF A LINEEXERCISE - EXERCISE 14(A)18videos
EQUATION OF A LINE
- BOOK - ICSECHAPTER - EQUATION OF A LINEEXERCISE - EXERCISE 14(B)39videos
EQUATION OF A LINE
- BOOK - ICSECHAPTER - CYLINDER, CONE AND SPHERE EXERCISE - EXERCISE 20 (G)23videos
CYLINDER, CONE AND SPHERE
- BOOK - ICSECHAPTER - FACTORISATIONEXERCISE - M.C.Q(Competency Based Questions )15videos
FACTORISATION
Similar Questions
Match the equations A, B, C and D with the lines L1,L2,L3andL4, whose graphs are roughly drawn in the given diagram.
A≡y=2x, B≡y−2x+2=0,
C≡3x+2y=6, D=y=2
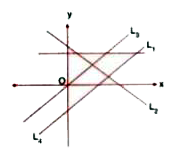 View Solution
View SolutionThe equation of line l1 is y=2x+3, and the equation of line l2 is y=2x-5.
View SolutionLet two parallel lines L1 and L2 with positive slope are tangent to the circle C1 : x2+y2−2x16y+64=0. If L1 is also tangent to the circle C2 : x2+y2−2x+2y−2=0 and the equation of L2 is a√ax−by+c−a√a=0 where a,b,c in N. then find the value of a+b+c7
View SolutionConsider the line L 1 : x 1 y 2 z 1 312 +++ ==, L2 : x2y2z3 123
View SolutionConsider the line L 1 : x 1 y 2 z 1 312 +++ ==, L2 : x2y2z3 123
View SolutionA line L passing through (1, 1) intersect lines L1:12x+5y=13 and L2:12x+5y=65 at A and B respectively. If AB=5 , then line L can be
View SolutionGiven ABCD is a parallelogram with vertices as A(1,2),B(3,4) and C(2,3). Then the equation of line AD is (A) x+y+1=0 (B) x−y+1=0 (C) −x+y+1=0 (D) x+y−1=0
View SolutionLet (2,3) be the focus of a parabola and x+y=0 and x−y=0 be its two tangents. Then equation of its directrix will be (a) 2x−3y=0 (b) 3x+4y=0 (c) x+y=5 (d) 12x−5y+1=0
View SolutionLet L be a normal to the parabola y2=4x. If L passes through the point (9, 6), then L is given by (a) y−x+3=0 (b) y+3x−33=0 (c) y+x−15=0 (d) y−2x+12=0
View SolutionIf the lines L1:2x−3y−6=0,L2:x+y−4=0 and L3:x+2 and tanA,tanB and tanC are the roots of the equation ax3+bx2+cx−15=0 then a=3 (b) b=−15 c=28 (d) a+b+c=15
View Solution

