The line through P(5, 3) intersects y-axis at Q.

Write the equation of the line.
The correct Answer is:y=x−2
Step by step video, text & image solution for The line through P(5, 3) intersects y-axis at Q. Write the equation of the line. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
- BOOK - ICSECHAPTER - EQUATION OF A LINEEXERCISE - EXERCISE 14(D)41videos
EQUATION OF A LINE
- BOOK - ICSECHAPTER - EQUATION OF A LINEEXERCISE - EXERCISE 14(E)68videos
EQUATION OF A LINE
- BOOK - ICSECHAPTER - EQUATION OF A LINEEXERCISE - EXERCISE 14(B)39videos
EQUATION OF A LINE
- BOOK - ICSECHAPTER - CYLINDER, CONE AND SPHERE EXERCISE - EXERCISE 20 (G)23videos
CYLINDER, CONE AND SPHERE
- BOOK - ICSECHAPTER - FACTORISATIONEXERCISE - M.C.Q(Competency Based Questions )15videos
FACTORISATION
Similar Questions
In the given figure, The line through P (5, 3) intersects Y-axis at Q.
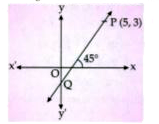
Write the slope of the line.View SolutionThe line through P(5, 3) intersects y-axis at Q.
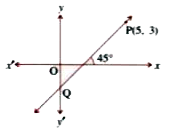
Write the slope of the line.View SolutionThe line through P(5, 3) intersects y-axis at Q.
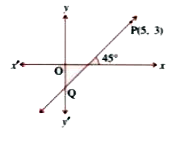
Find the co-ordinates of Q.View SolutionIn the given figure , The line through P (5, 3) intersects Y-axis at Q.
Find the coordinates of Q.
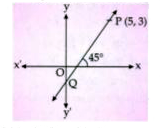
Find the coordinates of Q.View SolutionIn the figure, given, ABC is a triangle and BC is parallel to the y-axis. AB and AC intersect the y-axis at P and Q respectively.
Find the equation of the line AC.View SolutionA straight line L through the point (3,-2) is inclined at an angle 60∘ to the line √3x+y=1 If L also intersects the x-axis then the equation of L is
View SolutionA straight line L through the point (3,-2) is inclined at an angle 60∘ to the line √3x+y=1. If L also intersects the x-axis then find the equation of L .
View SolutionThe line through (4,3)and(−6,0) intersects the line 5x+y=0. Find the angles of intersection.
View SolutionA line through point P(4, 3) meets x-axis at point A and the y-axis at point B. If BP is double of PA, find the equation of AB.
View SolutionA line intersects x-axis at point (-2, 0) and cuts off an intercept of 3 units from the positive side of y-axis. Find the equation of the line.
View Solution

