The line joining P(-4, 5) and Q(3, 2) intersects the y-axis at point R. PM and QN are perpendiculars from P and Q on the x-axis. Find:
the area of the quadrilateral PMNQ.
Step by step video & image solution for The line joining P(-4, 5) and Q(3, 2) intersects the y-axis at point R. PM and QN are perpendiculars from P and Q on the x-axis. Find: the area of the quadrilateral PMNQ. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SECTION AND MID-POINT FORMULABOOK - ICSECHAPTER - SECTION AND MID-POINT FORMULAEXERCISE - EXERCISE 13 (B)
20 Videos
- SECTION AND MID-POINT FORMULABOOK - ICSECHAPTER - SECTION AND MID-POINT FORMULAEXERCISE - EXERCISE 13 (C)
28 Videos
- SAMPLE PAPER 5 (MATHEMATICS)BOOK - ICSECHAPTER - SAMPLE PAPER 5 (MATHEMATICS)EXERCISE - SECTION C
8 Videos
- SHARES AND DIVIDENDSBOOK - ICSECHAPTER - SHARES AND DIVIDENDSEXERCISE - Exercise 3(C )
20 Videos
Similar Questions
The line joining P(-4, 5) and Q(3, 2) intersects the y-axis at point R. PM and QN are perpendiculars from P and Q on the x-axis. Find:
the ratio PR : RQ
View SolutionThe line joining P(-4, 5) and Q(3, 2) intersects the y-axis at point R. PM and QN are perpendiculars from P and Q on the x-axis. Find:
the co-ordinates of R.
View SolutionThe line through P(5, 3) intersects y-axis at Q.
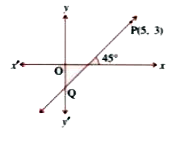
Find the co-ordinates of Q.
View SolutionThe line through P(5, 3) intersects y-axis at Q.
Write the slope of the line.
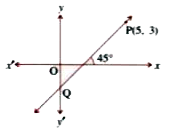
View SolutionThe line through P(5, 3) intersects y-axis at Q.

Write the equation of the line.
View SolutionUse a graph paper for this question . (Take two divisions = 1 unit on both the axes.)
Plot the points P (3,2) and Q(-3,-2) . From P and Q , draw perpendiculars PM and QN on the x - axis.
Assign the special name to geometrical figure PMQN and find its area.
View SolutionThe line through P (5, 3) intersects Y-axis at Q.
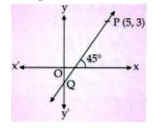
Write the equation of the line.
View SolutionA straight line passes through the points P(−1,4) and Q(5,−2). It intersects x-axis at point A and y-axis at point B. M is the midpoint of the line segment AB. Find :
the co-ordinates of point M.
View SolutionA line intersects the y-axis and x-axis at the points P and Q respectively. If (2, 5) is the mid-point of PQ, then find the coordinates of P and Q.
View SolutionUse a graph paper for this question . (Take two divisions = 1 unit on both the axes.)
Plot the points P (3,2) and (-3,-2) . From P and Q , draw perpendiculars PM and QN on the a - axis.
Write the co-ordinates of the point to which M is mapped on reflection in :
y - axis.
View Solution
