In a trapezium ABCD, side AB is parallel to side DC, and the diagonals AC and BD intersect each other at point P. Prove that :
PA×PD=PB×PC.
To prove that in trapezium ABCD, where AB is parallel to DC and the diagonals AC and BD intersect at point P, the relation PA×PD=PB×PC holds, we can follow these steps:
1. Draw the Trapezium: Start by drawing trapezium ABCD with AB parallel to DC. Label the points accordingly.
2. Draw the Diagonals: Draw the diagonals AC and BD, which intersect at point P.
3. Identify Angles:
- Note that angles ∠APB and ∠CPD are vertical angles. Therefore, ∠APB=∠CPD.
- Also, since AB is parallel to DC, the alternate interior angles ∠ABP and ∠CDP are equal. Thus, ∠ABP=∠CDP.
4. Establish Similarity:
- From the above angle relationships, we can conclude that triangles APB and CPD are similar by the AA (Angle-Angle) criterion of similarity (two angles of one triangle are equal to two angles of another triangle).
5. Set Up Proportions:
- Since triangles APB and CPD are similar, we can write the following proportion based on the corresponding sides:
PAPC=PBPD
6. Cross-Multiply:
- Cross-multiplying the proportions gives us:
PA×PD=PB×PC
7. Conclusion:
- Hence, we have proved that PA×PD=PB×PC.
- Note that angles ∠APB and ∠CPD are vertical angles. Therefore, ∠APB=∠CPD.
- Also, since AB is parallel to DC, the alternate interior angles ∠ABP and ∠CDP are equal. Thus, ∠ABP=∠CDP.
- From the above angle relationships, we can conclude that triangles APB and CPD are similar by the AA (Angle-Angle) criterion of similarity (two angles of one triangle are equal to two angles of another triangle).
- Since triangles APB and CPD are similar, we can write the following proportion based on the corresponding sides:
PAPC=PBPD
- Cross-multiplying the proportions gives us:
PA×PD=PB×PC
- Hence, we have proved that PA×PD=PB×PC.
Topper's Solved these Questions
- SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)BOOK - ICSECHAPTER - SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)EXERCISE - EXERCISE 15(B)
17 Videos
- SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)BOOK - ICSECHAPTER - SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)EXERCISE - EXERCISE 15(C)
24 Videos
- SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)BOOK - ICSECHAPTER - SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)EXERCISE - EXERCISE 15(E)
61 Videos
- SIMILARITY BOOK - ICSECHAPTER - SIMILARITY EXERCISE - MULTIPLE CHOICE QUESTIONS (COMPETENCY BASED QUESTIONS)
10 Videos
- SOLVING (SIMPLE) PROBLEMS (BASED ON QUADRATIC EQUATIONS)BOOK - ICSECHAPTER - SOLVING (SIMPLE) PROBLEMS (BASED ON QUADRATIC EQUATIONS)EXERCISE - Exercise 6(e )
18 Videos
Similar Questions
In a trapezium ABCD, side AB is parallel to side DC, and the diagonals AC and BD intersect each other at point P. Prove that :
ΔAPB is similar to ΔCPD.
View SolutionIn trapezium ABCD, side AB is parallel to side DC. Diagonals AC and BD intersect at point P. Prove that triangles APD and BPC are equal in area.
View SolutionIn quadrilateral ABCD, the diagonals AC and BD intersect each other at point O.
If AO = 2CO and BO = 2DO, show that:
OA×OD=OB×OC.
View SolutionThe given figure shows a trapezium in which AB is parallel to DC and diagonals AC and BD intersect at point P. If AP : CP = 3:5,

Find :
ΔAPB:ΔCPB
View SolutionThe given figure shows a trapezium in which AB is parallel to DC and diagonals AC and BD intersect at point P. If AP : CP = 3:5,
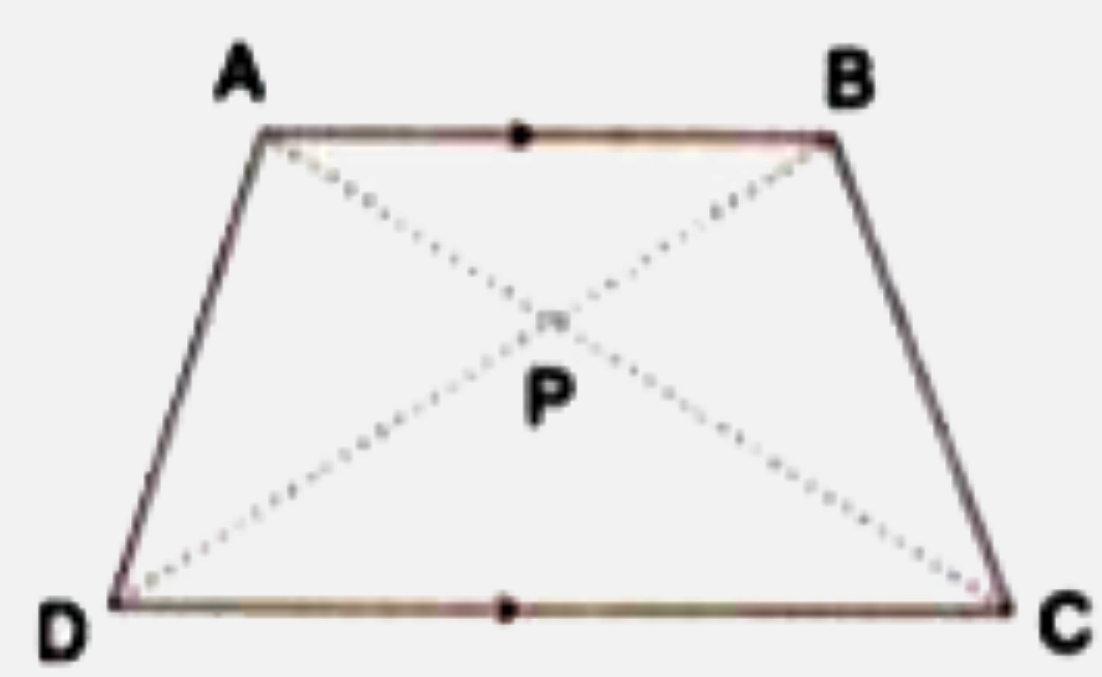
Find :
ΔDPC:ΔAPB
View SolutionThe given figure shows a trapezium in which AB is parallel to DC and diagonals AC and BD intersect at point P. If AP : CP = 3:5,

Find :
ΔAPB:ΔADB
View SolutionThe given figure shows a trapezium in which AB is parallel to DC and diagonals AC and BD intersect at point P. If AP : CP = 3:5,

Find :
ΔADP:ΔAPB
View SolutionThe given figure shows a trapezium in which AB is parallel to DC and diagonals AC and BD intersect at point O. If BO:OD=4:7, find
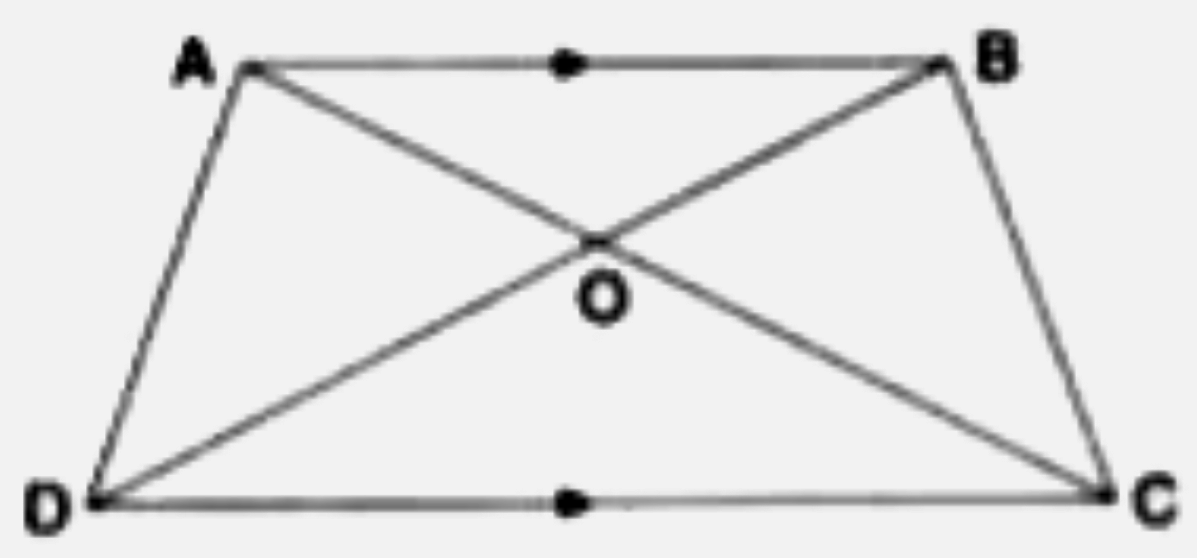
ΔAOB:ΔACB
View SolutionThe given figure shows a trapezium in which AB is parallel to DC and diagonals AC and BD intersect at point O. If BO:OD=4:7, find

ΔABD:ΔBOC
View SolutionThe given figure shows a trapezium in which AB is parallel to DC and diagonals AC and BD intersect at point O. If BO:OD=4:7, find

ΔDOC:ΔAOB
View Solution
