In ΔABC, angle ABC is equal to twice the angle ACB, and bisector of angle ABC meets the opposite side at point P. Show that :
CB:BA=CP:PA
To solve the problem, we need to show that in triangle ΔABC, if ∠ABC=2×∠ACB and the bisector of angle ABC meets the opposite side at point P, then the ratio CB:BA=CP:PA.
1. Define Angles:
Let ∠ACB=θ. Then, according to the problem, ∠ABC=2θ.
2. Identify Angles in Triangle:
In triangle ABC, the sum of angles is 180∘. Therefore:
∠CAB+∠ABC+∠ACB=180∘
Substituting the known angles:
∠CAB+2θ+θ=180∘
This simplifies to:
∠CAB+3θ=180∘
Thus, we find:
∠CAB=180∘−3θ
3. Use the Angle Bisector Theorem:
The angle bisector BP divides the opposite side AC into segments AP and PC such that:
APPC=ABBC
4. Express Ratios:
We need to show that:
CBBA=CPPA
From the angle bisector theorem, we have:
APPC=ABBC
This can be rewritten as:
PCAP=BCAB
Taking the reciprocal gives:
APPC=ABBC
5. Relate the Ratios:
Since AP+PC=AC, we can express AP and PC in terms of CP and PA:
CPPA=BCAB
Thus, we have:
CBBA=CPPA
6. Conclusion:
Therefore, we have shown that:
CB:BA=CP:PA
Let ∠ACB=θ. Then, according to the problem, ∠ABC=2θ.
In triangle ABC, the sum of angles is 180∘. Therefore:
∠CAB+∠ABC+∠ACB=180∘
Substituting the known angles:
∠CAB+2θ+θ=180∘
This simplifies to:
∠CAB+3θ=180∘
Thus, we find:
∠CAB=180∘−3θ
The angle bisector BP divides the opposite side AC into segments AP and PC such that:
APPC=ABBC
We need to show that:
CBBA=CPPA
From the angle bisector theorem, we have:
APPC=ABBC
This can be rewritten as:
PCAP=BCAB
Taking the reciprocal gives:
APPC=ABBC
Since AP+PC=AC, we can express AP and PC in terms of CP and PA:
CPPA=BCAB
Thus, we have:
CBBA=CPPA
Therefore, we have shown that:
CB:BA=CP:PA
Topper's Solved these Questions
- SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)BOOK - ICSECHAPTER - SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)EXERCISE - EXERCISE 15(B)
17 Videos
- SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)BOOK - ICSECHAPTER - SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)EXERCISE - EXERCISE 15(C)
24 Videos
- SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)BOOK - ICSECHAPTER - SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)EXERCISE - EXERCISE 15(E)
61 Videos
- SIMILARITY BOOK - ICSECHAPTER - SIMILARITY EXERCISE - MULTIPLE CHOICE QUESTIONS (COMPETENCY BASED QUESTIONS)
10 Videos
- SOLVING (SIMPLE) PROBLEMS (BASED ON QUADRATIC EQUATIONS)BOOK - ICSECHAPTER - SOLVING (SIMPLE) PROBLEMS (BASED ON QUADRATIC EQUATIONS)EXERCISE - Exercise 6(e )
18 Videos
Similar Questions
In ΔABC, angle ABC is equal to twice the angle ACB, and bisector of angle ABC meets the opposite side at point P. Show that :
AB×BC=BP×CA
View SolutionIn triangle ABC, side AC is greater than side AB. If the internal bisector of angle A meets the opposite side at point D, prove that : ∠ADC is greater than ∠ADB.
View SolutionIn triangle ABC, side AC is greater than side AB. If the internal bisector of angle A meets the opposite side at point D, prove that : ∠ADC is greater than ∠ADB.
View SolutionIn triangle ABC,the bisector of interior angle A and the bisector angle C meet at point O. Prove that ∠AOC=12∠B
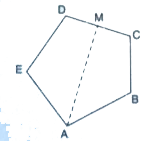
View SolutionABCDE is a regular pentagon. The bisector of angle A of the pentagon meet the side CD in point M. Show that ∠AMC=90∘.
View SolutionABC is a right triangle with AB = AC.If bisector of ∠A meet BC at D then prove that BC =2 AD .
View SolutionLet triangle ABC be an isosceles with AB=AC. Suppose that the angle bisector of its angle B meets the side AC at a point D and that BC=BD+AD. Measure of the angle A in degrees, is :
View SolutionTriangle ABC is right angles at B. Internet bisectors of acute angles A and C meet at point I. Find the measure of angle AIC
View SolutionTriangle ABC is similar to triangle PQR. If bisector of angle BAC meets BC at point D and bisector of angle QPR meets QR at point M, prove that : ABPQ=ADPM
View SolutionIn a triangle ABC , AB = AC and ∠A=36∘ If the internal bisector of angle C meets AB at D . Prove that AD = BC.
View Solution
