The horizontal distance between two towers is 75 m and the angular depression of the top of the first tower as see from the top of the second, which is 160 m high, is 45∘ . Find the height of the first tower.
80 m
81 m
85 m
None
The correct Answer is:C
To solve the problem step by step, we will use the information provided in the question and apply the concepts of trigonometry.
Step 1: Understand the Problem
We have two towers, Tower A (the first tower) and Tower B (the second tower). The height of Tower B (160 m) and the horizontal distance between the two towers (75 m) are given. The angle of depression from the top of Tower B to the top of Tower A is 45 degrees. We need to find the height of Tower A.
Step 2: Draw a Diagram
Draw a diagram to visualize the problem. Label the points:
- Point A: Top of Tower A
- Point B: Top of Tower B
- Point C: Base of Tower A
- Point D: Base of Tower B
The horizontal distance between points C and D (the bases of the two towers) is 75 m. The height of Tower B (point B) is 160 m. The angle of depression from point B to point A is 45 degrees.
Step 3: Identify the Triangle
From point B (top of Tower B), draw a line to point A (top of Tower A). This line forms a right triangle with the horizontal line from point B to point D (base of Tower B) and the vertical line from point D to point A (height of Tower A).
Step 4: Apply Trigonometry
In triangle BDA:
- The angle of depression is 45 degrees.
- The height of Tower B (BD) is 160 m.
- The horizontal distance (CD) is 75 m.
Since the angle of depression is equal to the angle of elevation from point A to point B, we can use the tangent function:
tan(45∘)=oppositeadjacent=h75
Where h is the height of Tower A (CD).
Step 5: Calculate the Height
Since tan(45∘)=1:
1=h75
Cross-multiplying gives:
h=75
Step 6: Find the Height of Tower A
Now, we need to find the height of Tower A (CD). The total height of Tower B (160 m) minus the height from point D to point A (75 m) gives us:
CD=160−75=85 m
Conclusion
The height of the first tower (Tower A) is 85 meters.
We have two towers, Tower A (the first tower) and Tower B (the second tower). The height of Tower B (160 m) and the horizontal distance between the two towers (75 m) are given. The angle of depression from the top of Tower B to the top of Tower A is 45 degrees. We need to find the height of Tower A.
Draw a diagram to visualize the problem. Label the points:
- Point A: Top of Tower A
- Point B: Top of Tower B
- Point C: Base of Tower A
- Point D: Base of Tower B
From point B (top of Tower B), draw a line to point A (top of Tower A). This line forms a right triangle with the horizontal line from point B to point D (base of Tower B) and the vertical line from point D to point A (height of Tower A).
In triangle BDA:
- The angle of depression is 45 degrees.
- The height of Tower B (BD) is 160 m.
- The horizontal distance (CD) is 75 m.
tan(45∘)=oppositeadjacent=h75
Where h is the height of Tower A (CD).
Since tan(45∘)=1:
1=h75
Cross-multiplying gives:
h=75
Now, we need to find the height of Tower A (CD). The total height of Tower B (160 m) minus the height from point D to point A (75 m) gives us:
CD=160−75=85 m
The height of the first tower (Tower A) is 85 meters.
Topper's Solved these Questions
- HEIGHTS AND DISTANCES Book:ICSEChapter:HEIGHTS AND DISTANCES Exercise:Exercise 22 C
Explore 28 Videos
- HEIGHTS AND DISTANCES Book:ICSEChapter:HEIGHTS AND DISTANCES Exercise:Exercise 22 A
Explore 14 Videos
- GST [GOODS AND SERVICES TAX] Book:ICSEChapter:GST [GOODS AND SERVICES TAX] Exercise:Exercise 1(B)
Explore 16 Videos
- LINEAR INEQUATIONSBook:ICSEChapter:LINEAR INEQUATIONSExercise:Competency Based Questions
Explore 15 Videos
Similar Questions
The horizontal distance between two tower is 150 m. The angle of depression of the top of one tower as observed from the top of other tower, which is 120 m in hight, is 30∘. Find the height of the first tower.
View SolutionThe horizontal distance between two towers is 140m. The angle of elevation of the top of the first tower when seen from the top of the second tower is 300. If the height of the second tower is 60m, find the height of the first tower.
View SolutionThe horizontal distance between two trees of different heights is 60m. The angle of depression of the top of the first tree when seen from the top of the second tree is 45o . If the height of the second tree is 80m, find the height of the first tree.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 40 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower is 30∘ . Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower, is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe horizontal distance between two tower is 120 m. The angle of elevation of the top and angle of depression of the bottom of the first tower as observed from the second tower is 30∘and24∘ respectively.
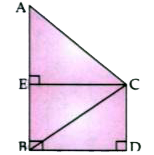
Find the height of the two towers. Give youe answer correct to 3 significant figures.
View SolutionThe angle elevation of the top of a tower from a point C on the ground. Which is 30 m away from the foot of the tower is 30∘ . Find the height of the tower.

View SolutionThe distance between two towers is 140 m while seeing from the top if the second tower, the angle of elevation of first tower is 30∘.If the height of the second tower is 60 m, then find the height of the first tower.
View Solution