The horizontal distance between two tower is 150 m. The angle of depression of the top of one tower as observed from the top of other tower, which is 120 m in hight, is 30∘. Find the height of the first tower.
To solve the problem step by step, we will follow the information provided and apply trigonometric principles.
Step 1: Understand the Problem
We have two towers. The height of the second tower (Tower B) is 120 m, and the horizontal distance between the two towers is 150 m. The angle of depression from the top of Tower B to the top of Tower A is 30 degrees. We need to find the height of Tower A.
Step 2: Draw a Diagram
Draw two vertical lines representing the two towers. Label the top of Tower A as point A and the top of Tower B as point B. The ground level between the two towers can be labeled as point C, and the horizontal distance between the two towers can be represented as line segment BC, which is 150 m.
Step 3: Identify the Angles and Heights
From point B (top of Tower B), the angle of depression to point A (top of Tower A) is 30 degrees. The height of Tower B (AB) is 120 m.
Step 4: Use Trigonometry
In triangle ABE, where:
- AB = height of Tower B = 120 m
- BC = horizontal distance = 150 m
- Angle of depression = 30 degrees
We can use the tangent function, which relates the angle of depression to the opposite side (height difference) and the adjacent side (horizontal distance).
Step 5: Set Up the Equation
Using the tangent of the angle of depression:
tan(30∘)=AEED
Where:
- AE is the height difference between the two towers.
- ED is the horizontal distance (150 m).
Step 6: Substitute Known Values
We know that:
tan(30∘)=1√3
So, we can write:
1√3=AE150
Step 7: Solve for AE
Rearranging the equation gives:
AE=150⋅1√3=150√3=50√3 m
Step 8: Calculate the Height of Tower A
The height of Tower A (DC) can be found using:
DC=AB−AE
Substituting the known values:
DC=120−50√3
Final Answer
The height of the first tower (Tower A) is:
DC=120−50√3 m
We have two towers. The height of the second tower (Tower B) is 120 m, and the horizontal distance between the two towers is 150 m. The angle of depression from the top of Tower B to the top of Tower A is 30 degrees. We need to find the height of Tower A.
Draw two vertical lines representing the two towers. Label the top of Tower A as point A and the top of Tower B as point B. The ground level between the two towers can be labeled as point C, and the horizontal distance between the two towers can be represented as line segment BC, which is 150 m.
From point B (top of Tower B), the angle of depression to point A (top of Tower A) is 30 degrees. The height of Tower B (AB) is 120 m.
In triangle ABE, where:
- AB = height of Tower B = 120 m
- BC = horizontal distance = 150 m
- Angle of depression = 30 degrees
Using the tangent of the angle of depression:
tan(30∘)=AEED
Where:
- AE is the height difference between the two towers.
- ED is the horizontal distance (150 m).
We know that:
tan(30∘)=1√3
So, we can write:
1√3=AE150
Rearranging the equation gives:
AE=150⋅1√3=150√3=50√3 m
The height of Tower A (DC) can be found using:
DC=AB−AE
Substituting the known values:
DC=120−50√3
The height of the first tower (Tower A) is:
DC=120−50√3 m
Topper's Solved these Questions
Similar Questions
The horizontal distance between two towers is 140m. The angle of elevation of the top of the first tower when seen from the top of the second tower is 300. If the height of the second tower is 60m, find the height of the first tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 40 m away from the foot of the tower is 30∘. Find the height of the tower.
View Solution
Knowledge Check
The horizontal distance between two towers is 75 m and the angular depression of the top of the first tower as see from the top of the second, which is 160 m high, is 45∘ . Find the height of the first tower.
A80 mB81 mC85 mDNone
The angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower, is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower is 30∘ . Find the height of the tower.
View SolutionThe horizontal distance between two tower is 120 m. The angle of elevation of the top and angle of depression of the bottom of the first tower as observed from the second tower is 30∘and24∘ respectively.
Find the height of the two towers. Give youe answer correct to 3 significant figures.
View SolutionThe angle elevation of the top of a tower from a point C on the ground. Which is 30 m away from the foot of the tower is 30∘ . Find the height of the tower.

View Solution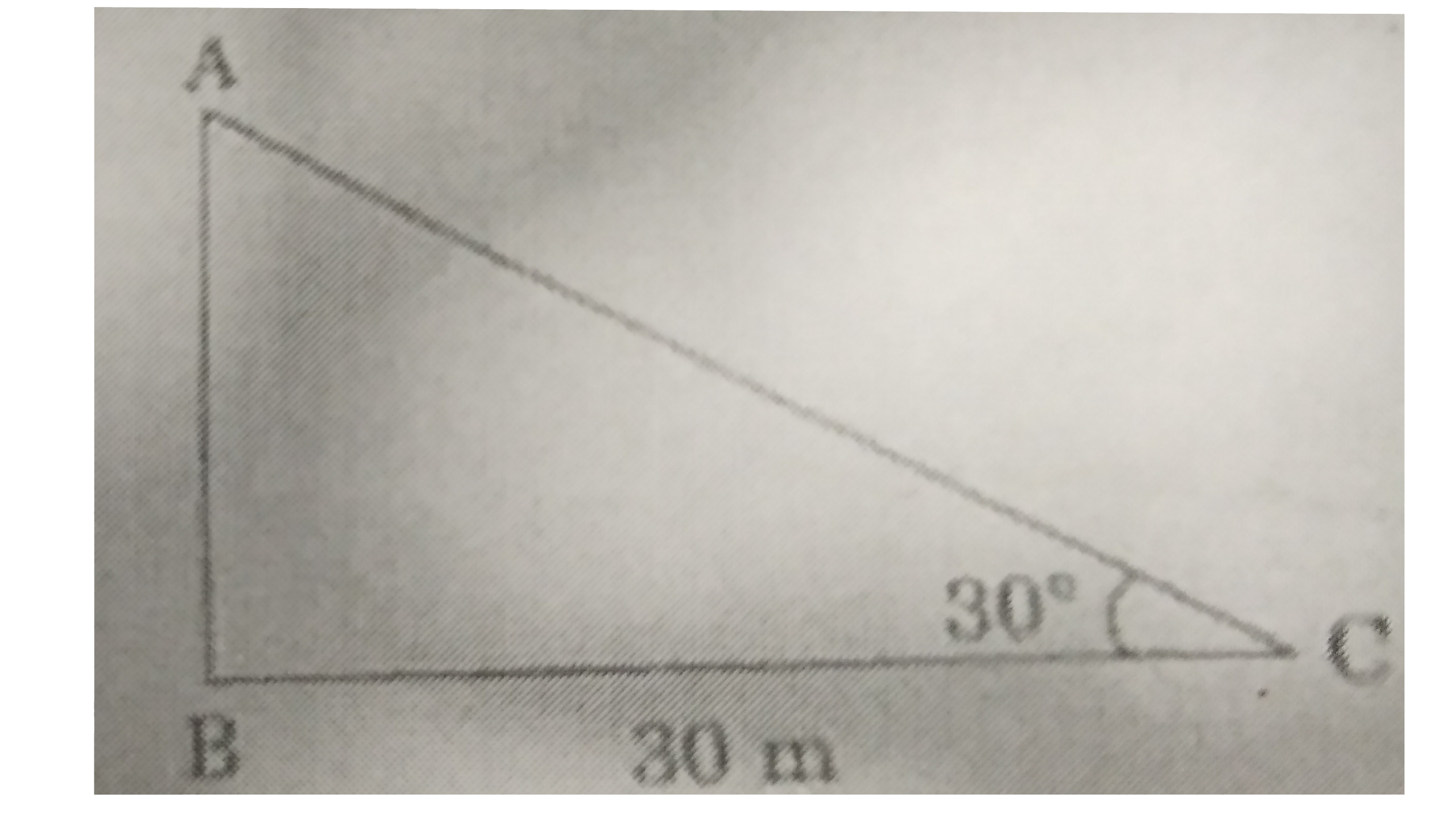
If figure, the angle of elevation of the top of a tower from a point C on the ground which is 30 m away from the foot of the tower, is 30∘. Find th height of the tower.
View SolutionA particle is dropped from the top of a tower. It covers 40 m in last 2s. Find the height of the tower.
View Solution
