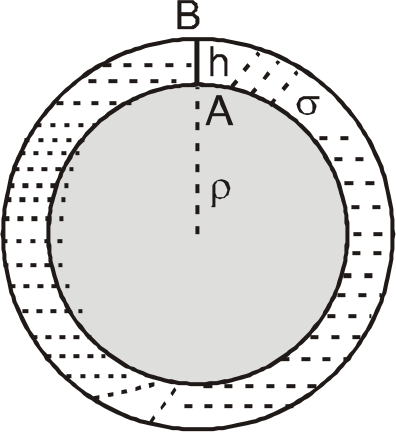
Suppose the earth was covered by an oceam of uniform depth $h, (h < < R)$ Let $σ$ be densityof ocean and $p$ be mean density of earth. Let $Δ g$ be the approxemate difference of value of net acceleration duet to gravity between the bottom of the ocean and top $(Δ g = g_{top} - g_{bottom})$ . Choose the correct option.

A

$Δ g = \frac{4}{3} π G h [2 p - 3 σ]$

B

$Δ g = \frac{4}{3} G π h [3 σ - 2 p]$

C

$Δ g = \frac{4}{3} G π h [2 σ - 3 σ]$

D

$Δ g = \frac{4}{3} G π h [3 p - 3 σ]$

Video Solution

Text Solution

Verified by Experts

$g_{A} = \frac{4}{3} G π R p$
$g_{e} = \frac{G [\frac{4}{3} π p R^{3} + [\frac{4}{3} π {(R + h)}^{3} - \frac{4}{3} π R^{2}] σ]}{{(R + h)}^{2}}$
$= \frac{4}{3} G π p R [1 - \frac{2 h}{R}] \frac{4}{3} G π [(R + h) - (1 - \frac{2 h}{R}) R] σ$
$Δ g = g_{B} - 9_{A} = \frac{4}{3} π G h [3 σ - 2 p]$

|

Updated on:21/07/2023

Class 12 PHYSICS QUESTION BANK

Topper's Solved these Questions

Similar Questions

RESONANCE ENGLISH-REVISION DPP-All Questions

Home
Ask Doubt
Profile