AB is a light rigid rod, which is rotating about a vertical axis passing through end A. A spring of force constant k and natural length l is attached at A and its other end attached to a small bead of mass m. The bead can slide without friction on the rod. At the initial moment, the bead is at rest (w.r.t the rod) and the spring is unstretched. Select incorrect options :
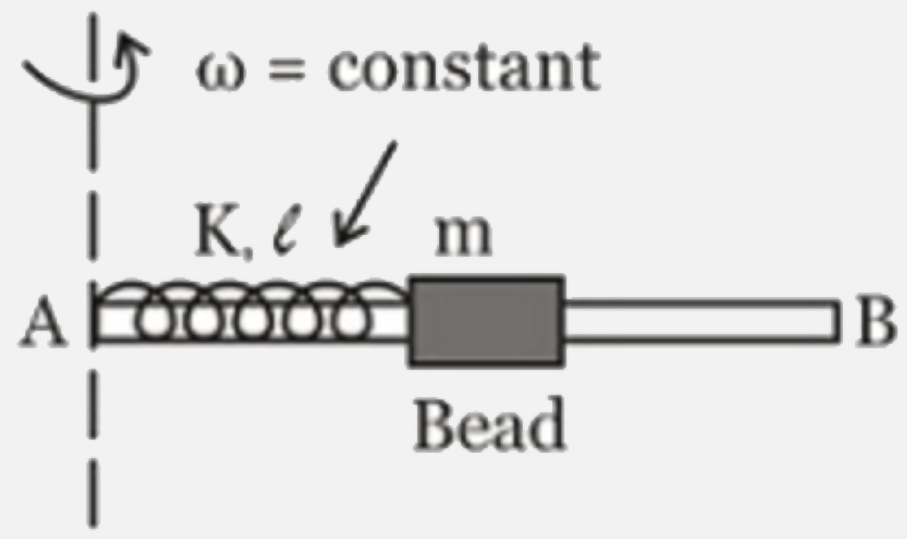
The maximum velocity attained by the bead w.r.t. the rod is given by Vmax=√mω4l2k−mω2
The maximum velocity attained by the bead w.r.t the rod is given by Vmax=√(mω4+Kmω2−K)ω2l2
The maximum extension in the spring in given by Xmax=2mω2lK−mω2
The maximum value of contact force between the bead and the rod is greater than mg
Velocity will be maximum at equilibrium position
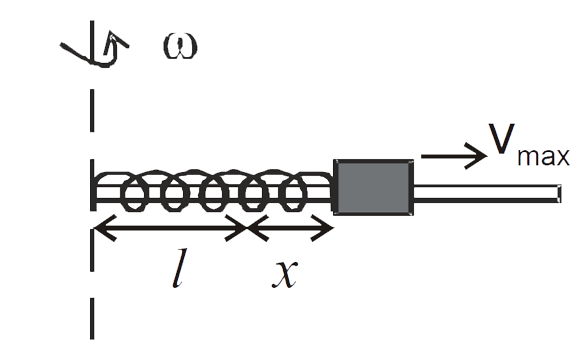
⇒mω2(l+x)=kx
⇒x=mω2lK−mω2
12mV2max=x∫0mω2(l+x)dx−12kx2
⇒Vmax=2mω2lx+mω2x2−kx2m
V2max=(mω2l+mω2(l+x)−kx)xm
⇒V2max=ω2l=mω4l2mω2−K
Vmax=√mω4l2k−mω2
For maximum extension
x∫0mω2(l+x)dx−12kx2max=0⇒xmax=2mω2lk−mω2
Topper's Solved these Questions
Similar Questions
AB is a light rigid rod. Which is rotating about a vertical axis passing through A,A spring of force constant K and natural length l is attached at A and its other end is attached to a small bead of mass m. The bead can slide without friction on the rod. At the initial moment the bead is at rest (w.rt. the rod) and the spring is unstreached Select correct option
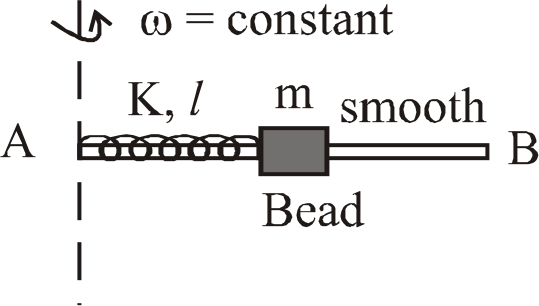
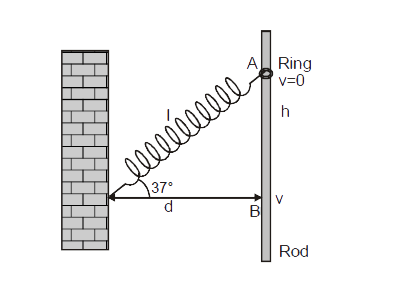
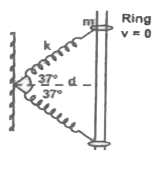
View SolutionOne end of a light spring of natural length d and spring constant k is fixed on a rigid wall and the other is attached to a smooth ring of mass m which can slide without friction on a vertical rod fixed at a distance d from the wall. Initially the spring makes an angle of 37∘ with the horizontal as shown in fig. When the system is released from rest, find the speed of the ring when the spring becomes horizontal.
[ sin 37∘ = 3/5]
View Solution
Knowledge Check
The radius of gyration of an uniform rod of length l about an axis passing through one of its ends and perpendicular to its length is.
Al√2Bl3Cl√3Dl2
On end of a light spring of natural length d and spring constant k is fixed on a rigid wall and the other is attached to a smooth ring of mass m which can slide without friction on a vertical rod fixed at a distance d from the wall. Initially the spring makes an angle of 37∘ with the horizontal. as shown in figure. When the system is released from rest, find the speed of the ring when the spring becomes horizontal. (sin37∘=35).

View SolutionA bead of mass m can slide without friction along a vertical ring of radius R. One end of a spring of force constant k=3mgR is connected to the bead and the other end is fixed at the centre of the ring. Initially, the bead is at the point A and due to a small push it starts sliding down the ring. If the bead momentarily loses contact with the ring at the instant when the spring makes an angle 60∘ with the vertical, then the natural length of the spring is
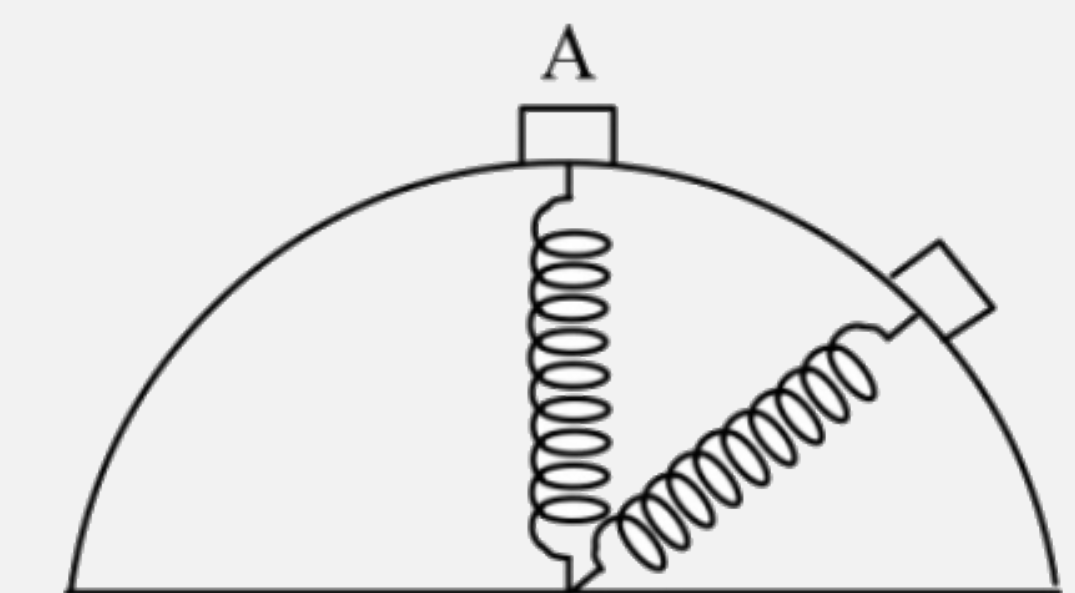
View SolutionA rod of length l=2m is maintained to rotate with a constant angular velocity ω=1rad/s about vertical axis passing through one end (fig). There is a spring of spring constant k=1N/m which just encloses rod inside it in natural length. One end of the spring is attached to axis of rotation. S is sleeve of mass m=1kg which can just fix on rod. All surfaces are smooth. With what minimum kinetic energy (in J) sleeve should be projected so that it enters on the rod without impulse and completely compresses the spring.

View SolutionOne end a light spring of natural length d and spring constant k(=mg/d) is fixed on a rigid support and the other end is fixed to a smoth ring of mass m which can slide without friction on a vertical rod fixed at a distance d from the support. Initially , the spring makes an angle of 37∘ with the horizontal as shown in the figure. The system is released from rest. The speed of the ring at the same angle subtended downward will be
View SolutionA rod of mass M and length l attached with a small bob of mass m at its end is freely rotating about a vertical axis passing through its other end with a constant angular speed ω. Find the force exerted by the system (rod and bob) on the pivot. Assume that gravity is absent.
View SolutionMoment of inertia of a thin rod of mass m and length l about an axis passing through a point l/4 from one end and perpendicular to the rod is
View SolutionA long uniform rod of length L and mass M is free to rotate in a horizontal plane about a vertical axis through its one end 'O'. A spring of force constant k is connected between one end of the rod and PQ. When the rod is in equilibrium it is parallel to PQ.
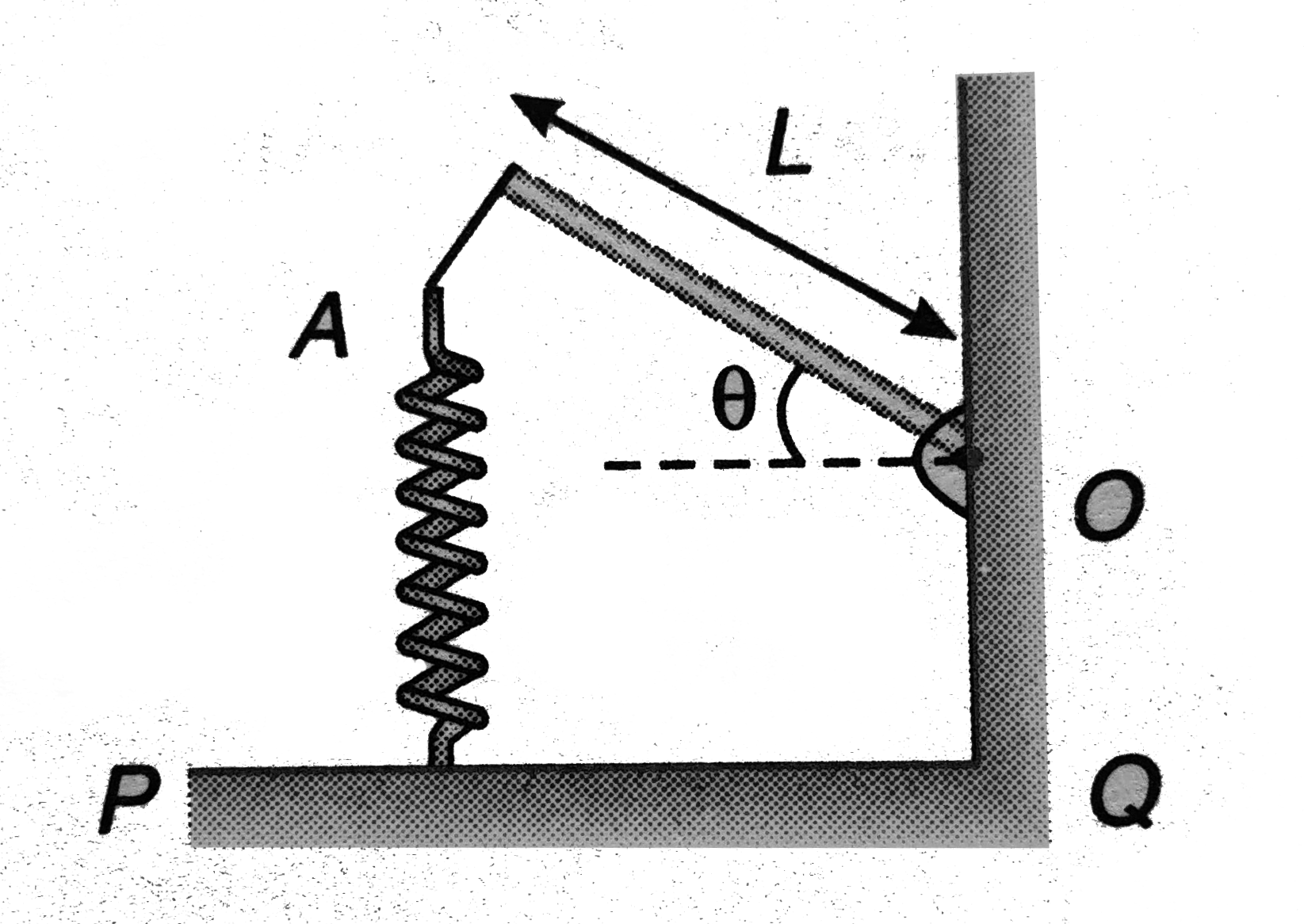
(a)What is the period of small oscillation that result when the rod is rotated slightly and released ?
(b) What will be the maximum speed of the displacement end of the rod, if the amplitude of motion is θ0?
View Solution
