A steel scale is to be prepared such that the millimeter intervals are to be accurate within 6×10−5mm. The maximum temperature variation form the temperature of calibration during the reading of the millimeter marks is (α=12×10−6/∘C)
4.0∘C
4.5∘C
5.0∘C
5.5∘C
The correct Answer is:C
To solve the problem step by step, we will use the formula for linear expansion and the given data.
Step 1: Identify the given values
- Length of the interval (L) = 1 mm = 1×10−3 m (convert to meters for consistency)
- Change in length (ΔL) = 6×10−5 mm = 6×10−8 m (convert to meters)
- Coefficient of linear expansion (α) = 12×10−6°C−1
Step 2: Write down the formula for linear expansion
The formula for linear expansion is given by:
ΔL=L⋅α⋅ΔT
where:
- ΔL = change in length
- L = original length
- α = coefficient of linear expansion
- ΔT = change in temperature
Step 3: Rearrange the formula to solve for ΔT
We need to isolate ΔT:
ΔT=ΔLL⋅α
Step 4: Substitute the known values into the equation
Substituting the values we have:
ΔT=6×10−8m(1×10−3m)⋅(12×10−6°C−1)
Step 5: Calculate ΔT
Calculating the denominator:
(1×10−3m)⋅(12×10−6°C−1)=12×10−9m°C−1
Now substituting back into the equation:
ΔT=6×10−812×10−9=612×101=0.5×101=5°C
Step 6: Conclusion
The temperature variation (ΔT) is 5°C.
Final Answer
ΔT=5°C
---
- Length of the interval (L) = 1 mm = 1×10−3 m (convert to meters for consistency)
- Change in length (ΔL) = 6×10−5 mm = 6×10−8 m (convert to meters)
- Coefficient of linear expansion (α) = 12×10−6°C−1
The formula for linear expansion is given by:
ΔL=L⋅α⋅ΔT
where:
- ΔL = change in length
- L = original length
- α = coefficient of linear expansion
- ΔT = change in temperature
We need to isolate ΔT:
ΔT=ΔLL⋅α
Substituting the values we have:
ΔT=6×10−8m(1×10−3m)⋅(12×10−6°C−1)
Calculating the denominator:
(1×10−3m)⋅(12×10−6°C−1)=12×10−9m°C−1
Now substituting back into the equation:
ΔT=6×10−812×10−9=612×101=0.5×101=5°C
The temperature variation (ΔT) is 5°C.
ΔT=5°C
Topper's Solved these Questions
- CALORIMETRY AND THERMAL EXPANSIONBOOK - RESONANCE ENGLISHCHAPTER - CALORIMETRY AND THERMAL EXPANSIONEXERCISE - Exercise-2
1 Video
- CALORIMETRY AND THERMAL EXPANSIONBOOK - RESONANCE ENGLISHCHAPTER - CALORIMETRY AND THERMAL EXPANSIONEXERCISE - Exercie-3
1 Video
- CALORIMETRY AND THERMAL EXPANSIONBOOK - RESONANCE ENGLISHCHAPTER - CALORIMETRY AND THERMAL EXPANSIONEXERCISE - Exercise-1
1 Video
- CALORIMETRYBOOK - RESONANCE ENGLISHCHAPTER - CALORIMETRYEXERCISE - Exercise
19 Videos
- CAPACITORBOOK - RESONANCE ENGLISHCHAPTER - CAPACITOREXERCISE - Exercise
45 Videos
Similar Questions
A steel tape measures that length of a copper rod as 90.0 cm when both are at 10∘C, the calibration temperature, for the tape. What would the tape read for the length of the rod when both are at 30∘C. Given αsteel=1.2×10−5 per.∘CandαCu=1.7×10−5per.∘C
View SolutionWhat is the percentage change in length of 1m iron rod it its temperature changes by 100∘C.α for iron is 2×10−5/∘C.
View Solution
Knowledge Check
A steel metre scale is to be ruled so that the millimetre intervals are accurate to within about 5×10−5mm at a certain temperature. What is the maximum temperature variation allowable during the ruling ? Given α for steel =1.1×10−5.∘C−1.
A8∘CB9∘CC4.5∘CD10∘C
The scale on a steel meter stick is calibrated at 15∘C. What is the error in the reading of 60cm at 27∘C ? αsteel=1.2×10−5(.∘C)−1.
View SolutionA steel tape is correctly calibrated at 20∘C and is used to measure the length of a table at 30∘C. Find the percentage error in the measurement of length. [αsteel=11×10−5/.∘C]
View SolutionThe temperature of sun is 5500 K and it emits maximum intensity radiation in the yellow region(5.5×10−7m). The maximum radiation from a furnace occurs at wavelength 11×10−7m The temperature of furnace is
View SolutionA steel tape placed around the earth at the equator when the temperature is 10∘C. What will be the clearance between the tape and the ground (assumed to be uniform) if the temperature of the tape rises to 40∘C? Neglect expansion of the earth. Radius of earth at equator is 6400km & αsteel=1.2×10−5K−1
View SolutionA rail track made of steel having length 10 m is clamped on a railway line at its two ends
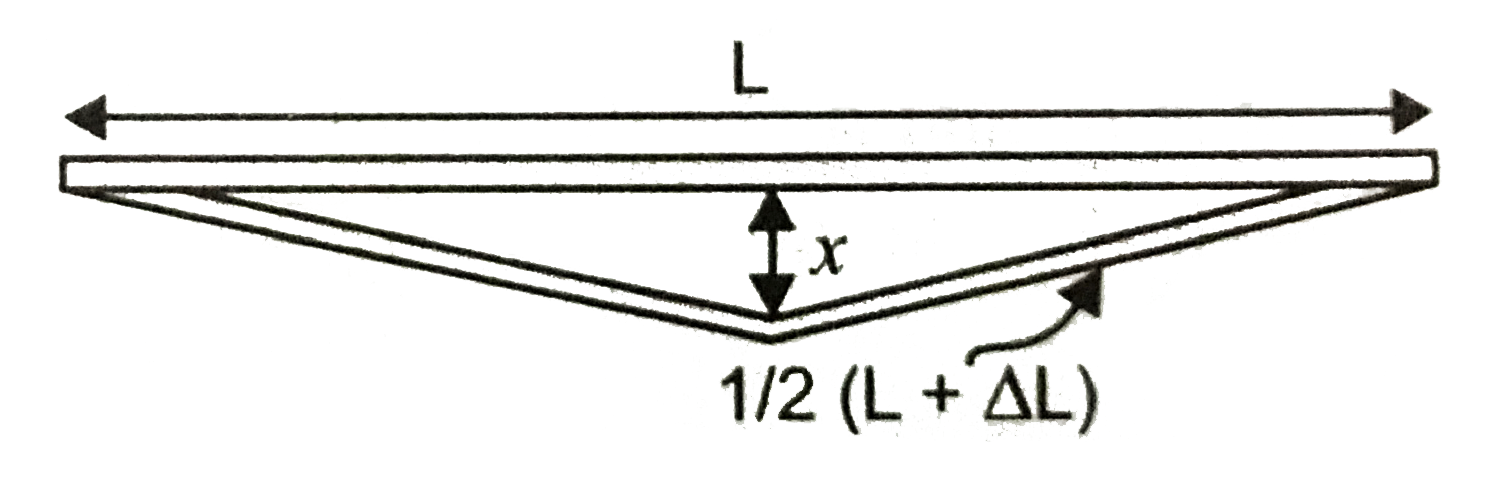
on a summer day due to rise in temperature by 20∘C, it is deformed as shown in fig. Find x (displacement of the centre) if αsteel=1.2×10−5/∘C.
View Solution
The variation of length of two metal rods A and B with change in temperature is shown in Fig. the coefficient of linear expansion αA for the metal A and the temperature T will be? ( alpha_(A)=3x10^(-6)/ C )
View SolutionThe wavelength of maximum energy released during an atomic axplosion was 2.93×10−10m. Given that Wien's constant is 2.93×10−3m−K, the maximum temperature attained must be of the order of
View Solution