A liquid with coefficient of volume expansion γ is filled in a container of a material having coefficient of linear expansion α . If the liquid overflows on heating, then
γ>3α
γ<3α
γ=3α
none of these
The correct Answer is:A
To solve the problem, we need to analyze the thermal expansion of both the liquid and the container when heated. Here’s a step-by-step solution:
Step 1: Understand the initial conditions
Let the initial volume of the liquid and the container be V0.
Step 2: Calculate the new volume of the liquid after heating
The volume expansion of the liquid can be expressed using the coefficient of volume expansion γ:
VL=V0(1+γΔT)
where ΔT is the change in temperature.
Step 3: Calculate the new volume of the container after heating
Assuming the container is cubical with side length L, the initial volume is:
V0=L3
After heating, the new side length L′ of the container can be expressed as:
L′=L(1+αΔT)
Thus, the new volume of the container VC becomes:
VC=(L′)3=L3(1+αΔT)3
Using the binomial expansion, we can approximate this as:
VC≈V0(1+3αΔT)(neglecting higher order terms)
Step 4: Set up the inequality for overflow
For the liquid to overflow, the volume of the liquid after heating must be greater than the volume of the container after heating:
VL>VC
Substituting the expressions from Steps 2 and 3:
V0(1+γΔT)>V0(1+3αΔT)
Step 5: Simplify the inequality
Dividing both sides by V0 (assuming V0>0):
1+γΔT>1+3αΔT
Subtracting 1 from both sides:
γΔT>3αΔT
Since ΔT is positive, we can safely divide both sides by ΔT:
γ>3α
Conclusion
Thus, the condition for the liquid to overflow when heated is:
γ>3α
Let the initial volume of the liquid and the container be V0.
The volume expansion of the liquid can be expressed using the coefficient of volume expansion γ:
VL=V0(1+γΔT)
where ΔT is the change in temperature.
Assuming the container is cubical with side length L, the initial volume is:
V0=L3
After heating, the new side length L′ of the container can be expressed as:
L′=L(1+αΔT)
Thus, the new volume of the container VC becomes:
VC=(L′)3=L3(1+αΔT)3
Using the binomial expansion, we can approximate this as:
VC≈V0(1+3αΔT)(neglecting higher order terms)
For the liquid to overflow, the volume of the liquid after heating must be greater than the volume of the container after heating:
VL>VC
Substituting the expressions from Steps 2 and 3:
V0(1+γΔT)>V0(1+3αΔT)
Dividing both sides by V0 (assuming V0>0):
1+γΔT>1+3αΔT
Subtracting 1 from both sides:
γΔT>3αΔT
Since ΔT is positive, we can safely divide both sides by ΔT:
γ>3α
Thus, the condition for the liquid to overflow when heated is:
γ>3α
Topper's Solved these Questions
- CALORIMETRY AND THERMAL EXPANSIONBOOK - RESONANCE ENGLISHCHAPTER - CALORIMETRY AND THERMAL EXPANSIONEXERCISE - Exercise-2
1 Videos
- CALORIMETRY AND THERMAL EXPANSIONBOOK - RESONANCE ENGLISHCHAPTER - CALORIMETRY AND THERMAL EXPANSIONEXERCISE - Exercie-3
1 Videos
- CALORIMETRY AND THERMAL EXPANSIONBOOK - RESONANCE ENGLISHCHAPTER - CALORIMETRY AND THERMAL EXPANSIONEXERCISE - Exercise-1
1 Videos
- CALORIMETRYBOOK - RESONANCE ENGLISHCHAPTER - CALORIMETRYEXERCISE - Exercise
19 Videos
- CAPACITORBOOK - RESONANCE ENGLISHCHAPTER - CAPACITOREXERCISE - Exercise
45 Videos
Similar Questions
Unit of coefficient of linear expansion
View SolutionDefine the coefficient of linear expansion.
View SolutionOn heating a liquid having coefficient of volume expension α in a container having coefficient of linear expansion α/2, the level of the liquid in the container would
View SolutionA fluid having a thermal coefficient of volume expansion γ is filled in a cylindrical vessel up to a height h0. The coefficient of linear thermal expansion of the material of the vessel is α . If the fluid is heated with the vessel , then find the level of liquid when temperature increases by Δθ.
View SolutionIn figure which strip brass or steel have higher coefficient of linear expansion.
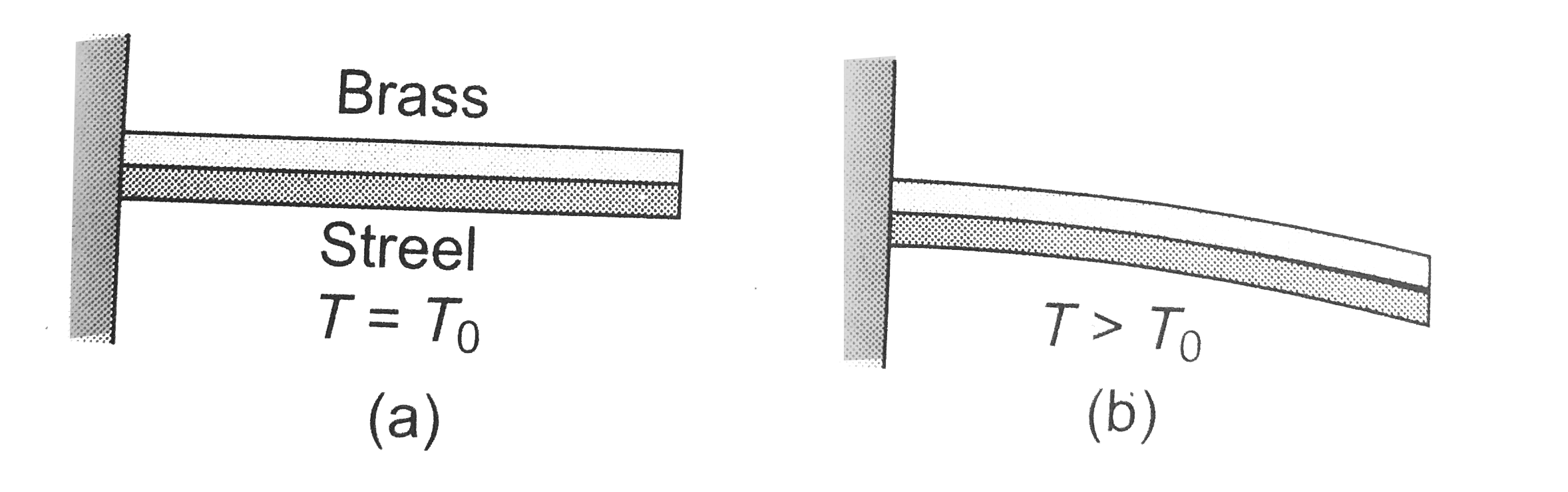
View SolutionMercury has a small coefficient of volume expansion.
View SolutionLet γ : coefficient of volume expansion of the liquid and α : coefficient of linear expansion of the material of the tube
View SolutionCoefficient of linear and coefficient of superficial expansion
View SolutionCoefficient of areal expansion of a solid is 2×10−5.∘C−1. Calculate its coefficient of linear expansion.
View SolutionRelate the thermal coefficient of linear expansion, α, the the thermal coefficient of area expansion β.
View Solution
