On heating a liquid having coefficient of volume expension in a container having coefficient of linear expansion the level of the liquid in the container would
rise
fall
remains almost stationary
Cannot be bredicted
The correct Answer is:B
To solve the problem, we need to analyze the effects of thermal expansion on both the liquid and the container when they are heated.
1. Identify the Coefficients of Expansion:
- The coefficient of volume expansion of the liquid is given as α.
- The coefficient of linear expansion of the container is given as α2.
2. Relate Linear Expansion to Volume Expansion:
- The volume expansion coefficient (γ) of a material is related to its linear expansion coefficient (α) by the formula:
γ=3α(for three-dimensional expansion)
- For the container, since it has a linear expansion coefficient of α2, the volume expansion coefficient of the container (γc) will be:
γc=3(α2)=3α2
3. Compare the Coefficients:
- Now we compare the volume expansion coefficients:
- Liquid: γL=α
- Container: γc=3α2
- Since 3α2>α, the container expands more than the liquid when heated.
4. Effect on Liquid Level:
- Initially, assume the liquid fills the container to the top. As the container expands more than the liquid, the space available in the container increases more than the volume of the liquid.
- Therefore, the level of the liquid in the container will fall.
5. Conclusion:
- The level of the liquid in the container will decrease upon heating.
Final Answer:
The level of the liquid in the container would fall.
---
- The coefficient of volume expansion of the liquid is given as α.
- The coefficient of linear expansion of the container is given as α2.
- The volume expansion coefficient (γ) of a material is related to its linear expansion coefficient (α) by the formula:
γ=3α(for three-dimensional expansion)
- For the container, since it has a linear expansion coefficient of α2, the volume expansion coefficient of the container (γc) will be:
γc=3(α2)=3α2
- Now we compare the volume expansion coefficients:
- Liquid: γL=α
- Container: γc=3α2
- Since 3α2>α, the container expands more than the liquid when heated.
- Initially, assume the liquid fills the container to the top. As the container expands more than the liquid, the space available in the container increases more than the volume of the liquid.
- Therefore, the level of the liquid in the container will fall.
- The level of the liquid in the container will decrease upon heating.
The level of the liquid in the container would fall.
Topper's Solved these Questions
- THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESBook:DC PANDEY ENGLISHChapter:THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESExercise:Check point 14.3
Explore 20 Videos
- THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESBook:DC PANDEY ENGLISHChapter:THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESExercise:Check point 14.4
Explore 25 Videos
- THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESBook:DC PANDEY ENGLISHChapter:THERMOMETRY THERMAL EXPANSION AND KINETIC THEORY OF GASESExercise:Check point 14.1
Explore 10 Videos
- SUPERPOSITION OF WAVESBook:DC PANDEY ENGLISHChapter:SUPERPOSITION OF WAVESExercise:Level 2 Subjective
Explore 8 Videos
- THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASESBook:DC PANDEY ENGLISHChapter:THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASESExercise:Level 2 Subjective
Explore 9 Videos
Similar Questions
A liquid with coefficient of volume expansion γ is filled in a container of a material having coefficient of linear expansion α . If the liquid overflows on heating, then
View SolutionA liquid with coefficient of volume expansion γ is filled in a container of a material having coefficient of linear expansion α . If the liquid overflows on heating, then
View SolutionLet γ : coefficient of volume expansion of the liquid and α : coefficient of linear expansion of the material of the tube
View SolutionRelate the thermal coefficient of linear expansion, α, the the thermal coefficient of area expansion β.
View SolutionCan we related the thermal coefficient of linear expansion, α, to the thermal coefficient of volume expansion?
View SolutionIn figure which strip brass or steel have higher coefficient of linear expansion.
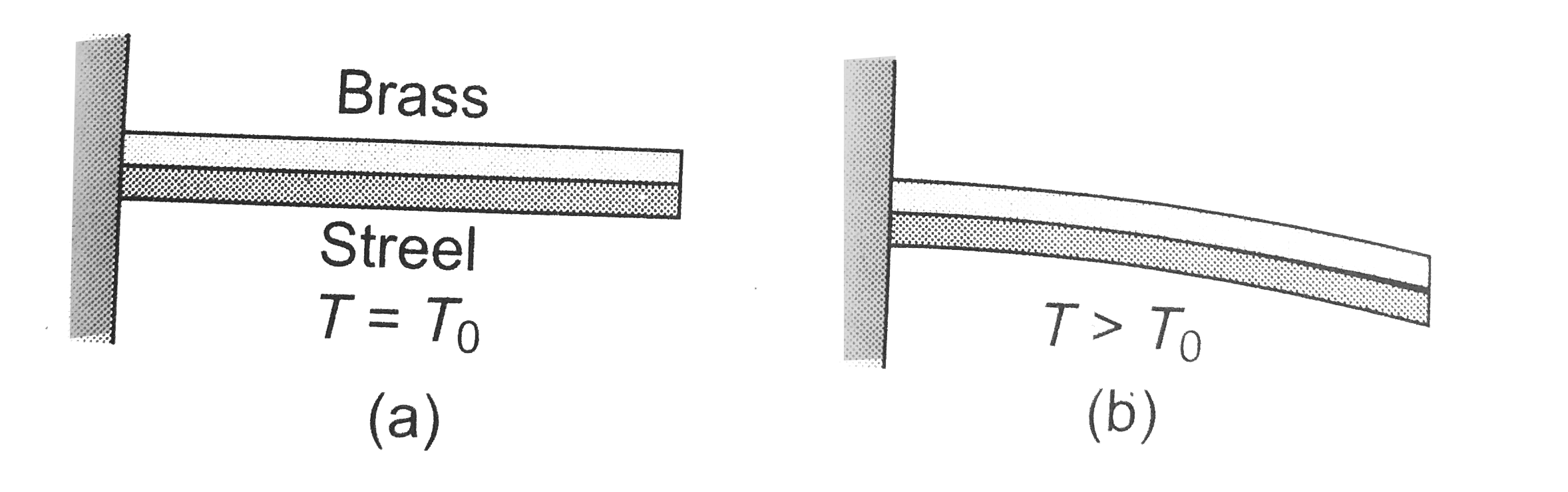
View SolutionTwo metallic rods of length l and 3l have coefficient of linear expansion α and 3α respectively. The coefficient of linear expansion ofr their series combinations, is
View SolutionA thread of liquid is in a uniform capillary tube of length L. As measured by a ruler. The temperature of the tube and thread of liquid is raised by ΔT. If γ be the coefficient of volume expansion of the liquid and α be the coefficient of linear expansion of the material of the tube, then the increase ΔL in the length of the thread, again measured by the ruler will be
View SolutionA thread of liquid is in a uniform capillary tube of length L. As measured by a ruler. The temperature of the tube and thread of liquid is raised by ΔT. If γ be the coefficient of volume expansion of the liquid and α be the coefficient of linear expansion of the material of the tube, then the increase ΔL in the length of the thread, again measured by the ruler will be
View SolutionLiquids have no definite volume.
View Solution