(a) The brass scale of a barometer gives correct reading at 0∘C . Coefficient of linear expansion of brass is 2.0×10−5/∘C . The barometer reads 75cm at 27∘C . What is the atmospheric pressure at 27∘C ?
(b) A barometer reads 75.0cm on a steel scale. The room temperature is 30∘C . The scale is correctly graduated for 0∘C . The coefficient of linear expansion of steel is α=1.2×10−5/∘C and the coefficient of volume expansion of mercury is γ=1.8×10−4/∘C . Find the correct atmospheric pressure.
The correct Answer is:75.0405cm
To solve the problem step by step, we will break it down into two parts as given in the question.
Part (a)
1. Identify the given values:
- Coefficient of linear expansion of brass, α=2.0×10−5∘C−1
- Initial temperature, T1=0∘C
- Final temperature, T2=27∘C
- Barometer reading at T2, L+ΔL=75cm
2. Calculate the change in temperature:
ΔT=T2−T1=27∘C−0∘C=27∘C
3. Calculate the change in length due to thermal expansion:
ΔL=αLΔT
Since we need to find L, we can express it as:
L+ΔL=75cm⟹L+αLΔT=75cm
4. Factor out L:
L(1+αΔT)=75cm
5. Solve for L:
L=75cm1+αΔT
Substituting the values:
L=75cm1+(2.0×10−5)×27
L=75cm1+0.00054=75cm1.00054≈74.95cm
6. Convert the length to atmospheric pressure:
Atmospheric pressure P in atm is given by:
P=L×100760
Thus,
P=74.95×100760≈0.9861atm
Part (b)
1. Identify the given values:
- Coefficient of linear expansion of steel, α=1.2×10−5∘C−1
- Coefficient of volume expansion of mercury, γ=1.8×10−4∘C−1
- Initial temperature, T1=0∘C
- Final temperature, T2=30∘C
- Barometer reading at T2, L+ΔL=75.0cm
2. Calculate the change in temperature:
ΔT=T2−T1=30∘C−0∘C=30∘C
3. Calculate the change in length due to thermal expansion:
ΔL=αLΔT
Again, we express it as:
L+ΔL=75.0cm⟹L+αLΔT=75.0cm
4. Factor out L:
L(1+αΔT)=75.0cm
5. Solve for L:
L=75.0cm1+αΔT
Substituting the values:
L=75.0cm1+(1.2×10−5)×30
L=75.0cm1+0.00036=75.0cm1.00036≈74.973cm
6. Calculate the change in volume of mercury:
The change in height of mercury due to volume expansion is given by:
L1+ΔL1=L+ΔL1
Where ΔL1=γ3L1ΔT.
7. Express the equation:
L1(1+γ3ΔT)=74.973cm
8. Solve for L1:
L1=74.973cm1+1.8×10−43×30
L1=74.973cm1+0.0018=74.973cm1.0018≈74.838cm
9. Convert the length to atmospheric pressure:
P=L1×100760
Thus,
P=74.838×100760≈0.98478atm
- Coefficient of linear expansion of brass, α=2.0×10−5∘C−1
- Initial temperature, T1=0∘C
- Final temperature, T2=27∘C
- Barometer reading at T2, L+ΔL=75cm
ΔT=T2−T1=27∘C−0∘C=27∘C
ΔL=αLΔT
Since we need to find L, we can express it as:
L+ΔL=75cm⟹L+αLΔT=75cm
L(1+αΔT)=75cm
L=75cm1+αΔT
Substituting the values:
L=75cm1+(2.0×10−5)×27
L=75cm1+0.00054=75cm1.00054≈74.95cm
Atmospheric pressure P in atm is given by:
P=L×100760
Thus,
P=74.95×100760≈0.9861atm
- Coefficient of linear expansion of steel, α=1.2×10−5∘C−1
- Coefficient of volume expansion of mercury, γ=1.8×10−4∘C−1
- Initial temperature, T1=0∘C
- Final temperature, T2=30∘C
- Barometer reading at T2, L+ΔL=75.0cm
ΔT=T2−T1=30∘C−0∘C=30∘C
ΔL=αLΔT
Again, we express it as:
L+ΔL=75.0cm⟹L+αLΔT=75.0cm
L(1+αΔT)=75.0cm
L=75.0cm1+αΔT
Substituting the values:
L=75.0cm1+(1.2×10−5)×30
L=75.0cm1+0.00036=75.0cm1.00036≈74.973cm
The change in height of mercury due to volume expansion is given by:
L1+ΔL1=L+ΔL1
Where ΔL1=γ3L1ΔT.
L1(1+γ3ΔT)=74.973cm
L1=74.973cm1+1.8×10−43×30
L1=74.973cm1+0.0018=74.973cm1.0018≈74.838cm
P=L1×100760
Thus,
P=74.838×100760≈0.98478atm
Topper's Solved these Questions
Similar Questions
A barometer reads 75.0 cm on a steel scale. The room temperature is 30oC. The scale is correctly graduated for 0C. The coefficient of linear expansion of steel is α=1.2×10−5C−1 and the coefficient of volume expansion of mercury isσ=1.8×10_4C−1. ? Find the correct atmospheric pressure.
View SolutionThe coefficient of linear expansion of gold is 14×10−6/∘C. Find the coefficient of linear expansion on Fahrenheit scale.
View Solution
Knowledge Check
The volume of a metal sphere increases by 0.24% when its temperature is raised by 40∘C . The coefficient of linear expansion of the metal is .......... .∘C
A2×105.∘C−1B6×10−5.∘C−1C18×10−5.∘C−1D1.2×10−5.∘C−1
A glass vessel of volume 100cm3 is filled with mercury and is heated from 25∘C→75∘C. What volume of mercury will overflow? Coefficient of linear expansion of glass =1.8×10−6/'∘C and coefficeient of volume expansion of mercury is 1.8×10−4/'∘C.
View SolutionA barometer with a brass scale reads 755 mm on a day when the temperatures is 25∘C. If the scale is correctly graduated at 0∘C, find the true pressure at 0∘C (interms of height of Hg) given that the coefficient of linear expansion of brass is 18×10−6/K. Coefficient of cubical expansion of mercury=182×10−6/K.
View Solution
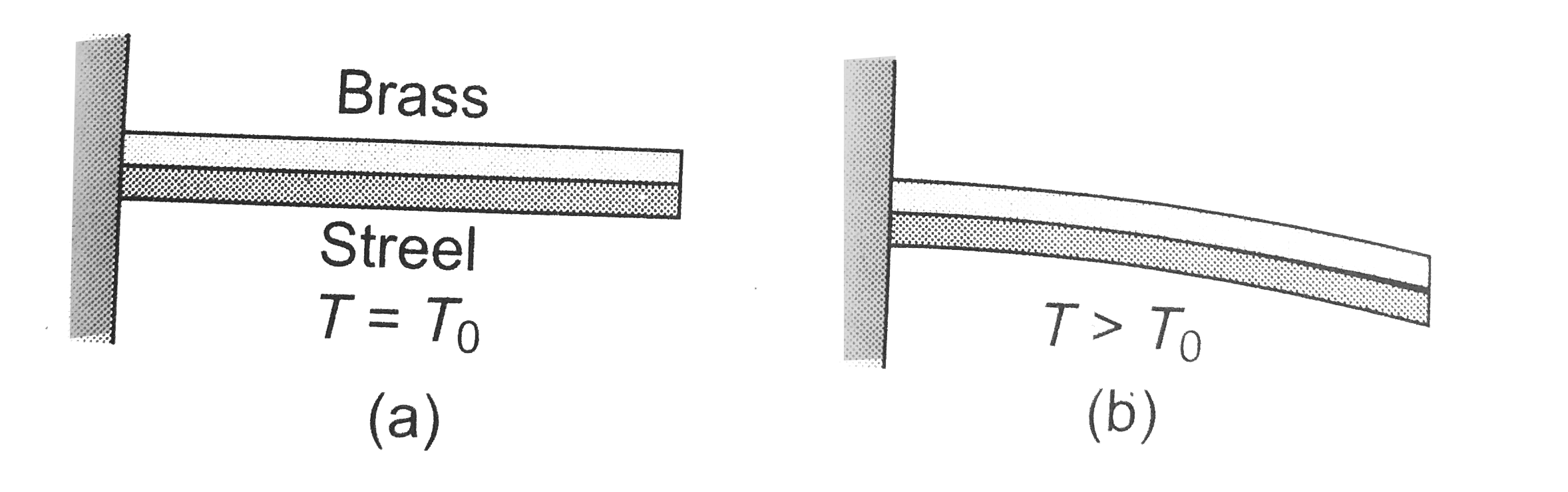
In figure which strip (brass or steel) has higher coefficient of linear expansion.
View SolutionA metallic bar is heated from 0∘C to 100∘C. The coefficient of linear expansion is 10−5K−1. What will be the percentage increase in length
View SolutionIn figure which strip brass or steel have higher coefficient of linear expansion.
View SolutionThe real coefficient of volume expansion of glycerine is 0.000597per∘C and linear coefficient of expansion of glass is 0.000009par∘C . Then the apparent volume coefficient of glycerine is
View SolutionThe real coefficient of volume expansion of glycerine is 0.000597per∘C and linear coefficient of expansion of glass is 0.000009par∘C . Then the apparent volume coefficient of glycerine is
View Solution