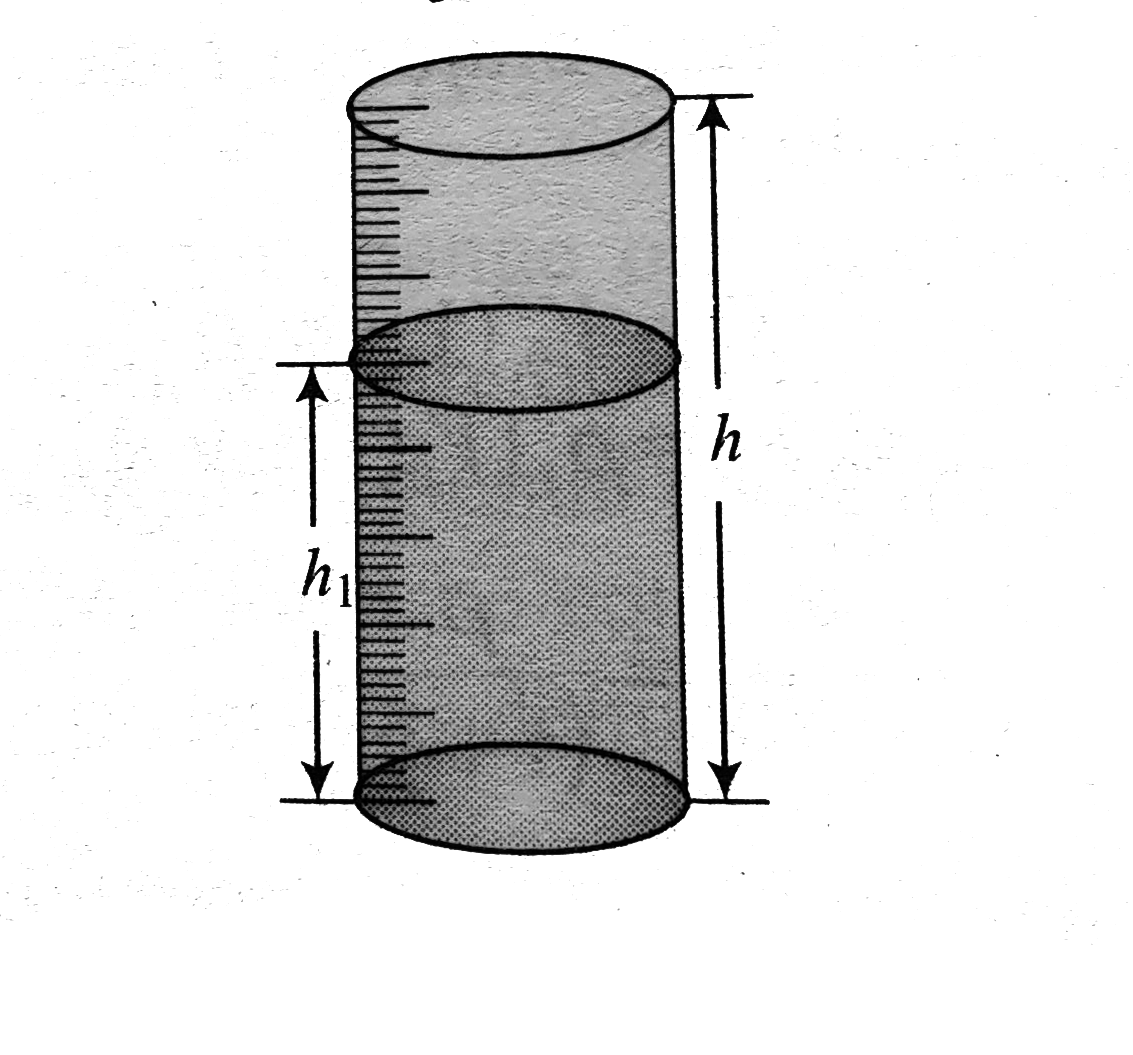
Consider a cylindrical container of cross-section area A length h and having coefficient of linear expansion αc. The container is filled by liquid of real expansion coefficient γL up to height h1. When temperature of the system is increased by Δθ then
(a). Find out the height, area and volume of cylindrical container and new volume of liquid.
(b). Find the height of liquid level when expansion of container is neglected.
(c). Find the relation between γL and αc for which volume of container above the liquid level
(i) increases
(ii). decreases
(iii). remains constant.
(d). On the surface of a cylindrical container a scale is attached for the measurement of level of liquid of liquid filled inside it. If we increase the temperature of the temperature of the system by Δθ, then
(i). Find height of liquid level as shown by the scale on the vessel. Neglect expansion of liquid.
(ii). Find the height of liquid level as shown by the scale on the vessel. Neglect expansion of container.
The correct Answer is:(a) hf=h{1+αcΔθ}
Af=A{1+2αcΔθ}
Vf=Ah{1+3αcΔθ}
volume of liquid Vw=Ah1(1+γLΔθ)
(b) hf=h1{1+γLΔθ}
(c ) (i) 3hαa>h1γl
(ii) 3hαc<h1γl
(iii) 3hαc=h1γl
(d) ΔV=Ah(γL−3αc)Δθ
(i) hf=h1(1−3αcΔθ)
(ii) hf=h1(1+γLΔθ)
(iii) (1)γL>2αc
(2) γL<2αc
(3) γL=2αc
(a) hf=h{1+αcΔθ}
Af=A{1+2αcΔθ}
vf=Ah{1+3αcΔθ}
volume of liquid Vw=Ah1(1+γLΔθ)
(b) hf=h1{1+γLΔθ}
(c ) (i) 3hαc>h1γl (ii) 3hαc<h1γl (iii) 3hαc=h1γl
(d) ΔV=Ah(γL−3αc)Δθ
(e) (i) hf=h1(1−3αcΔθ) (ii) hf=h1(1+γLΔθ)
(iii) (1)γL>2αc(2)γL<2αc(3)γL=2αc
Topper's Solved these Questions
Similar Questions
On heating a liquid having coefficient of volume expension α in a container having coefficient of linear expansion α/2, the level of the liquid in the container would
View SolutionA liquid with coefficient of volume expansion γ is filled in a container of a material having coefficient of linear expansion α . If the liquid overflows on heating, then
View Solution
Knowledge Check
- Question 1 - Select One
A long cylindrical metal vessel of volume V and coefficient of linear expansion α contains a liquid. The level of liquid has not changed on heating. The coefficient of real expansion of the liquid is.
AV−αVBV+αVCVV−αD3α - Question 2 - Select One
On heating a liquid having coefficient of volume expension α in a container having coefficient of linear expansion α/2, the level of the liquid in the container would
AriseBfallCremains almost stationaryDCannot be bredicted - Question 3 - Select One
On heating a liquid of coefficient of cubical expansion γ in a container having coefficient of linear expansion γ/3. The level of liquid in the container will
AriseBfallCwill remain almost stationaryDit is difficult to say
A liquid with coefficient of volume expansion γ is filled in a container of a material having coefficient of linear expansion α . If the liquid overflows on heating, then
View SolutionA solid cube is first floating in a liquid. The coefficient of linear expansion of cube is α and the coefficient of volume expansion of liquid is γ. On increasing the temperature of (liquid + cube) system, the cube will sink if
 View Solution
View SolutionA solid cube is first floating in a liquid. The coefficient of linear expansion of cube is α and the coefficient of volume expansion of liquid is γ. On increasing the temperature of (liquid + cube) system, the cube will sink if
 View Solution
View SolutionA liquid with coefficient of volume expansion γ is filled in a container of a material having coefficient of linear expansion α . If the liquid overflows on heating, then
View SolutionA solid with coefficient of linear expansion aplha just floats in a liquid whose coefficient of volume expansion is γ . If the system is heated, the solid will
View Solution

