A solid aluminium sphere and a solid copper sphere of twice the radius are heated to the same temperature and are allowed to cool under identical surrounding temperatures. Assume that the emisssivity of both the spheres is the same. Find ratio of (a) the rate of heat loss from the aluminium sphere to the rate of heat loss from the copper sphere and (b) the rate of fall of temperature of the aluminium sphere to the rate of fall of temperature of copper sphere. The specific heat capacity of aluminium $= 900 J k g^{- 1} C^{- 1}$ . and that of copper $= 390 J k g^{- 1} C^{- 1}$ . The density of copper $= 3.4$ times the density of aluminium.

Video Solution

Text Solution

Verified by Experts

The correct Answer is:(a) $1 : 4$
(b) $2.9 : 1$

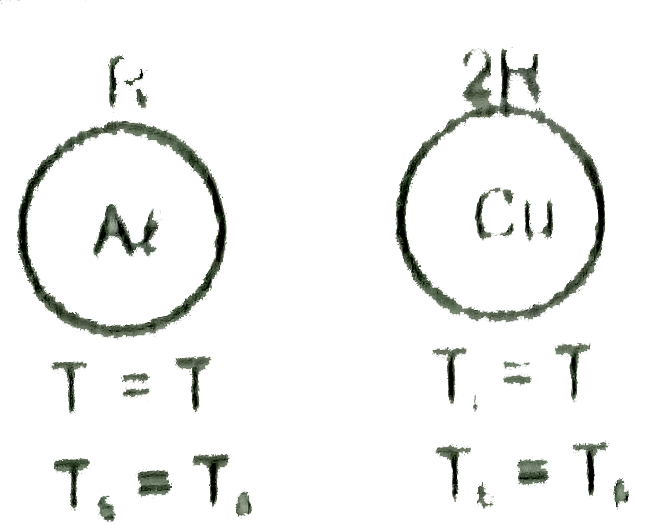
$C$ -cooling rate $H -$ heat loss
$\frac{H_{A l}}{H_{C u}} = \frac{σ A_{A I} (T_{A I^{4} - T_{0}^{4}}^{4})}{σ e A_{C u} (T_{C u^{4} - T_{0}^{4}}^{4})}$
at $t = 0 T_{A l} = T_{C u} = T = \frac{A_{A l}}{A_{C u}} = \frac{R^{2}}{{(2 R)}^{2}} = \frac{1}{4}$
(b) $C -$ cooling rate
$\frac{C_{A l}}{C_{C u}} = (\frac{A_{A l}}{m_{A l} S_{A l}}) (\frac{m_{C u} S_{C u}}{A_{C u}})$
$= (\frac{A_{A l}}{A_{C u}}) (\frac{m_{C u}}{m_{A l}}) (\frac{S_{C u}}{S_{A l}})$
$= {(\frac{R}{2 R})}^{2} \times {{(\frac{2 R}{R})}^{3} \times 3.4} \times \frac{390}{900} = 2.9$

|

Updated on:21/07/2023

Class 11 PHYSICS THERMAL EXPANSION AND CALORIMETRY

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A solid sphere and a hollow sphere of same material and size are heated to same temperature and allowed to cool in the same surroundings. If the temperature difference between each sphere and its surrounding is T, then
Athe hollow sphere will cool at a faster rate for all, values of T
Bthe solid sphere will cool at a faster rate for all value of T
Cboth spheres will cool at the same rate for all values of T
Dboth spheres will cool at the same rate only for small value of T.

RESONANCE ENGLISH-HEAT TRANSFER-Advancel Level Problems

Home
Ask Doubt
Profile