A uniform cylinder of length L and thermal conductivity k is placed on a metal plate of the same area S of mass m and infinite conductivity. The specific heat of the plate is c. The top of the cylinder is maintained at T0. Find the time required for the temperature of the plate to rise from T1 to T2(T1<T2<T0).
The correct Answer is:mCLKSln(T0−T1T0−T2)
To solve the problem step by step, we will analyze the heat transfer from the cylinder to the metal plate and derive the time required for the temperature of the plate to rise from T1 to T2.
Step 1: Understanding the System
We have a uniform cylinder of length L and thermal conductivity k placed on a metal plate with mass m, area S, and specific heat c. The top of the cylinder is maintained at temperature T0, and we want to find the time required for the plate's temperature to rise from T1 to T2.
Step 2: Heat Transfer Equation
The rate of heat transfer dqdt through the cylinder can be expressed using Fourier's law of heat conduction:
dqdt=kA(T0−T)L
where T is the temperature of the plate at any time t.
Step 3: Relating Heat Transfer to Temperature Change
The heat absorbed by the metal plate can also be expressed in terms of its mass and specific heat:
dqdt=mcdTdt
where T is the temperature of the plate.
Step 4: Setting Up the Equation
Equating the two expressions for dqdt:
mcdTdt=kS(T0−T)L
Step 5: Rearranging the Equation
Rearranging gives us:
dTT0−T=kSmcLdt
Step 6: Integrating Both Sides
Now we will integrate both sides. The left side will be integrated from T1 to T2, and the right side from 0 to t:
∫T2T1dTT0−T=kSmcL∫t0dt
Step 7: Performing the Integration
The left side integrates to:
−ln(T0−T)∣∣∣T2T1=−ln(T0−T2)+ln(T0−T1)=ln(T0−T1T0−T2)
The right side integrates to:
kSmcLt
Step 8: Setting the Equations Equal
Setting both integrals equal gives us:
ln(T0−T1T0−T2)=kSmcLt
Step 9: Solving for Time t
Now, we can solve for t:
t=mcLkSln(T0−T1T0−T2)
Final Result
Thus, the time required for the temperature of the plate to rise from T1 to T2 is:
t=mcLkSln(T0−T1T0−T2)
We have a uniform cylinder of length L and thermal conductivity k placed on a metal plate with mass m, area S, and specific heat c. The top of the cylinder is maintained at temperature T0, and we want to find the time required for the plate's temperature to rise from T1 to T2.
The rate of heat transfer dqdt through the cylinder can be expressed using Fourier's law of heat conduction:
dqdt=kA(T0−T)L
where T is the temperature of the plate at any time t.
The heat absorbed by the metal plate can also be expressed in terms of its mass and specific heat:
dqdt=mcdTdt
where T is the temperature of the plate.
Equating the two expressions for dqdt:
mcdTdt=kS(T0−T)L
Rearranging gives us:
dTT0−T=kSmcLdt
Now we will integrate both sides. The left side will be integrated from T1 to T2, and the right side from 0 to t:
∫T2T1dTT0−T=kSmcL∫t0dt
The left side integrates to:
−ln(T0−T)∣∣∣T2T1=−ln(T0−T2)+ln(T0−T1)=ln(T0−T1T0−T2)
The right side integrates to:
kSmcLt
Setting both integrals equal gives us:
ln(T0−T1T0−T2)=kSmcLt
Now, we can solve for t:
t=mcLkSln(T0−T1T0−T2)
Thus, the time required for the temperature of the plate to rise from T1 to T2 is:
t=mcLkSln(T0−T1T0−T2)
Topper's Solved these Questions
Similar Questions
The amount of heat energy required to raise the temperature of 1 g of Helium at NTP, from T1 K to T2 K is :
View SolutionA solid sphere of radius 'R' density ρ and specific heat 'S' initially at temperature T0 Kelvin is suspended in a surrounding at temperature 0K. Then the time required to decrease the temperature of the sphere from T0 to T02 kelvin is (Assume sphere behaves like a black body)
View SolutionTwo plates of equal area are placed in contact with each other. The thickness of the plates are 2.0 cm and 3.0 cm respectively. The outer face of first plate is at −25∘C and that of second plate is at +25∘C. The conductivities of the plates are in the ratio 2:3. Calculate the temperature of the common surface of the plates.
View SolutionGibbs-Helmoholtz equation relates the free energy change to the enthalpy and entropy changes of the process as
(ΔG)PT=ΔH−TΔS
The magnitude of ΔH does not change much with the change in temperature but the energy factor TΔS changes appreciably. Thus, spontaneity of a process depends very much on temperature.
The dissolution of CaCl2.6H2O in a large volume of water is endothermic to the extent of 3.5kcalmol−1. For the reaction. CaCl2(s)+6H2O(l)→CaCl2.6H2O(s)ΔHis−23.2kcal. The heat of solution of anhydrous CaCI2 in large quantity of water will beView SolutionThree rods of same cross-section but different length and conductivity are joined in series . If the temperature of the two extreme ends are T1andT2(T1>T2) find the rate of heat transfer H.
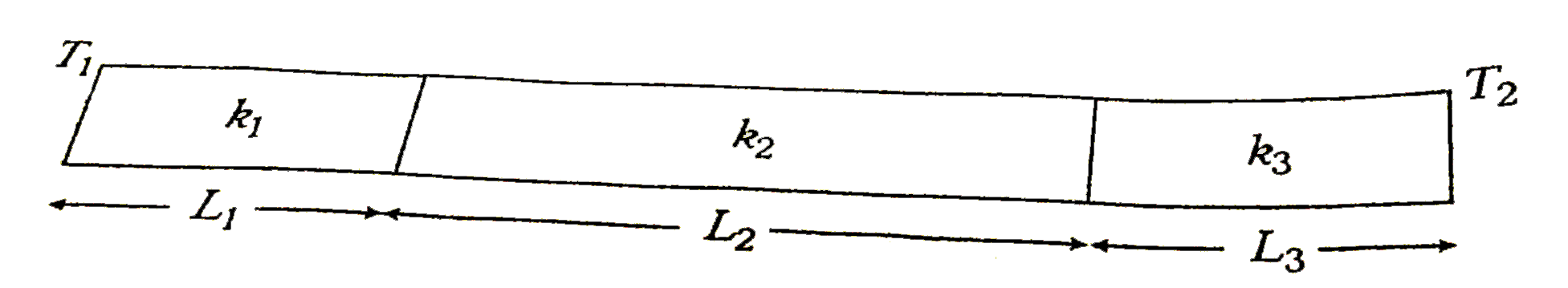 View Solution
View SolutionThe specific heat of a metal at low temperature varies according to S=(4/5)T3 where T is the absolute temperature. Find the heat energy needed to raise unit mass of the metal from T = 1 K →T= 2K.
View SolutionA liquid of mass m and specific heat c is heated to a temperature 2T. Another liquid of mass m/2 and specific heat 2 c is heated to a temperature T. If these two liquids are mixed, the resulting temperature of the mixture is
View SolutionA rod of length l with thermally insulated lateral surface consists of material whose heat conductivity coefficient varies with temperature as k=a/T, where a is a constant. The ends of the rod are kept at temperatures T1andT2. Find the function T(x), where x is the distance from the end whose temperature is T1.
View SolutionA rod of length l and cross sectional area A has a variable conductivity given by K=αT, where α is a positive constant T is temperatures in Kelvin. Two ends of the rod are maintained at temperatures T1 and T2(T1>T2). Heat current flowing through the rod will be
View SolutionThree rods of equal length, area of cross section and thermal conductivity are connected as shown in the figure.
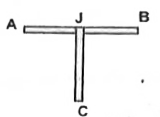
There is no heat loss through lateral surface of the rods and temperature of ends are TA=100∘C,TB=70∘C and TC=50∘C. Find the temperature of junction.View Solution

