The square of the angular velcoity ω of a certain wheel increases linearly with the angular displacement during 100rev of the wheel's motion as shown. Compute the time t required for the increase.

The correct Answer is:40π7sec.
We have Δθ=2π×(100rev)=200πrad
So ω2=ω20+2αΔθ
⇒1600=900+2α×200π
⇒α=700400π=74π
Also ω=ω0+αt
⇒40=30+74πt
⇒t=40π7
Topper's Solved these Questions
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 484videos
DAILY PRACTICE PROBLEMS
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 496videos
DAILY PRACTICE PROBLEMS
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 466videos
DAILY PRACTICE PROBLEMS
- BOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Exercise53videos
CURRENT ELECTRICITY
- BOOK - RESONANCE ENGLISHCHAPTER - ELASTICITY AND VISCOCITYEXERCISE - Advanced Level Problems9videos
ELASTICITY AND VISCOCITY
Similar Questions
The angular velocity of a wheel increases from 100 to 300 in 10 s. The number of revolutions made during that time is
View SolutionA wheel is a rest. Its angular velocity increases uniformly and becomes 80rads−1 after 5 s. The total angular displacement is
View SolutionA wheel is at rest. Its angular velocity increases uniformly and becomes 80 radian per second after 5 second. The total angular displacement is :-
View SolutionThe angular velocity of a gar is controlled according to ω=12−3t2 where ω in radian per second, is positive in the clockwise sense and t is the time in seconds. Find the net angular displacement △θ from the time t=0 to t=3s. Also, find the number of revolutions N through which the gear turns during the 3s.
View SolutionA wheel having moment of inertia 2π2 kgm2 is at rest. It is rotated by a 60 W ideal motor for one minute. The angular displacement traversed by the wheel in one minute is
View Solution
The assembly of two discs as shown in figure is placed on a rough horizontal surface and the front disc is given an initial angular velocity ω0. Determine the final linear and angular velocity when both the discs start rolling. it is given that friction is sufficient to sustain rolling the rear wheel from the starting of motion.View SolutionA ring of radius R is made of a thin wire of material of density ρ having cross section area a. The ring rotates with angular velocity ω about an axis passing through its centre and perpendicular to the plane. If we consider a small element of the ring,it rotates in a circle. The required centripetal force is provided by the component of tensions on the element towards the centre. A small element of length dl of angular width dθ is shown in the figure.

If for a given mass of the ring and angular velocity, the radius R of the ring is increased to 2R, the new tension will beView SolutionA ring of radius R is made of a thin wire of material of density ρ having cross section area a. The ring rotates with angular velocity ω about an axis passing through its centre and perpendicular to the plane. If we consider a small element of the ring,it rotates in a circle. The required centripetal force is provided by the component of tensions on the element towards the centre. A small element of length dl of angular width dθ is shown in the figure.
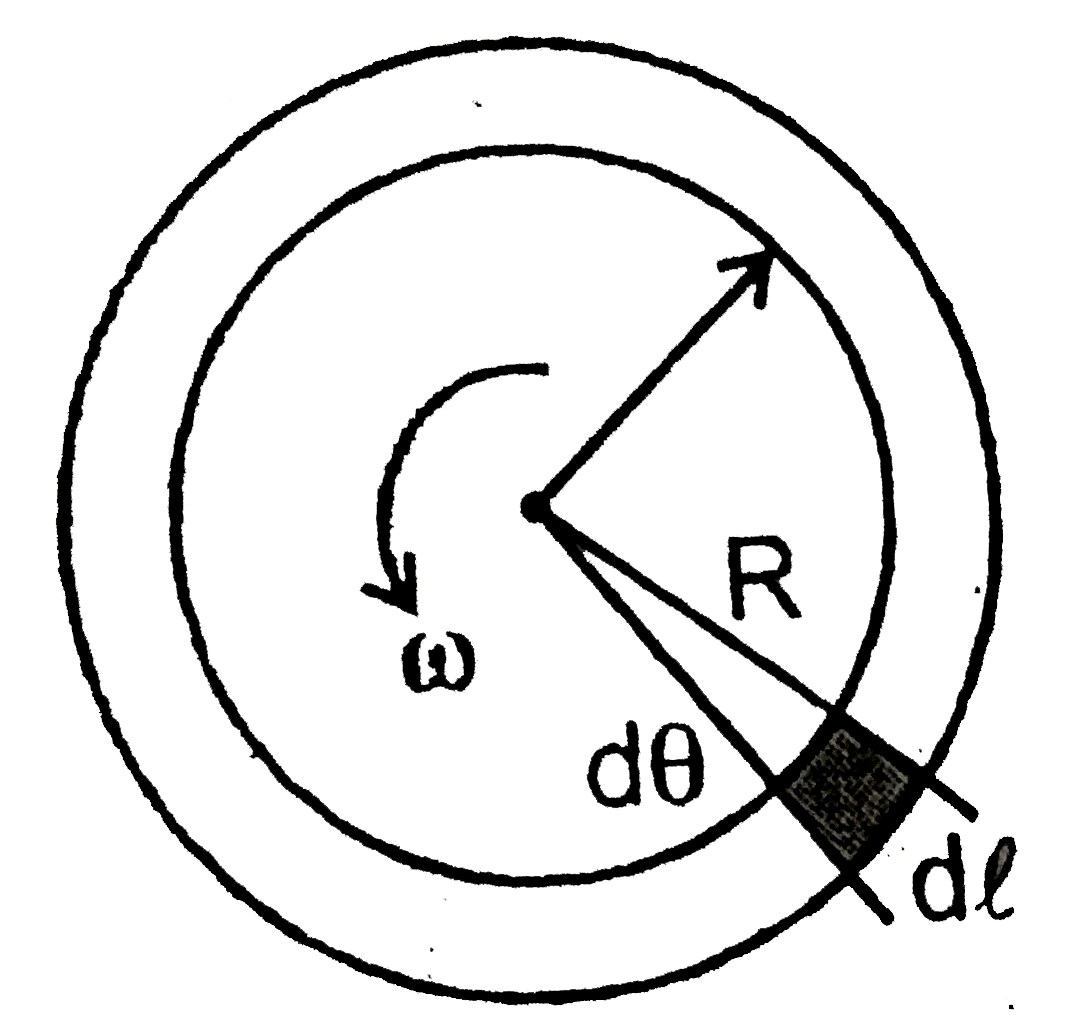
If T is the tension in the ring, thenView SolutionThe magnitude of displacement of a particle moving in a circle of radius a with constant angular speed ω varries with time t is
View SolutionThe side of a square is increasing at the rate of 0.2 cm/s. The rate of increase of perimeter w.r.t time is :
View Solution

