A disc is hinged such that it can freely rotate in a vertical plane about a point on its radius. If radius of disc is , then what will be minimum time period of its simple harmonic motion ?
The correct Answer is:C
To find the minimum time period of the simple harmonic motion of a disc hinged at a point on its radius, we will follow these steps:
Step 1: Understand the system
The disc can be treated as a physical pendulum. The time period T of a physical pendulum is given by the formula:
T=2π√Imgx
where:
- I is the moment of inertia of the disc about the hinge point,
- m is the mass of the disc,
- g is the acceleration due to gravity,
- x is the distance from the hinge to the center of mass of the disc.
Step 2: Calculate the moment of inertia
For a disc of radius R and mass m, the moment of inertia about its center is:
Icenter=12mR2
When the disc is hinged at a point on its radius, we can use the parallel axis theorem to find the moment of inertia about the hinge point:
I=Icenter+md2
where d is the distance from the center of the disc to the hinge point. Since the hinge is at a distance R2 from the center (as the hinge is on the radius), we have:
d=R2
Thus,
I=12mR2+m(R2)2=12mR2+14mR2=34mR2
Step 3: Determine the distance to the center of mass
The center of mass of the disc is at a distance of R2 from the hinge point (since the hinge is at the radius). Therefore, we have:
x=R2
Step 4: Substitute values into the time period formula
Now, substituting I and x into the time period formula:
T=2π
⎷34mR2mg⋅R2
This simplifies to:
T=2π
⎷34R2g⋅R2
=2π√3R2g
Step 5: Find the minimum time period
To find the minimum time period, we need to find the condition for x. The minimum time period occurs when x=R√2. Thus, substituting this back into the time period formula:
Tmin=2π
⎷34mR2mg⋅R√2
This simplifies to:
Tmin=2π√3R√22g
Final Result
Thus, the minimum time period of the simple harmonic motion of the disc is:
Tmin=2π√R√2g
The disc can be treated as a physical pendulum. The time period T of a physical pendulum is given by the formula:
T=2π√Imgx
where:
- I is the moment of inertia of the disc about the hinge point,
- m is the mass of the disc,
- g is the acceleration due to gravity,
- x is the distance from the hinge to the center of mass of the disc.
For a disc of radius R and mass m, the moment of inertia about its center is:
Icenter=12mR2
When the disc is hinged at a point on its radius, we can use the parallel axis theorem to find the moment of inertia about the hinge point:
I=Icenter+md2
where d is the distance from the center of the disc to the hinge point. Since the hinge is at a distance R2 from the center (as the hinge is on the radius), we have:
d=R2
Thus,
I=12mR2+m(R2)2=12mR2+14mR2=34mR2
The center of mass of the disc is at a distance of R2 from the hinge point (since the hinge is at the radius). Therefore, we have:
x=R2
Now, substituting I and x into the time period formula:
T=2π
⎷34mR2mg⋅R2
This simplifies to:
T=2π
⎷34R2g⋅R2
=2π√3R2g
To find the minimum time period, we need to find the condition for x. The minimum time period occurs when x=R√2. Thus, substituting this back into the time period formula:
Tmin=2π
⎷34mR2mg⋅R√2
This simplifies to:
Tmin=2π√3R√22g
Thus, the minimum time period of the simple harmonic motion of the disc is:
Tmin=2π√R√2g
Topper's Solved these Questions
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 765videos
DAILY PRACTICE PROBLEMS
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - DPP 778videos
DAILY PRACTICE PROBLEMS
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 747videos
DAILY PRACTICE PROBLEMS
- BOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Exercise53videos
CURRENT ELECTRICITY
- BOOK - RESONANCE ENGLISHCHAPTER - ELASTICITY AND VISCOCITYEXERCISE - Advanced Level Problems9videos
ELASTICITY AND VISCOCITY
Similar Questions
An uniform disc is rotating at a constant speed in a vertical plane about a fixed horizontal axis passing through the centre of the disc. A piece of the disc from its rim detaches itself from the disc at the instant when it is at horizontal level with the centre of the disc and moving upwards, then about the fixed axis.
STATEMENT-1 : Angular speed of the disc about the aixs of rotation will increase.
and
STATEMENT-2 : Moment of inertia of the disc is decreased about the axis of rotation.View SolutionA uniform semicircular ring of mass m and radius r is hinged at end A so that it can rotate freely about end A in the vertical plane as shown in the figure. Calculate angle made by line AB with vertical in equilibrium position and time period of small oscillation of ring.
 View Solution
View SolutionA disc of a mass M and radius R can rotate freely in vertical plane about a horizontal axis at O. Distant r from the center of disc as shown in the figure. The disc is released from rest in the shown position.

The angular acceleration of disc when OC rotates by an angles of 37∘ isView SolutionThe rotation of earth about its axis is
a) Periodic motion
b) Simple harmonic motion
c) Periodic but not simple harmonic motion
d) Non- Periodic motionView SolutionA uniform disc of mass m & radius R is pivoted at its centre O with its plane vertical as shown in figure A circular portion of disc of radius R2 is removed from it. The time period of small oscillations of remaining portion about O is -
 View Solution
View SolutionWhen a body is hinged at a point and a force is acting on the body in such a way that the line of action of force is at some distance from the hinged point, the body will start rotating about the hinged point. The angular acceleration of the body can be calculated by finding the torque of that force about the hinged point. A disc of mass m and radius R is hinged at point A at its bottom and is free to rotate in the vertical plane. A force of magnitude F is acting on the ring at top most point.
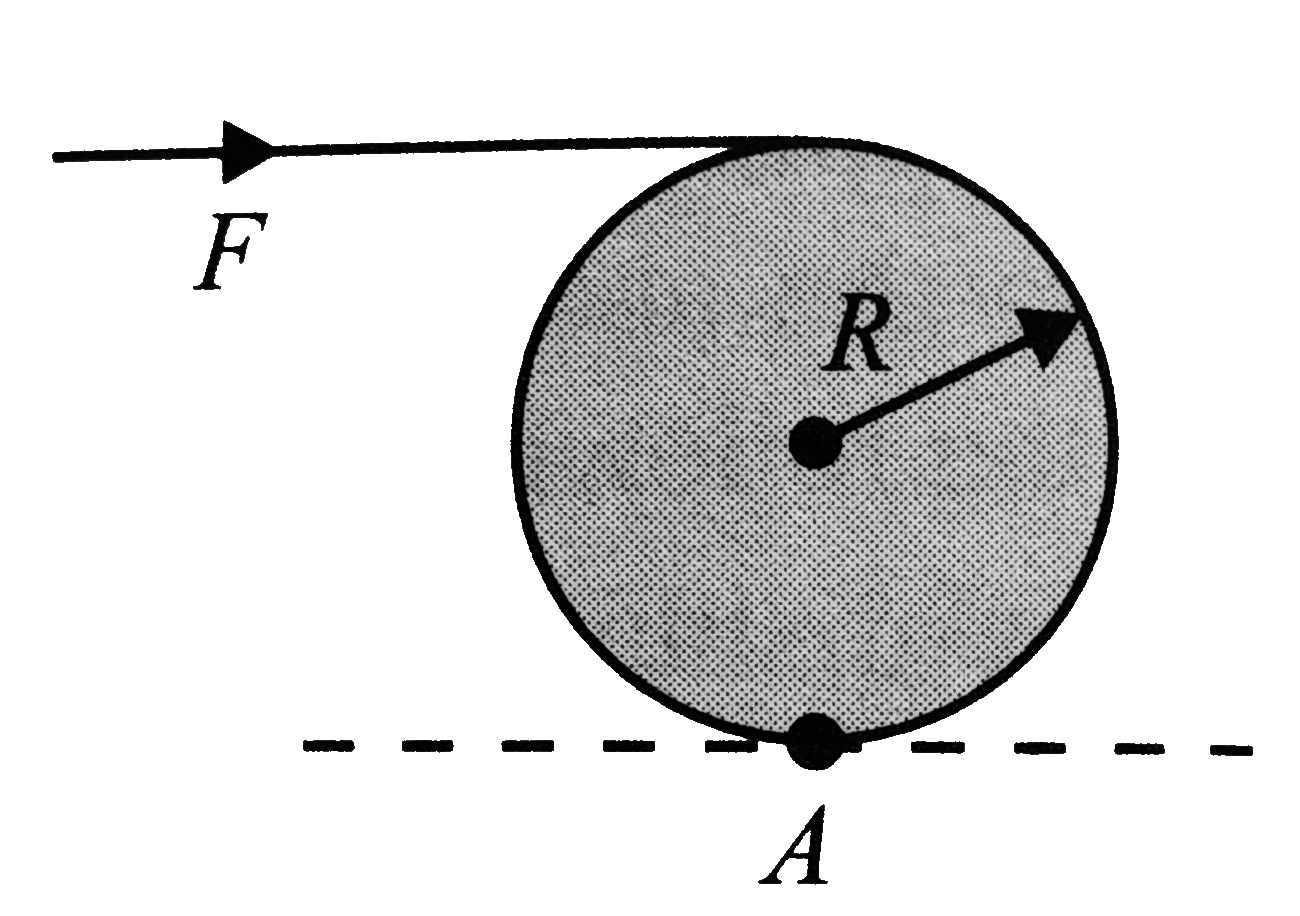
Component of reaction at the hinge in the horizontal direction isView SolutionWhen a body is hinged at a point and a force is acting on the body in such a way that the line of action of force is at some distance from the hinged point, the body will start rotating about the hinged point. The angular acceleration of the body can be calculated by finding the torque of that force about the hinged point. A disc of mass m and radius R is hinged at point A at its bottom and is free to rotate in the vertical plane. A force of magnitude F is acting on the ring at top most point.
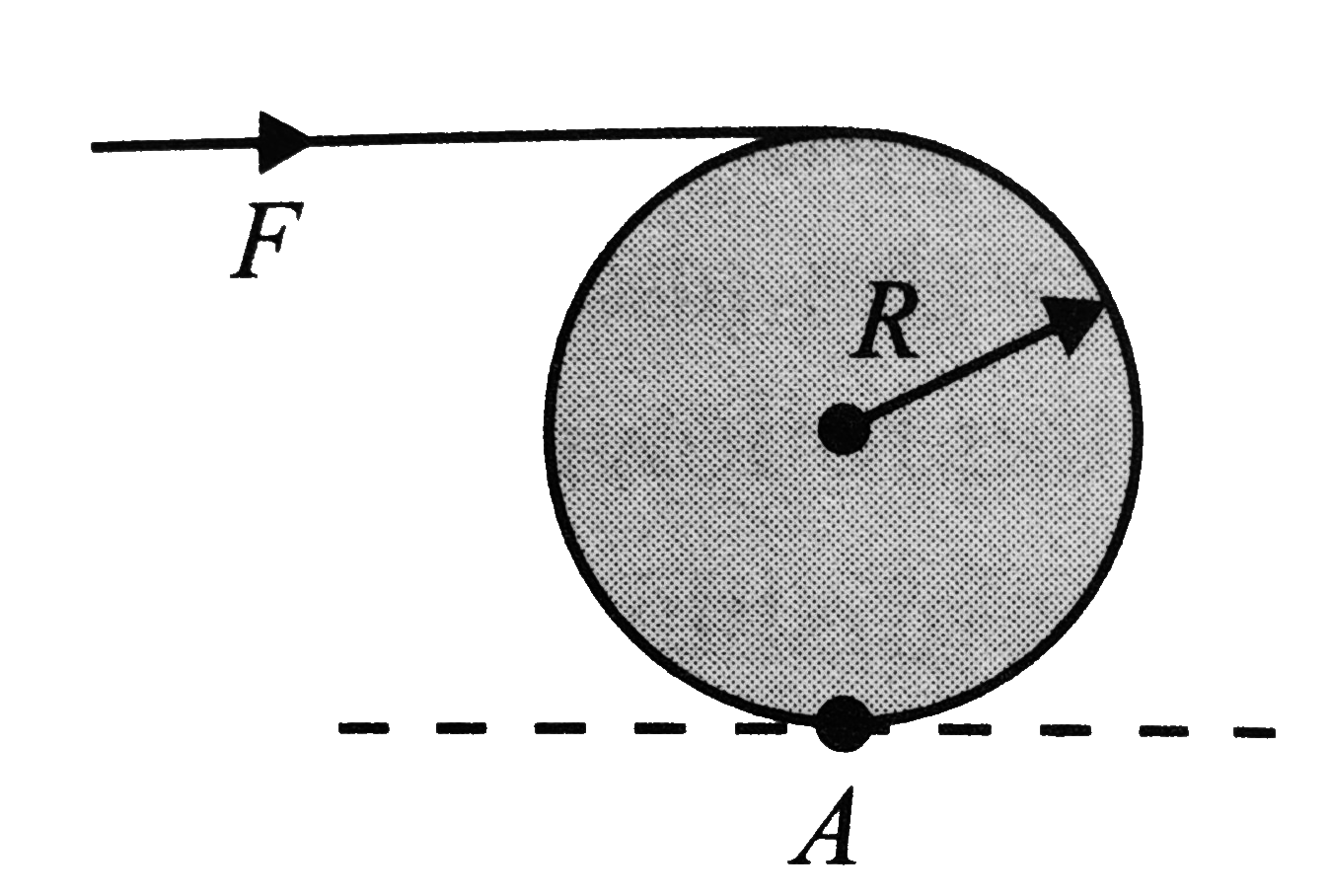
Tangential acceleration of the centre of mass isView SolutionWhen a body is hinged at a point and a force is acting on the body in such a way that the line of action of force is at some distance from the hinged point, the body will start rotating about the hinged point. The angular acceleration of the body can be calculated by finding the torque of that force about the hinged point. A disc of mass m and radius R is hinged at point A at its bottom and is free to rotate in the vertical plane. A force of magnitude F is acting on the ring at top most point.
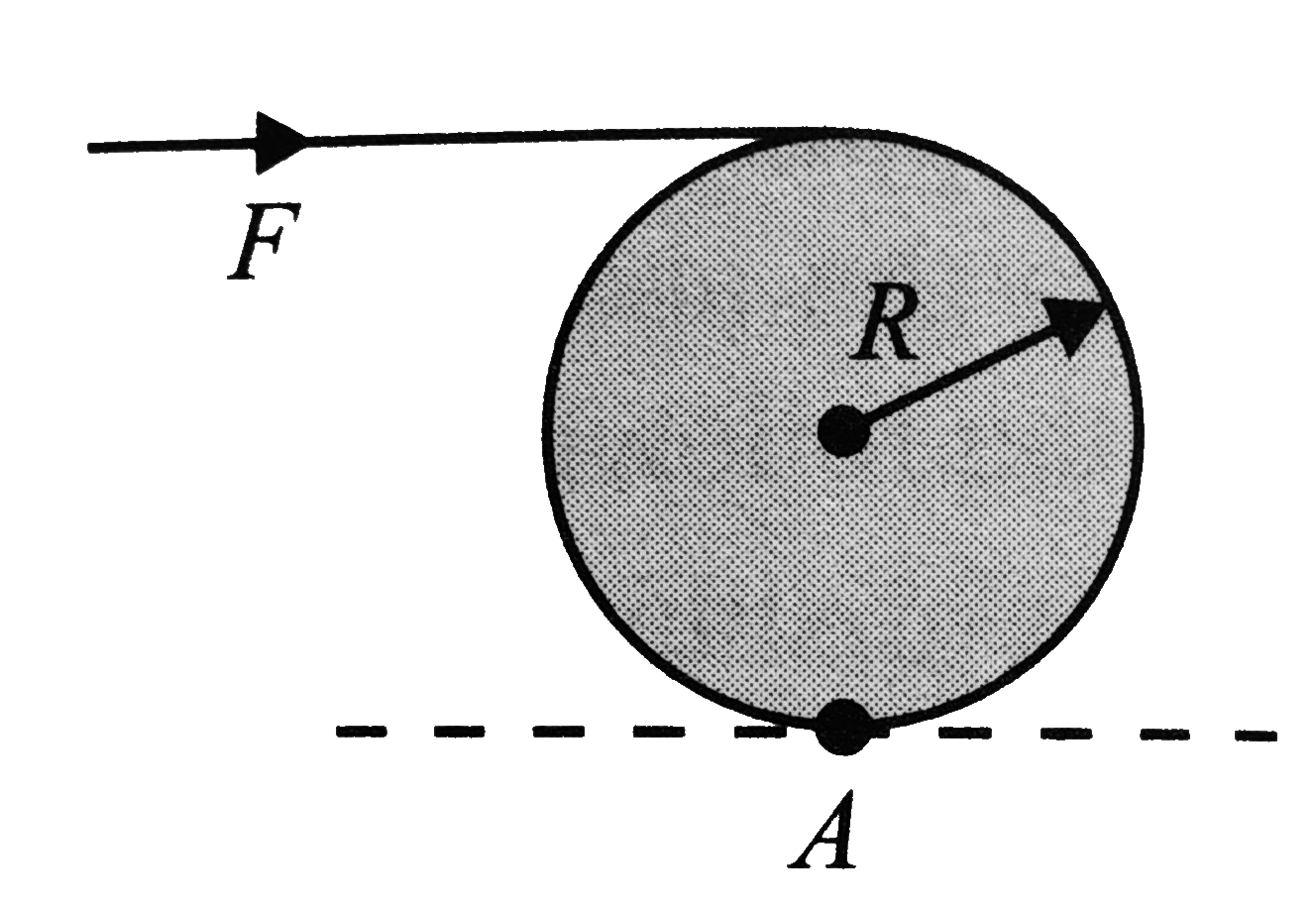
Component of reaction at the hinge in the vertical direction isView SolutionFor a disc of given density and thickness, its moment of inertia varies with radius of the disc as :
View SolutionA uniform disc is rotating at a constantt speed in a vertical plane about a fixed horizontal axis passing through the centre of the disc. A piece of the disc from its rim detaches itself from the disc at the instant when it is at horizontal level with the centre of the disc and moving upward. Then about the fixed axis, the angular speed of the
View Solution

