For a disc of given density and thickness, its moment of inertia varies with radius of the disc as :
1∝R2
1∝R4
1∝R3
1∝R
The correct Answer is:B
To find how the moment of inertia of a disc varies with its radius, we can follow these steps:
1. Define the Variables:
- Let the density of the disc be ρ.
- Let the thickness of the disc be d.
- Let the radius of the disc be R.
2. Consider an Elemental Ring:
- Take an elemental ring at a distance r from the center of the disc with a thickness dr.
- The circumference of this ring is 2πr.
3. Calculate the Mass of the Elemental Ring:
- The mass dm of the elemental ring can be expressed as:
dm=ρ⋅d⋅(2πr⋅dr)
- Here, ρ⋅d is the volume density times the thickness, giving the mass per unit area.
4. Moment of Inertia of the Elemental Ring:
- The moment of inertia dI of the elemental ring about the axis of rotation (which is through the center of the disc) is given by:
dI=r2⋅dm=r2⋅(ρ⋅d⋅2πr⋅dr)
- Simplifying this, we have:
dI=ρ⋅d⋅2πr3⋅dr
5. Integrate to Find the Total Moment of Inertia:
- To find the total moment of inertia I of the disc, integrate dI from r=0 to r=R:
I=∫R0dI=∫R0ρ⋅d⋅2πr3⋅dr
- Since ρ, d, and 2π are constants, they can be taken out of the integral:
I=ρ⋅d⋅2π∫R0r3⋅dr
6. Calculate the Integral:
- The integral ∫R0r3⋅dr can be calculated as:
∫R0r3⋅dr=(r44)R0=R44
7. Substitute Back to Find I:
- Substituting the result of the integral back into the equation for I:
I=ρ⋅d⋅2π⋅R44
- This simplifies to:
I=ρ⋅d⋅π2R4
8. Conclusion:
- Thus, the moment of inertia I of the disc varies with the radius R as:
I∝R4
Final Answer:
The moment of inertia of a disc of given density and thickness varies with the radius R as I∝R4.
- Let the density of the disc be ρ.
- Let the thickness of the disc be d.
- Let the radius of the disc be R.
- Take an elemental ring at a distance r from the center of the disc with a thickness dr.
- The circumference of this ring is 2πr.
- The mass dm of the elemental ring can be expressed as:
dm=ρ⋅d⋅(2πr⋅dr)
- Here, ρ⋅d is the volume density times the thickness, giving the mass per unit area.
- The moment of inertia dI of the elemental ring about the axis of rotation (which is through the center of the disc) is given by:
dI=r2⋅dm=r2⋅(ρ⋅d⋅2πr⋅dr)
- Simplifying this, we have:
dI=ρ⋅d⋅2πr3⋅dr
- To find the total moment of inertia I of the disc, integrate dI from r=0 to r=R:
I=∫R0dI=∫R0ρ⋅d⋅2πr3⋅dr
- Since ρ, d, and 2π are constants, they can be taken out of the integral:
I=ρ⋅d⋅2π∫R0r3⋅dr
- The integral ∫R0r3⋅dr can be calculated as:
∫R0r3⋅dr=(r44)R0=R44
- Substituting the result of the integral back into the equation for I:
I=ρ⋅d⋅2π⋅R44
- This simplifies to:
I=ρ⋅d⋅π2R4
- Thus, the moment of inertia I of the disc varies with the radius R as:
I∝R4
The moment of inertia of a disc of given density and thickness varies with the radius R as I∝R4.
Topper's Solved these Questions
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (GRAVITATION)
25 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Properties of Matter & Fluid Mechanics)(Elasticity)
20 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (COLLISION AND CENTRE OF MASS )
12 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
Similar Questions
A circular disc of radius R and thickness R6 has moment of inertia I about its axis. If it is melted and casted into a solid sphere then what will be its moment of inertia, about its diametric axis?
View SolutionA stationary horizontal disc is free to rotate about its axis. When a torque is applied on it its, kinetic energy as a function of θ, where θ is the angle by which it has rotated, is given as kθ. If its moment of inertia is I then the angular acceleration of the disc is :
View SolutionA circular disc of radius R and thickness R6 has moment of inertia about an axis passing through its centre and perpendicular to its plane. It is melted and recasted into a solid sphere. The moment of inertia of the sphere about its diameter as axis of rotation is
View SolutionWhat is the moment of inertia of a circular disc about one of its diameters ?
View SolutionThe mass of a disc is 700 gm and its radius of gyration is 20 cm. What is its moment of inertia if it rotates about an axis passing through its centre and perpendicular to the face of the disc?
View SolutionMoment of inertia of a disc about an axis parallel to diameter and at a distance x from the centre of the disc is same as the moment of inertia of the disc about its centre axis. The radius of disc is R. The value of x is
View SolutionThe diagram shows a uniform disc of mass M & radius 'a', if the moment of inertia of the disc about the axis XY is I, its moment of inertia about an axis through O and perpendicular to the plane of the disc is

View SolutionA solid sphere of mass M, radius R and having moment of inertia about as axis passing through the centre of mass as I, is recast into a disc of thickness t, whose moment of inertia about an axis passing through its edge and perpendicular to its plance remains I. Then, radius of the disc will be.
View SolutionThe moment of inertia of a uniform flat disc about its own axis is I. The radius of the disc is a A section ABCD of the disc (as shown in figure) is cut off and separated. The moment of inertia of the remainin g part of the disc about the same axis will be
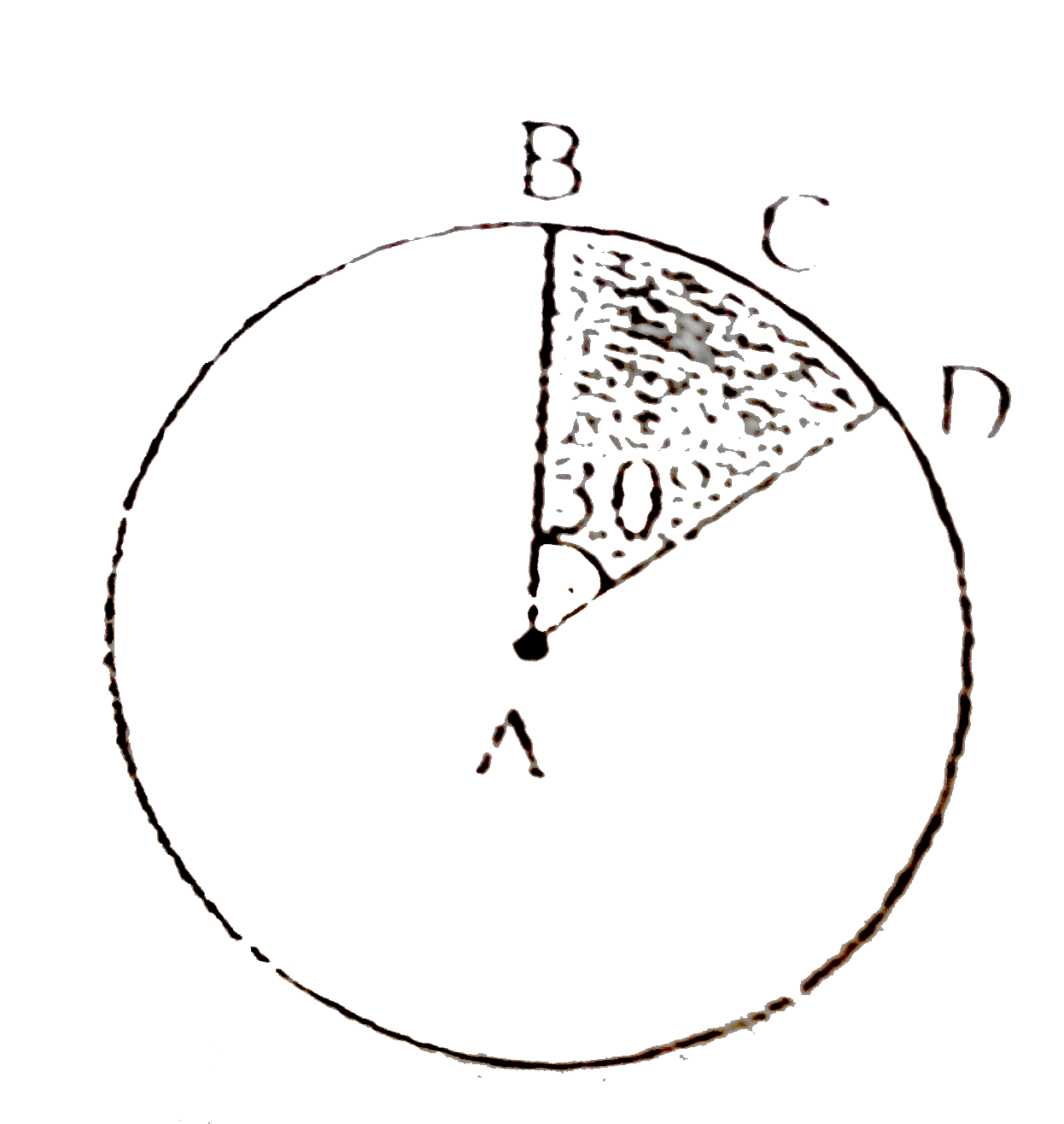
View SolutionA disc with moment of inertia I is rotating with some angular speed. Second disc is initially at rest. Now second disc with moment of inertia 3I is placed on first disc and starts rotating. Find loss of kinetic energy in fraction
View Solution
