A concave mirror of radius of curvature R has a circular outline of radius r. A circular disc is to be placed normal to the axis at the focus so that it collects all the light that is reflected from the mirror from a beam parallel to the axis. For r >>R, the area of this disc has to be at least
πr64R4
πr44R2
πr54R3
πr4R2
The correct Answer is:A
To solve the problem, we need to determine the minimum area of a circular disc that can be placed at the focus of a concave mirror, which collects all the light reflected from the mirror when a beam of light is parallel to the principal axis.
1. Understanding the Geometry of the Concave Mirror:
- The radius of curvature of the concave mirror is R.
- The focal length f of the concave mirror is given by the formula:
f=R2
2. Positioning the Disc:
- The disc is to be placed normal to the axis at the focus of the mirror, which is at a distance f=R2 from the mirror.
3. Ray Diagram:
- Consider rays of light coming parallel to the principal axis. When these rays strike the mirror, they will reflect and converge at the focal point.
- The rays that hit the edge of the mirror will create a cone of light that converges at the focus.
4. Using Similar Triangles:
- Let r be the radius of the circular outline of the mirror.
- The distance from the edge of the mirror to the focal point is R2.
- We can form similar triangles to relate the radius of the disc r0 to the radius of the mirror r.
5. Setting Up the Similar Triangles:
- The triangles formed by the radius of the mirror and the radius of the disc at the focus can be expressed as:
r0r=R2x
- Here, x is the distance from the focal point to the point on the principal axis directly below the edge of the mirror.
6. Expressing x:
- The distance x can be expressed in terms of r and R:
x=r2−R2
7. Substituting and Simplifying:
- Substitute x back into the triangle ratio:
r0=r⋅R2r2−R2
- Rearranging gives:
r0=rRr−R
8. Finding the Area of the Disc:
- The area A of the disc is given by:
A=πr20
- Substituting for r0:
A=π(rRr−R)2
9. Considering the Condition r≫R:
- Since r≫R, we can approximate r−R≈r:
A≈π(rRr)2=πR2
10. Final Result:
- Therefore, the area of the disc must be at least:
A≈πR2
- The radius of curvature of the concave mirror is R.
- The focal length f of the concave mirror is given by the formula:
f=R2
- The disc is to be placed normal to the axis at the focus of the mirror, which is at a distance f=R2 from the mirror.
- Consider rays of light coming parallel to the principal axis. When these rays strike the mirror, they will reflect and converge at the focal point.
- The rays that hit the edge of the mirror will create a cone of light that converges at the focus.
- Let r be the radius of the circular outline of the mirror.
- The distance from the edge of the mirror to the focal point is R2.
- We can form similar triangles to relate the radius of the disc r0 to the radius of the mirror r.
- The triangles formed by the radius of the mirror and the radius of the disc at the focus can be expressed as:
r0r=R2x
- Here, x is the distance from the focal point to the point on the principal axis directly below the edge of the mirror.
- The distance x can be expressed in terms of r and R:
x=r2−R2
- Substitute x back into the triangle ratio:
r0=r⋅R2r2−R2
- Rearranging gives:
r0=rRr−R
- The area A of the disc is given by:
A=πr20
- Substituting for r0:
A=π(rRr−R)2
- Since r≫R, we can approximate r−R≈r:
A≈π(rRr)2=πR2
- Therefore, the area of the disc must be at least:
A≈πR2
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.42
20 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.43
20 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.40
20 Videos
- CURRENT ELECTRICITYBOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)
19 Videos
- ELECTRO MAGNETIC WAVESBOOK - RESONANCE ENGLISHCHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 3
27 Videos
Similar Questions
A circular metallic disc of radius R has a small circular cavity of radius r as shown in figure. On heating the system

View SolutionThe radius of gyration of a uniform circular ring of radius R, about an axis which is a chord of circle of length √3R is ,
View SolutionThe radius of gyration of a uniform circular ring of radius R, about an axis which is a chord of circle of length √3R is ,
View SolutionThe figure shows a disc of radius 3R from which a circular hole of radius R is cut as shown in the figure. The distance of the centre of mass of the remaining object from the point O is
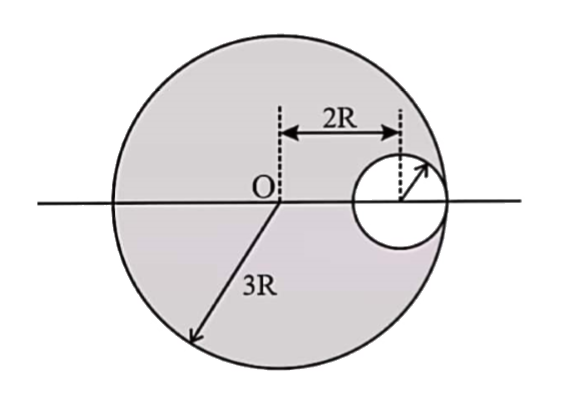
View SolutionThe radius of gyration of a solid shapere of radius r about a certain axis is r. The distance of this axis from the centre of the shpere is
View SolutionFrom the circular disc of radius 4R two small discs of radius R are cut off. The centre of mass of the new structure will be at

View SolutionFrom a uniform disc of radius R, a circular section of radius R/2 is cut out. The centre of the hole is at R/2 from the centre of the original disc. Locate the centre of mass of the resulting flat body.
View SolutionA disc of radius R has a concentric hole of radius R /2 and its mass is M. Its moment of inertia about an axis through its centre and perpendicular to its plane, is
View SolutionConvex and concave mirrors have the same radii of curvature R. The distanc between the
mirrors is 2R. At what point on the common optical axis of the mirrors should a point source of
light A be placed for the rays to coverage at the point A after being reflected first on the convex
and then on the concave mirror?
View SolutionA uniform circular disc has a radius of 20cm. A circular disc of radius 10cm is cut concentrically from the original disc. The shift in centre of mass is
View Solution
