The potential energy of a partical veries with distance x as shown in the graph.
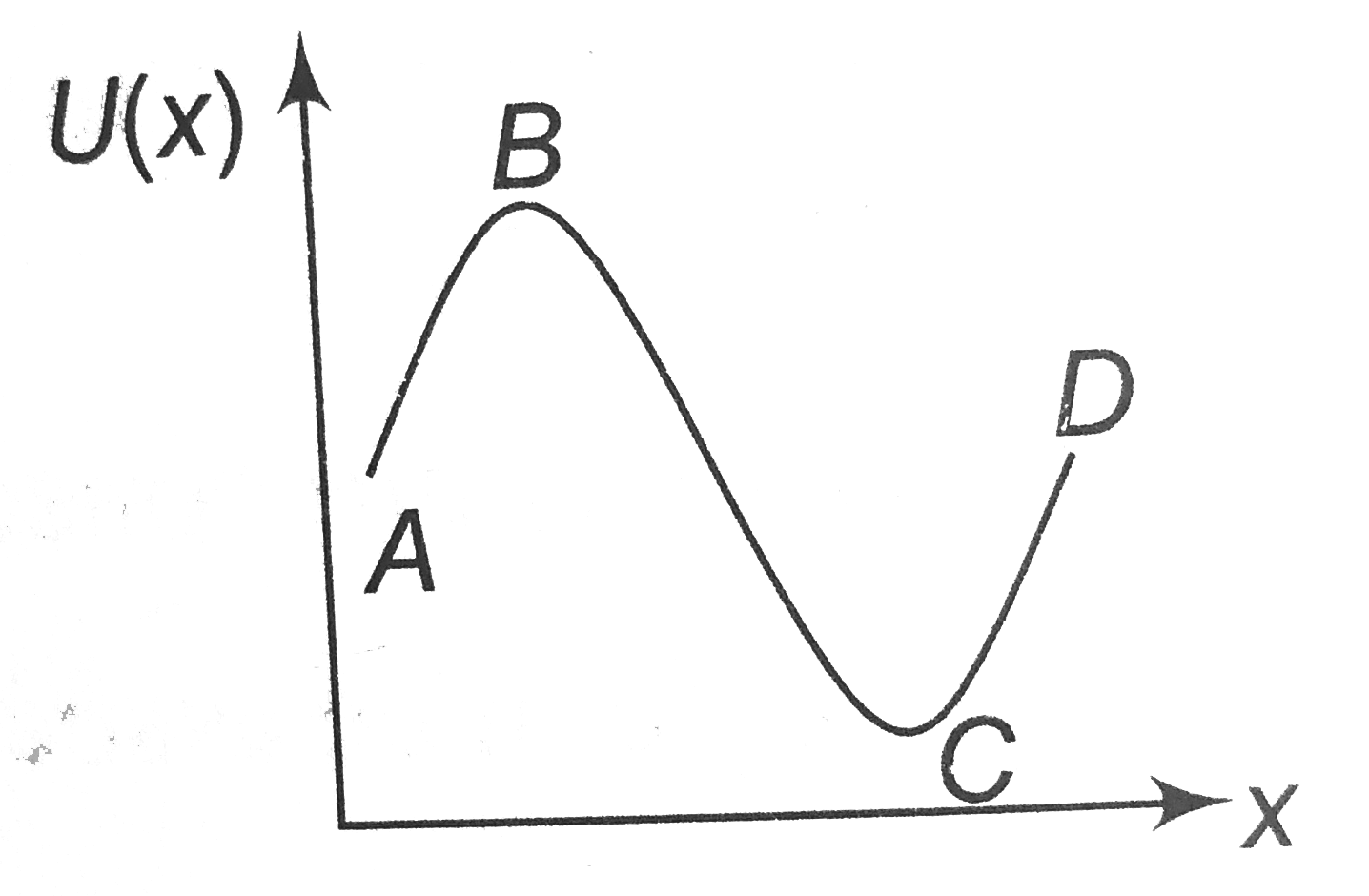
The force acting on the partical is zero at
C
B
B and C
A and D
The correct Answer is:C
F=−dUdx=0 at B and C
Topper's Solved these Questions
Similar Questions
force F on a partical moving in a straight line veries with distance d as shown in the figure. The work done on the partical during its displacement of 12m is
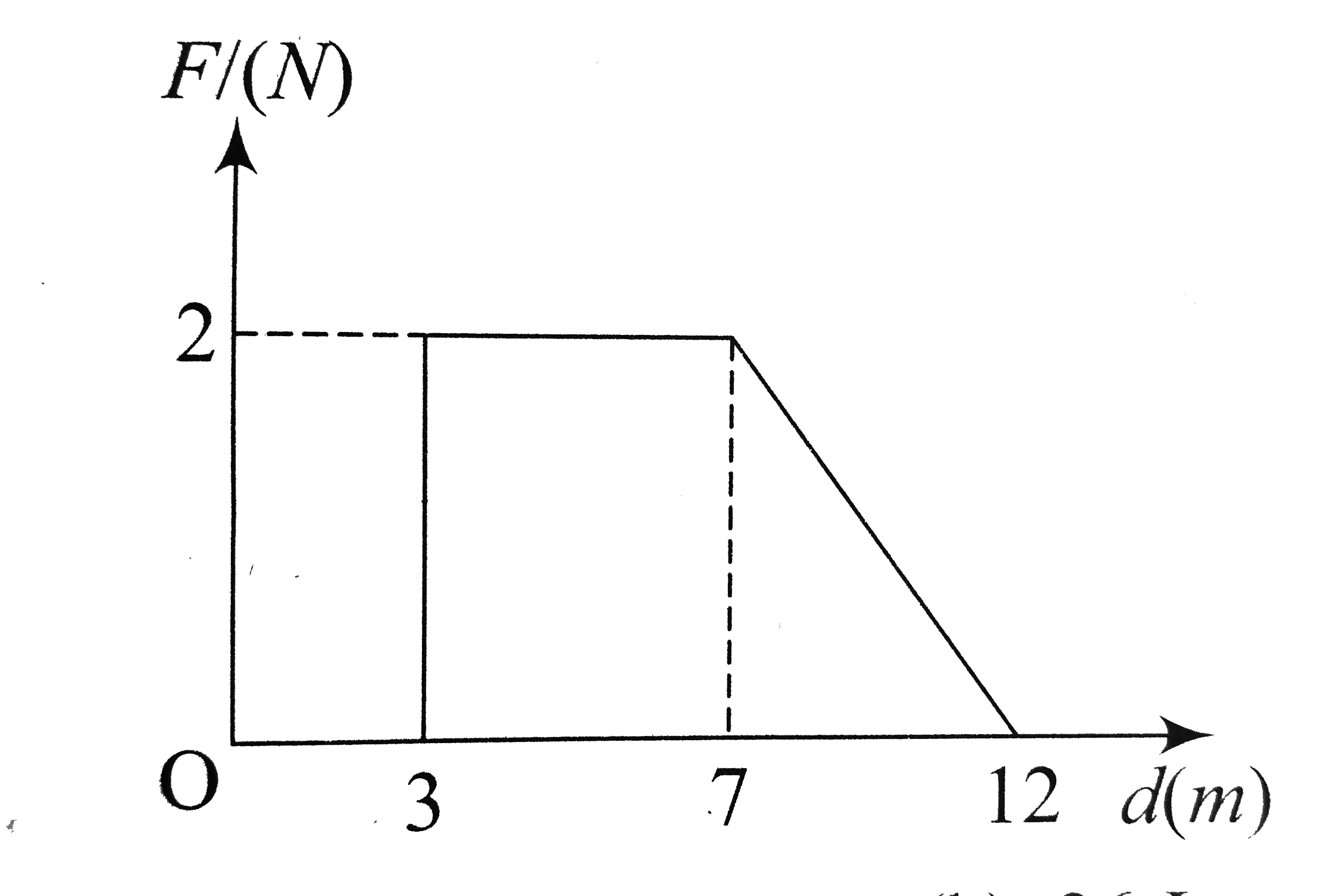
View SolutionThe displacement time graph of a particle executing S.H.M as shown in the figure.
 The corresponding force-time graph of the partical will be
The corresponding force-time graph of the partical will be
View Solution
Knowledge Check
The potential energy of a particle varies with distance x as shown in the graph. The force acting on the particle is zero at
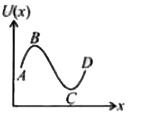 ACBBCB and CDA and D
ACBBCB and CDA and DThe potential energy of a particle varies with distance x as shown in the graph. The force acting on the particle is zero at
 ACBBCB and CDA and D.
ACBBCB and CDA and D.force F on a partical moving in a straight line veries with distance d as shown in the figure. The work done on the partical during its displacement of 12m is
 A21JB26JC13JD18J
A21JB26JC13JD18J
The potential energy of a body is given by U=A−Bx2 (where x is the displacement). The magnitude of force acting on the partical is
View SolutionThe potential energy of a partical is SHM is 2×10−4 J at the extreme positions. What will be its potential energy when the particle is midway between the mean and extreme position ?
View SolutionA particle of mass 0.2kg undergoes SHM according to the equation x(t)=3sin(πt+π/4).
i. What is the total energy of the particle if potential energy of zero at mean position ?
ii. What are the kinetic and potential energies of particle at time t=1s?
iii. At what time instants is the particles energies purely kinetic?
View SolutionThe potential energy of a body is given by U=A−Bx2 (where x is the displacement). The magnitude of force acting on the partical is
View SolutionAssertion: The change in kinetic energy of a partical is equal to the work done on it by the net force.
Reason: Change in kinetic energy of partical is equal to the work done in case of a system of one partical.
View Solution
