A heavy & big sphere is hang with a string of length l. This sphere move in a horizontal circular path making an angle θ with vertical then its time period is
T=2π√lg
T=2π√lsinθg
T=2π√lcosθg
T=2π√lgcosθ
The correct Answer is:C
T=2π√lcosθg
Topper's Solved these Questions
Similar Questions
A conical pendulum of length L makes an angle θ with the vertical. The time period will be

View SolutionIf the string of a conical pendulum makes an angle θ with horizontal, then square of its time period is proportional to
View SolutionA stone tied to a string of length l is whirled in a horizontal circle at a constant angular speed ω in a circle of radius r. If the string makes an angle θ with vertical then the tension in the string is :
View SolutionA pendulum consists of a small mass m at the end of a string of length L. The pendulum is pulled aside making an angle θ with the vertical and released. Taking the suspension point as the axis, at the instant of release
View SolutionA cart moves with a constant speed along a horizontal circular path. From the cart, a particle is thrown up vertically with respect to the cart.
View SolutionA charged particle moves in a circular path in a uniform magnetic field. If its speed is reduced, then its tiem period will
View SolutionA heavy particle hanging from a string of length I is projected horizontally with speed √gl . Find the speed of the particle at the point where the tension in the string equals weight of the particle.
View SolutionA particle of mass m is attached to the celling of a cabin with an inextensible light string of length l. The cabin is moving upward with an acceleration a . The particle is taken to a position such that string makes an angle θ with vertical. When string becomes vertical, find the tension in the string.
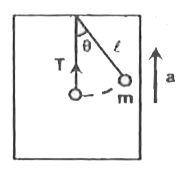
View SolutionTwo particles are projected with same initial velocity one makes angle θ with horizontal while other makes an angle θ with vertical. If their common range is R then product of their time of flight is directly proportional to :
View SolutionA sphere of mass 20 g is whirled around in a horizontal circular path on the end of a string, the other end of which is stationary. If the tension in the string is 12.62 N, calculate the length of the string.
View Solution
